A well of diameter 3 m is dug 14 m deep. The earth taken out of it has been spread evenly all around it in the shape of a circular ring of width 4 m to form an embankment. Find the height of the embankment.

Video Solution

Text Solution

Verified by Experts

Given the height of deep well which form a cylinder $(h_{1})$ =14m
and radius of deep well $(r_{1}) = \frac{3}{2}$
Let the width and height of the embankement form a circular ring are d and $h_{2}$
Here,
Now the voluem of deep well (cylinder)
$= π r_{1}^{2} h_{1} = \frac{22}{7} \times \frac{3}{2} \times \frac{3}{2} \times 14$
=99 $m^{3}$
Here, the embankemnt form a hollow cylinder
$∴$ The volume of water filled in a spherical galss vessel
= volume of cylinder+ volume of sphere
$= π r_{1}^{2} h_{1} + \frac{4}{3} π r_{2}^{3}$
Let the width and height of the embankment form a circular ring are d and $h_{2}$
Here,
Now the volume of deep well (cylinder)
$= π r_{1^{2}}^{2} h +_{1} = \frac{22}{7} \times \frac{3}{2} \times \frac{3}{2} \times \frac{3}{2} \times 14$
= $99 m^{3}$
Here the embankemnet form a hollow cylinder
$∴$ Volume of hollow cylinder (embankment) = $π {(r_{1} + d)}_{1}^{2} . h_{2} - π r_{1}^{2} h_{2}^{2}$
$= π \frac{3}{2 + 4^{2}} \times h_{2} 0 π (\frac{3}{2^{2}}) h_{2} = π [(\frac{11}{2^{2}}) h_{2} - \frac{9}{4} h_{2}]$
Now accordinng of question,
volume of deep well (cylinder)=volume of embankenet (hollow cylinder)
$99 = π [\frac{11}{{(2)}^{2}} \times h_{2} - \frac{9}{(4) h_{2}}]$
$= \frac{7 \times 99}{22} = \frac{121}{4} \times h_{2} - \frac{9}{4} h_{2} = \frac{112 h_{2}}{4}$
$h_{2} = \frac{7 \times 99 \times 4}{22 \times 112} = \frac{9}{8} = 11.25$

|

Updated on:21/07/2023

Class 10 MATHS SURFACE AREAS AND VOLUMES

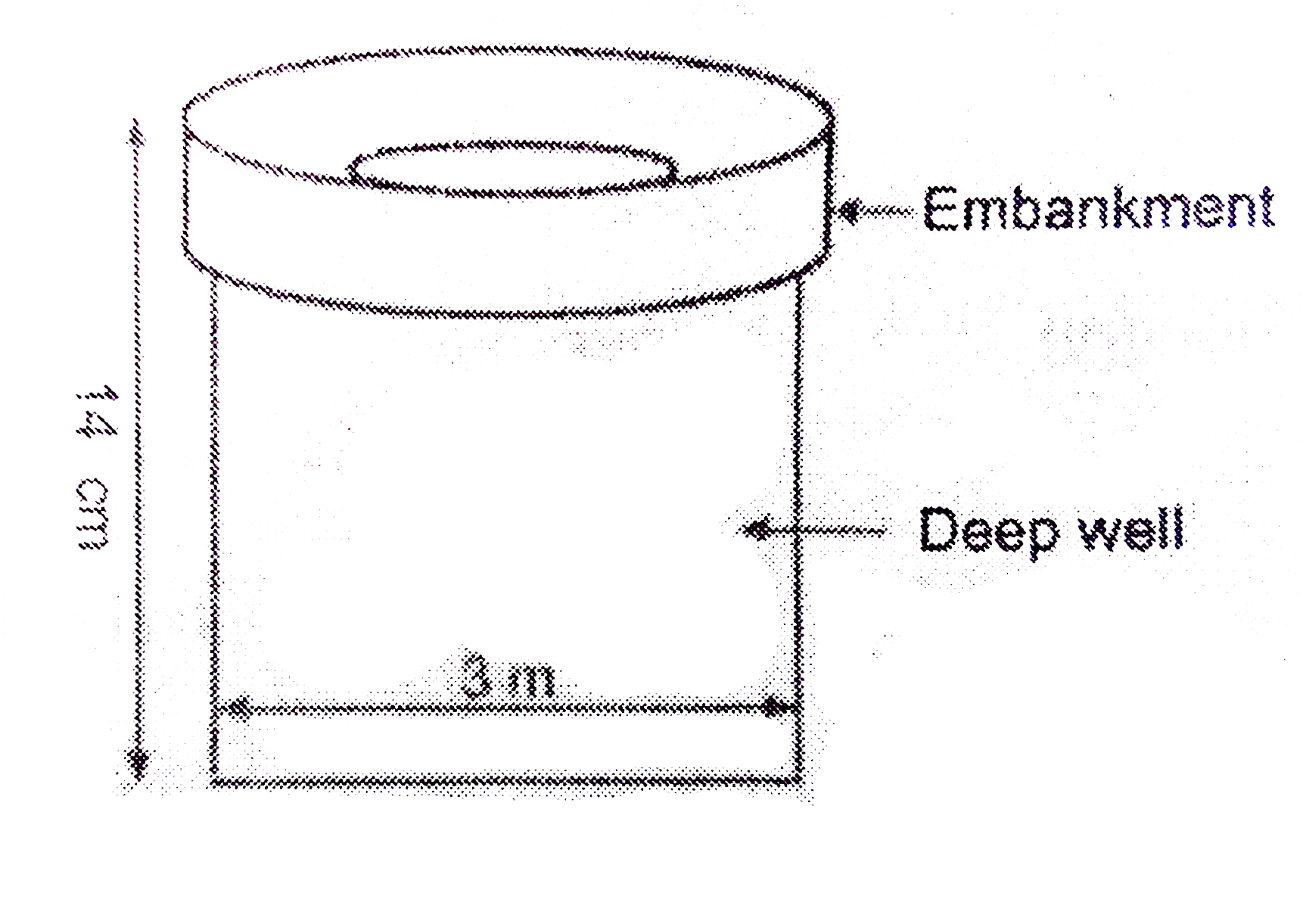
Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-VOLUME AND SURFACE AREA OF SOLIDS-Problems From NCERT/exemplar

Home
Ask Doubt
Profile