An open topped box is to be constructed by removing equal squares from each corner of a 3 metre by 8 metre rectangular sheet of aluminium and folding up the sides. Find the volume of the largest such box.
2003m3
2503m3
1003m3
3503m3
The correct Answer is:A
To find the volume of the largest open-topped box that can be constructed by removing equal squares from each corner of a 3m by 8m rectangular sheet of aluminum, we can follow these steps:
Step 1: Define the variables
Let x be the length of the side of the square cut from each corner. After cutting out the squares, the dimensions of the box will be:
- Length = 8−2x
- Width = 3−2x
- Height = x
Step 2: Write the volume formula
The volume V of the box can be expressed as:
V=Length×Width×Height=x(8−2x)(3−2x)
Step 3: Expand the volume expression
Now, we need to expand the expression for volume:
V=x(8−2x)(3−2x)
First, expand (8−2x)(3−2x):
(8−2x)(3−2x)=24−16x+6x−4x2=24−10x−4x2
Now, substituting back into the volume equation:
V=x(24−10x−4x2)=24x−10x2−4x3
Step 4: Differentiate the volume function
To find the maximum volume, we differentiate V with respect to x:
dVdx=24−20x−12x2
Step 5: Set the derivative to zero
To find critical points, set the derivative equal to zero:
24−20x−12x2=0
Rearranging gives:
12x2+20x−24=0
Dividing the entire equation by 4:
3x2+5x−6=0
Step 6: Factor the quadratic equation
Next, we factor the quadratic:
(3x−2)(x+3)=0
Thus, the solutions are:
x=23andx=−3
Since x must be positive, we take x=23.
Step 7: Determine if it is a maximum
To confirm that this critical point is a maximum, we can use the second derivative test:
d2Vdx2=−20−24x
Evaluating at x=23:
d2Vdx2=−20−24(23)=−20−16=−36
Since this is negative, x=23 is indeed a maximum.
Step 8: Calculate the maximum volume
Now, substitute x=23 back into the volume formula:
V=(23)(8−2⋅23)(3−2⋅23)
Calculating the dimensions:
=(23)(8−43)(3−43)
=(23)(243−43)(93−43)
=(23)(203)(53)
=2⋅20⋅527=20027 cubic meters
Final Answer
Thus, the maximum volume of the box is:
20027 cubic meters
Let x be the length of the side of the square cut from each corner. After cutting out the squares, the dimensions of the box will be:
- Length = 8−2x
- Width = 3−2x
- Height = x
The volume V of the box can be expressed as:
V=Length×Width×Height=x(8−2x)(3−2x)
Now, we need to expand the expression for volume:
V=x(8−2x)(3−2x)
First, expand (8−2x)(3−2x):
(8−2x)(3−2x)=24−16x+6x−4x2=24−10x−4x2
Now, substituting back into the volume equation:
V=x(24−10x−4x2)=24x−10x2−4x3
To find the maximum volume, we differentiate V with respect to x:
dVdx=24−20x−12x2
To find critical points, set the derivative equal to zero:
24−20x−12x2=0
Rearranging gives:
12x2+20x−24=0
Dividing the entire equation by 4:
3x2+5x−6=0
Next, we factor the quadratic:
(3x−2)(x+3)=0
Thus, the solutions are:
x=23andx=−3
Since x must be positive, we take x=23.
To confirm that this critical point is a maximum, we can use the second derivative test:
d2Vdx2=−20−24x
Evaluating at x=23:
d2Vdx2=−20−24(23)=−20−16=−36
Since this is negative, x=23 is indeed a maximum.
Now, substitute x=23 back into the volume formula:
V=(23)(8−2⋅23)(3−2⋅23)
Calculating the dimensions:
=(23)(8−43)(3−43)
=(23)(243−43)(93−43)
=(23)(203)(53)
=2⋅20⋅527=20027 cubic meters
Thus, the maximum volume of the box is:
20027 cubic meters
Topper's Solved these Questions
Similar Questions
A box of maximum volume with top open is to be made by cutting out four equal squares from four corners of a square tin sheet of side length a feet and then folding up the flaps. Find the side of the square cut-off.
View Solution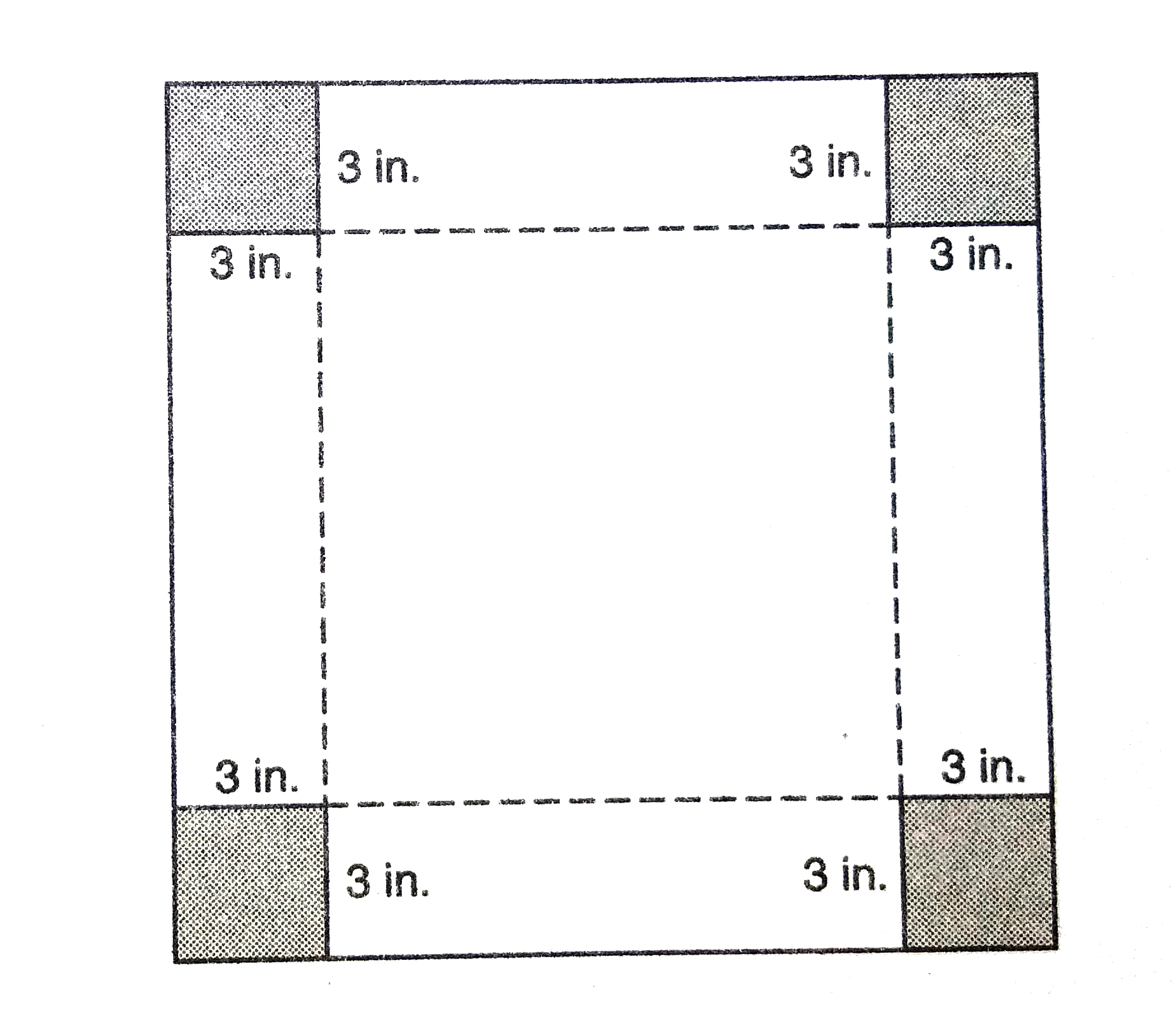
A box is constructed by cutting 3-inche square from the corner of a square sheet of cardboard, as shown in the accompanying diagram, and then folding the sides up. If the volume of the box is 75 inches, find the number of square inches in the area of the original sheet of cardboard?
View SolutionA square sheet of 2cm is cut from each corner of a rectangular piece of aluminium sheet of length 12cm and breadth 8cm. Find the area of the left over aluminium sheet.
View SolutionA rectangular sheet of tin 45 cm by 24 cm is to be made into a box without top by cutting off squares from the corners and folding up the flaps. What should be the side of the square in order the volume of the box is maximum.
View SolutionA rectangular sheet of tin 58 cm×44cm is to be made into an open box by cutting off equal squares from the corners and folding up the flaps.What should be the volume of box if the surface area of box is 2452 cm2?
View SolutionA square piece of tin of side 18 cm is to be made into a box without top by cutting a square from each corner and folding up the flaps to form a box. What should be the side of the square to be cut off so that the volume of the box is maximum? Also, find the maximum volume.
View SolutionA square piece of tin of side 18 cm is to be made into a box without top, by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?
View SolutionA rectangular sheet of fixed perimeter with sides having their lengths in the ratio 8:15 is converted into anopen rectangular box by folding after removing squares of equal area from all four corners. If the total area of removed squares is 100, the resulting box has maximum volume. Then the length of the sides of the rectangular sheet are (a) 24 (b) 32 (c) 45 (d) 60
View SolutionA rectangular sheet of fixed perimeter with sides having their lengths in the ratio 8:15 is converted into anopen rectangular box by folding after removing squares of equal area from all four corners. If the total area of removed squares is 100, the resulting box has maximum volume. Then the length of the sides of the rectangular sheet are 24 (b) 32 (c) 45 (d) 60
View SolutionThe volume of a cubical box is 32.768 cubic metres. Find the length of a side of the box.
View Solution
