Consider one sides AB of a square ABCD in order on line y=2x−17, and other two vertices C, D on y=x2
The maximum possible area of square ABCD is Solution in Bengali
1180
1250
1280
none
The correct Answer is:C
(3)
Let the equation of line CD be
y=2x+b (1)
and the length of side of square be a
Let the coordinates of C and D be (x1,y1)and(x2,y2), respectively.
Therefore,
y2−y1x2−x1=2 [∵CD∣∣AB]
Also, a2=(y2−y1)2+(x2−x1)2=5(x2−x1)2
ora2=5[x1+(x2)2−4x1x2] (2)
Solving (1) with y=x2, we get
x2=2x+b
orx1+x2=2,x1x2=−b
Putting in (2), we get
a2=20(b+1) (3)
Now, distance CD is

a=∣∣∣2x1−y1−17√5∣∣∣
=|−b−17|√5 [Using (1)]
or5a2=(b+17)2 (4)
From (3) and (4), we get
100(b+1)=(b+17)2
i.e., b=3or63
i.e., a2=80ora2=1280
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - MATRIX MATCH TYPE5videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - NUMERICAL VALUE TYPE32videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWER TYPE )26videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Numberical Value Type5videos
PAIR OF STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Comprehension8videos
PERMUTATION AND COMBINATION
Similar Questions
Consider the parabola (x−1)2+(y−2)2=(12x−5y+3)2169 and match the following lists :
 07:14View Solution
07:14View SolutionIf the length of the latus rectum rectum of the parabola 169{(x−1)2+(y−3)2}=(5x−12y+17)2 is L then the value of 13L4 is _________.
05:34View SolutionIf the focus of the parabola x2−ky+3=0 is (0,2), then a values of k is (are)
06:44View SolutionThe distance between the lines (x+7y)2+4√2(x+7y)−42=0 is_____________
05:03View SolutionGiven are six 0's, five 1's and four 2's . Consider all possible permutations of all these numbers. [A permutations can have its leading digit 0].
How many permutations have the first 0 preceding the first 1 ?
(a) 15C4×10C5 (b) 15C5×10C4 (c) 15C6×10C5 (d) 15C5×10C502:32View SolutionIf one side of the square is 2x−y+6=0, then one of the vertices is (2,1) . Find the other sides of the square.
02:43View SolutionOne side of square ABCD is on the line y=2x−17 and other two vertices are on parabola y=x2, if the minimum area of square is A then find A16.
02:53View SolutionConsider one sides AB of a square ABCD in order on line y=2x−17, and other two vertices C, D on y=x2
The minimum intercept of line CD on the y-axis is06:45View SolutionConsider one sides AB of a square ABCD in order on line y=2x−17, and other two vertices C, D on y=x2
The maximum possible area of square ABCD is06:18View SolutionABCD is a square of side 2a. Taking the centre of the square as origin and axes parallel to the sides AB and AD. The coordinates of the vertices of the square are
04:02View Solutionवर्ग ABCD का एक शीर्ष A मूलबिंदु है । यदि वर्ग की भुजाएँ AB और AD क्रमश : x-अक्ष और y-अक्ष हों और वर्ग की भुजा a हो , तो वर्ग के शीर्षों के निर्देशांक बताइए ।
06:37View SolutionABCD एक वर्ग है जिसकी भुजा a है । यदि AB और AD निर्देशाक्ष हों , तो सिद्ध कीजिए कि वर्ग के परिगत वृत्त का समीकरण x2+y2=a(x+y) है ।
05:04View SolutionThe area of a square ABCD is 36cm2. Find the area of the square obtained by joining the midpoints of the sides of the square ABCD.
08:26View SolutionABCD is a square with side 16 units and A is the origin. If the equation of the circle circumscribing the square ABCD is x2+y2=4k(x+y), then k=
08:11View SolutionABCD is a square , E is a point on AB such that BE=17 cm. The area of triangle ADE is 84 cm2 . What is the area of square ?
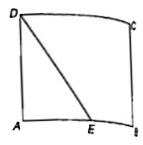 02:44View Solution
02:44View SolutionABCD একটি বর্গক্ষেত্র ও তার বাহুর দৈর্ঘ্য a, AB ও AD বাহুকে যথাক্রমে x ও y অক্ষ ধরে ABCD বর্গক্ষেত্রের পরিবৃত্তের সমীকরণ নির্ণয় করো|
14:56View Solution

