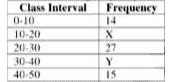
Consider the following frequency destribution :
Class interval0−1010−2020−3030−4040−50Frequency14x27y15
If the total of the frequencies is 100 and mode is 25, then which one of the following is correct?
x = 2y
2x = y
x = y
x = 3y
The correct Answer is:C
To solve the problem, we need to analyze the given frequency distribution and the conditions provided.
Given:
- Class intervals: 0-10, 10-20, 20-30, 30-40, 40-50
- Frequencies: 14, x, 27, y, 15
- Total frequency = 100
- Mode = 25
Step 1: Set up the equation for total frequency
The total frequency can be expressed as:
14+x+27+y+15=100
Step 2: Simplify the equation
Combine the known frequencies:
14+27+15=56
Thus, the equation becomes:
56+x+y=100
Step 3: Solve for x + y
Rearranging gives:
x+y=100−56
x+y=44
Step 4: Determine the modal class
Given that the mode is 25, we need to identify the modal class. The modal class is the class interval with the highest frequency. The frequency for the class intervals is:
- 0-10: 14
- 10-20: x
- 20-30: 27
- 30-40: y
- 40-50: 15
Since the mode is 25, the modal class must be 20-30 (as it contains the value 25).
Step 5: Analyze the frequencies
For the modal class (20-30) to be the mode, it must have the highest frequency compared to the other class intervals. Therefore:
27>x(since 27 is the frequency for 20-30)
27>y(since y is the frequency for 30-40)
Step 6: Check the options
We need to check the relationships between x and y based on the equation x+y=44 and the conditions x<27 and y<27.
1. Option A: x=2y
- Substitute: 2y+y=44 → 3y=44 → y=443 (not an integer)
2. Option B: x=y
- Substitute: y+y=44 → 2y=44 → y=22, x=22 (valid)
3. Option C: x=3y
- Substitute: 3y+y=44 → 4y=44 → y=11, x=33 (not valid as x exceeds 27)
Conclusion
The only valid option is:
- Option B: x=y, where both x and y equal 22.
Final Answer
Thus, the correct relationship is x=y.
- Class intervals: 0-10, 10-20, 20-30, 30-40, 40-50
- Frequencies: 14, x, 27, y, 15
- Total frequency = 100
- Mode = 25
The total frequency can be expressed as:
14+x+27+y+15=100
Combine the known frequencies:
14+27+15=56
Thus, the equation becomes:
56+x+y=100
Rearranging gives:
x+y=100−56
x+y=44
Given that the mode is 25, we need to identify the modal class. The modal class is the class interval with the highest frequency. The frequency for the class intervals is:
- 0-10: 14
- 10-20: x
- 20-30: 27
- 30-40: y
- 40-50: 15
For the modal class (20-30) to be the mode, it must have the highest frequency compared to the other class intervals. Therefore:
27>x(since 27 is the frequency for 20-30)
27>y(since y is the frequency for 30-40)
We need to check the relationships between x and y based on the equation x+y=44 and the conditions x<27 and y<27.
- Substitute: 2y+y=44 → 3y=44 → y=443 (not an integer)
2. Option B: x=y
- Substitute: y+y=44 → 2y=44 → y=22, x=22 (valid)
3. Option C: x=3y
- Substitute: 3y+y=44 → 4y=44 → y=11, x=33 (not valid as x exceeds 27)
The only valid option is:
- Option B: x=y, where both x and y equal 22.
Thus, the correct relationship is x=y.
Topper's Solved these Questions
- BOOK - NDA PREVIOUS YEARSCHAPTER - SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM EXERCISE - MCQ271videos
SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM
- BOOK - NDA PREVIOUS YEARSCHAPTER - TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSEXERCISE - MCQ238videos
TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The median class in the following frequency distribution is
Class interval :0−1010−2020−3030−4040−50Frequency :1213252010A10−20B20−30C30−40Dnone of these - Question 2 - Select One
Class Interval0.1010−2020−3030−4040−50Frequency5020510
What is the mean of the above frequency distribution?A25B26C27D28 - Question 3 - Select One
Class Interval0.1010−2020−3030−4040−50Frequency5020510
What is the median of the above frequency distribution?A23B24C25D26
Calculate mode from the following data :
Class Interval0−1010−2020−3030−4040−50Frequency 2 5 7 5 2View SolutionConstruct a histogram for the frequency distribution below:
Class Interval10−2020−3030−4040−5050−60Frequency63847View SolutionFind the mean of the following frequency distribution:
Class0−55−1010−1515−2020−2525−30Frequency122672View SolutionFind the median of the following data:
Class Interval0−1010−2020−3030−4040−50f68547View SolutionThe mode from given grouped data would be
Class Interval10−2020−3030−4040−5050−60Frequency57684View Solution