Corner points of feasible region for an LPP are (0,2),(3,0),(6,0),(6,8) and (0,5). Let F=4 x+6 y be the objective function. The minimum value of F occur atSolution in Kannada
(0,2) only
(3,0) only
the mid point of the line segment joining the points (0,2) and (3,0) only
any point on the line segment joining the points (0,2) and (3,0)
The correct Answer is:D
Step by step video, text & image solution for Corner points of feasible region for an LPP are (0,2),(3,0),(6,0),(6,8) and (0,5). Let F=4 x+6 y be the objective function. The minimum value of F occur at by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
The inequality 4x<5 is true, when x belongs to
01:39View Solution1+3+5+....+(2n−1)=
01:14View SolutionCorner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8), and (0,5). Let F=4x+6y be the objective function. Determine the minimum value of F occurs at
01:21View SolutionMaximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
05:57View SolutionConsider the following statements
I. If the feasible region of an LPP is undbounded then maximum or minimum value of the obJective function Z = ax + by may or may not exist .
II. Maximum value of the objective function Z = ax + by in an LPP always occurs at only one corner point of the feasible region.
Ill. In an LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
IV. In an LPP, the maximum value of the objective function Z = ax + by is always finite.
Which of the following statements are true?03:14View Solutionकिसी LPP के सुसंगत क्षेत्र के कोणीय बिन्दु (0,2), (3,0), (6,0),(6,8) तथा (0,5) है | मान लीजिए कि F=4x+6y उद्देश्य फलन है | F का न्यूनतम मान किस बिन्दु पर है ?
02:44View Solutionकिसी LPP के सुसंगत क्षेत्र के कोणीय बिन्दु (0,2), (3,0), (6,0),(6,8) तथा (0,5) है | मान लीजिए कि F=4x+6y उद्देश्य फलन है | F का अधिकतम मान −F का न्यूनतम मान बराबर है :
02:22View SolutionCorner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8), and (0,5). Let F-4x+6y be the objective function. Determine the minimum valur of F occurs at
02:06View SolutionMaximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
02:08View SolutionCorner points of the feasible region for an LPP are (0, 2), (3, 0), (6, 0), (6, 8) and (0, 5).
Let F = 4x + 6y be the objective function. (Maximum of F)-(Minimum of F) =02:20View SolutionMaximum value of the objective function Z = ax + by in a LPP always occurs at only one corner point of the feasible region.
View SolutionIn a LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
03:52View SolutionIf the corner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8),(0,5) then maximum of F - minimum of F =
02:58View SolutionThe feasible region for an LPP is shown in the following figure. Let F=3 x-4 y be the objective function. Maximum value of F is
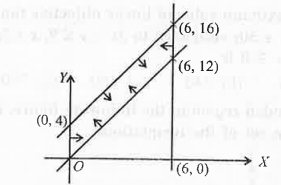 01:06View Solution
01:06View SolutionThe feasible region for an LPP is shown in the following figure. Let F=3 x-4 y be the objective function. Minimum value of F is
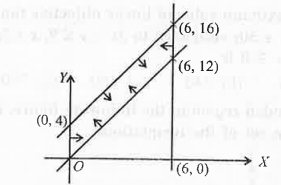 01:36View Solution
01:36View SolutionThe feasible region for an LPP is shown in the figure. Let F = 3 x - 4 y be the objective function. Maximum value of F is:
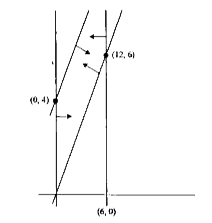 01:17View Solution
01:17View Solution

