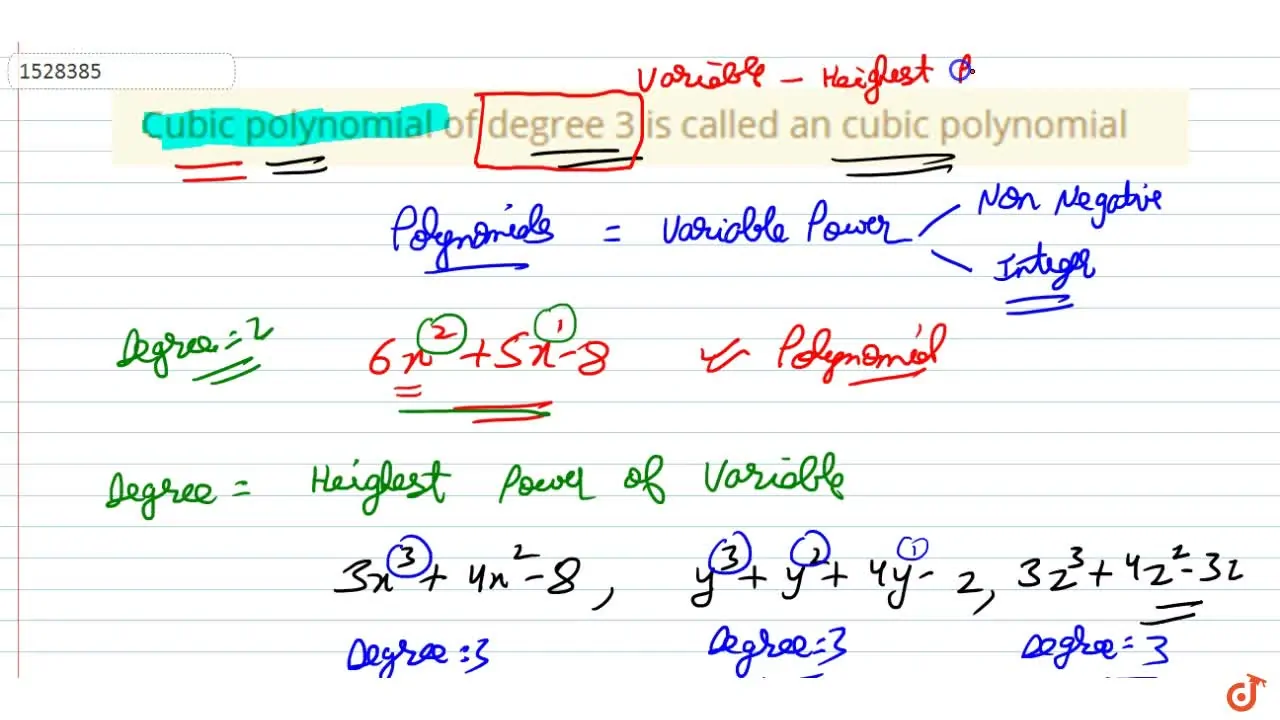
Cubic polynomial of degree 3 is called an cubic polynomial
Step by step video & image solution for Cubic polynomial of degree 3 is called an cubic polynomial by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Similar Questions
Answer the following questions below :
A polynomial of degree ………………… is called a cubic polynomial .View SolutionQuadratic polynomial a polynomial of degree 2 is called a quadratic polynomial
View SolutionBiquadratic polynomial a polynomial of degree 4 is called a biquadratic polynomial.
View SolutionLinear polynomial a polynomial of degree 1 is called a linear polynomial.
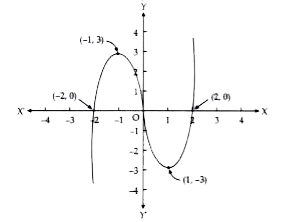
View SolutionGraph of cubic polynomial
View SolutionA polynomial of degree 7 is divided by a polynomial of degree 4. Degree of the quotient is
View SolutionGraph of the polynomial of degree 3 is shown. Find the polynomial represented by the graph. Where coordinate of R is (52,0)
View Solution√3 is a polynomial of degree
View Solution√2 is a polynomial of degree
View SolutionTypes of Polynomial on the basis of degree of Polynomial (iv) Cubic polynomial (v) Bi-quadratic polynomial
View Solution