Find the angle between the two planes 2x+y2z=5and 3x6y2z=7using vector method.
Video Solution
Text Solution
Direction ratios of the normal of plane 1 2x+y−2z=5 are (2,1,−2).
Direction ratios of the normal of plane 2 3x−6y−2z=7 are (3,−6,−2).
∴ Direction cosines of plane 1 = ⎛⎜
⎜⎝2√22+12+(−2)2,1√22+12+(−2)2,−2√22+12+(−2)2⎞⎟
⎟⎠
=(23,13,−23).
Direction cosines of plane 2 = ⎛⎜
⎜⎝3√32+(−6)2+(−2)2,−6√32+(−6)2+(−2)2,−2√32+(−6)2+(−2)2
=(37,−67,−27).
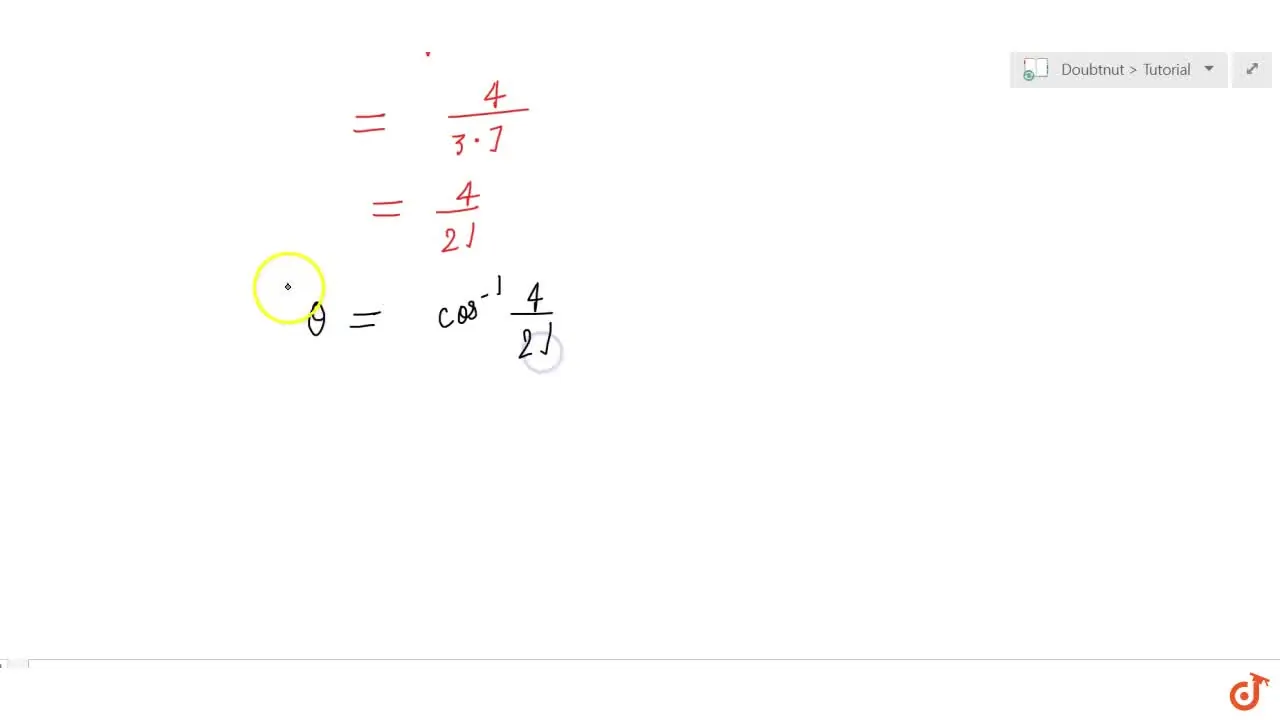
∴cosθ=l1l2+m1m2+n1n2=(23⋅37)+(13⋅−67)+(−23⋅−27)
⇒cosθ=421
∴θ=cos−1(421).
So, the angle between these two planes is cos−1(421).
|
Updated on:21/07/2023
Similar Questions
Find the angle between the planes 2x+y−2z=5and3x−6y−2z=7. Using vector method.
View SolutionFind the angle between the planes 2x−y+3z=6andx+y+2z=7.
View SolutionFind the angle between the two planes 3x−6y+2z=7and 2x+2y−2z=5.
View SolutionThe acute angle between the two planes x+y+2z
= 3 and 3x - 2y + 2z = 7 is …….View SolutionFind the angle between the plane:2x−y+z=6andx+y+2z=7
View SolutionFind the angle between the planes −x+y+2z=9andx+2y+z=5
View SolutionFind the angle between the planes x+y+2z=9adn2x−y+z=15.
View SolutionFind the angle between the plane:2x+y−2z=5and3x−6y−2z=7
View SolutionFind the angle between the planes x−y+2z=9and2x+y+z=7.
View SolutionFind the angle between the planes 2x−3y+6z=9 and xy plane
View Solution