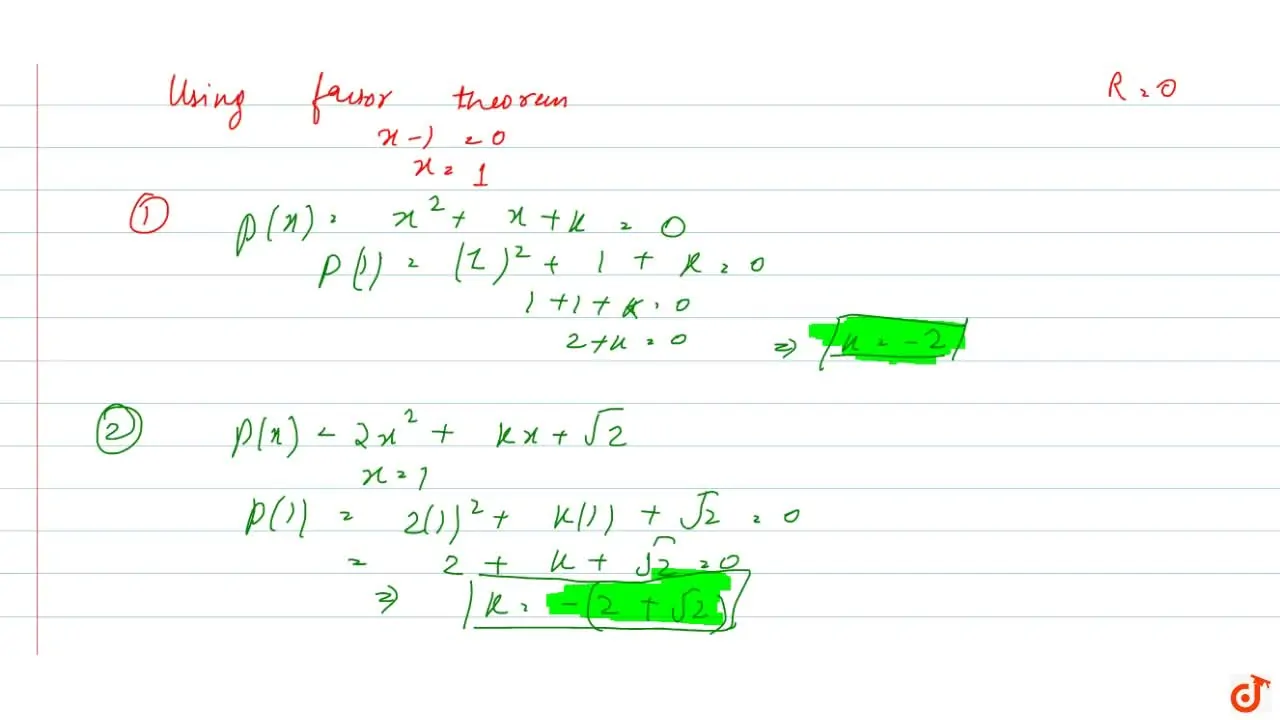
Find the value of k, if x−1is a factor of p(x) in each of the following cases:
(i) p(x)=x2+x+k (ii) p(x)=2x2+kx+√2
(iii) p(x)=kx2−√2x+1(iv) p(x)=kx2−3x+k
Here, (x−1) is a factor of p(x) that means from Factor theorem,
p(1)=0.
(i)p(x)=x2+x+k
As, p(1) is 0
0=12+1+k⇒k=−2
(ii)p(x)=2x2+kx+√2
As, p(1) is 0
2+k+√2=0⇒k=−(2+√2)
(iii)p(x)=kx2−√2x+1
As, p(1) is 0
k−√2+1=0⇒k=√2−1
(iv)p(x)=kx2−3x+k
As, p(1) is 0
k−3+k=0⇒2k=3
k=32
Related Playlists
- BOOK - NCERTCHAPTER - POLYNOMIALSEXERCISE - Exercise 2.33videos
POLYNOMIALS
- BOOK - NCERTCHAPTER - POLYNOMIALSEXERCISE - Exercise 2.24videos
POLYNOMIALS
- BOOK - NCERTCHAPTER - NUMBER SYSTEMSEXERCISE - EXERCISE 1.42videos
NUMBER SYSTEMS
- BOOK - NCERTCHAPTER - PROBABILITYEXERCISE - SOLVED EXAMPLES10videos
PROBABILITY
Similar Questions
Find the value of k , if x−1 is a factor of p(x) in each of the following cases :
(i) p(x)=x2+x+k
(ii) p(x)=2x2+kx+√2
(iii) p(x)=kx2−√2x+1
(iv) p(x)=kx2−3x+kView SolutionFind the value of k, if x-1 is a factor of p(x) in each of the following cases :
(i) p(x)=x2+x+k (ii)p(x)=2x2+kx+√2
(iii) p(x)=kx2−√2x+1 (iv)p(x)=kx2−3x+kView SolutionFind the value of k if (x-1) is factor of x3+3x2−4x+k.
View SolutionFind the value of k if x+1 is a factor of p(x): p(x)=kx2+3x+k
View SolutionFind the value of k, if (x-1) is a factor of 4x3+3x2−4x+k.
View SolutionFind the value of k, if x−1is a factor of 4x3+3x2−4x+k.
View SolutionFind the value of k, if (x−3) is a factor of k3x3−x2+3x−1.
View SolutionUse the Factor Theorem to determine whether g(x) is a factor of p(x) in each of the following cases:(i) p(x)=2x3+x2−2x−1,g(x)=x+1
(ii) p(x)=x3+3x2+3x+1,g(x)=x+2
(iii) p(x)=x3+4x2+x+6,g(x)=x−3View SolutionUse the factor theorem, to determine whether g(x) is a factor of p(x) in each of the following cases :
(i) p(x)=2x3+x2−2x−1,g(x)=x+1
(ii) p(x)=x3+3x2+3x+1,g(x)=x+2
(iii) p(x)=x3−4x2+x+6,g(x)=x−3View SolutionFind p(0), p(1) and p(2) for each of the following polynomials :
(i) p(y)=y2−y+1
(ii) p(t)=2+t+2t2−t3
(iii) p(x)=x3
(iv) p(x)=(x−1)(x+1)View Solution