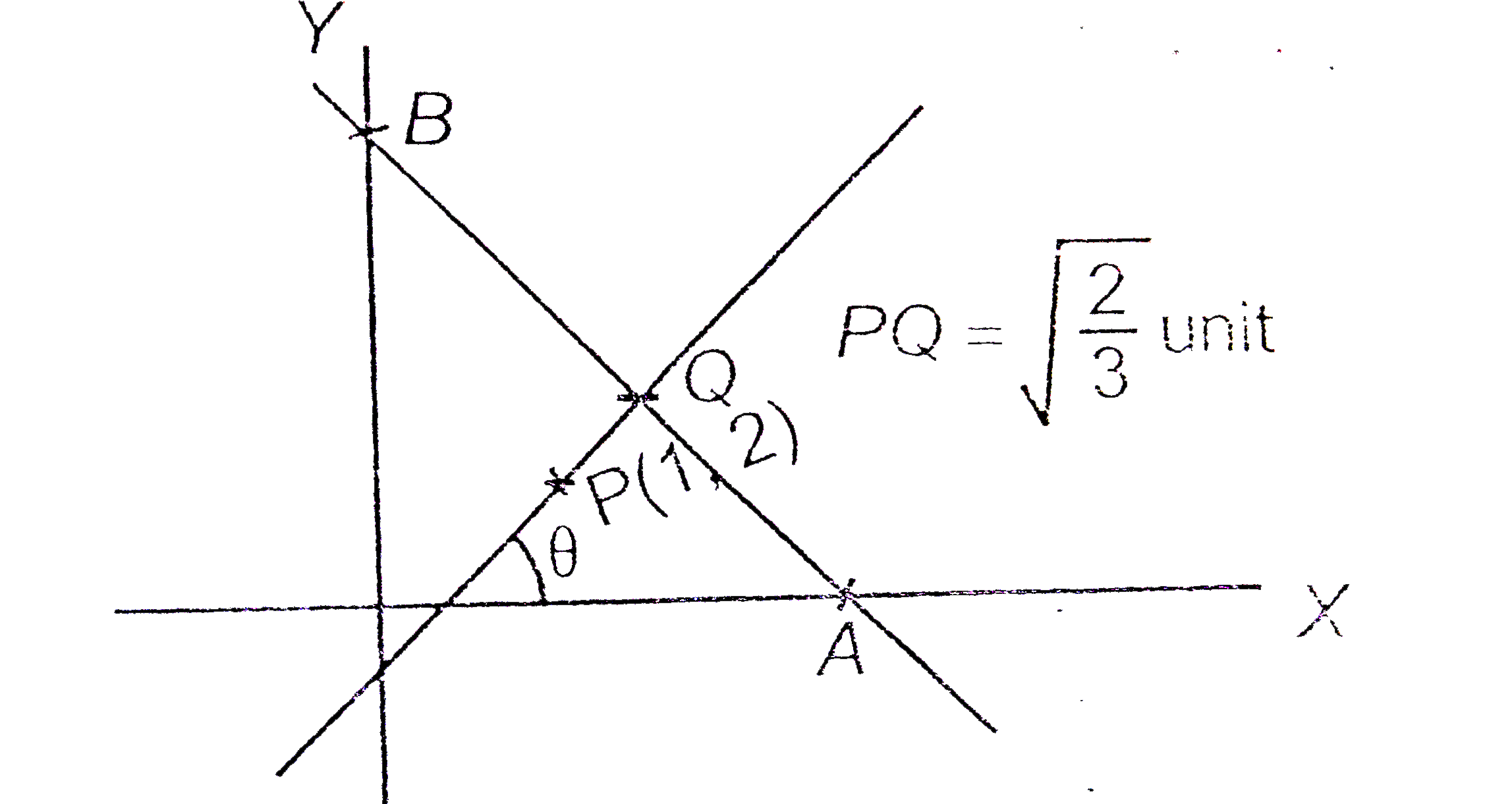
(i) Find the direction in which a straight line must be drawn torough the points (1,2) so that its point of intersection with the line x+y=4 may be the a distance √23 from this point.
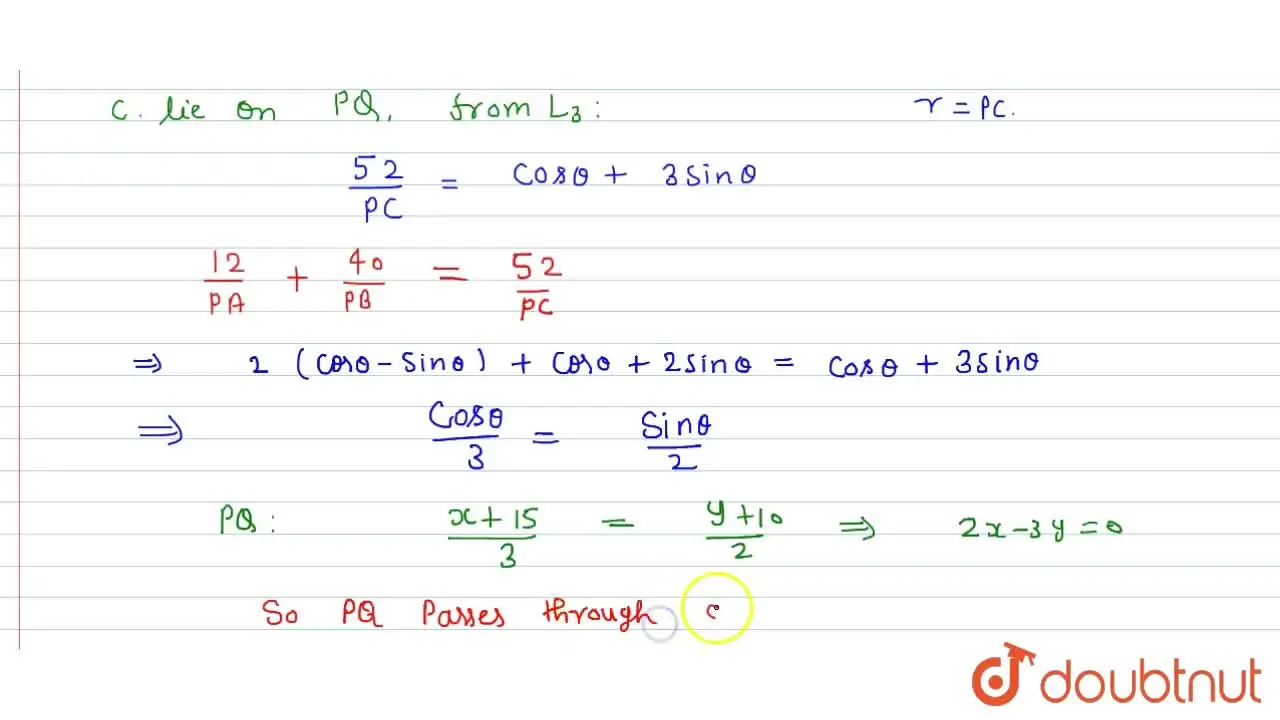
(ii) A straight line through P(−15,−10) meets the straight lies x−y−1=0,x+2y=5andx+3y=7 respectively at A,B and C, if 12PA+40PB=52PC, then prove that the straight line passes through origin.
(i) Let the straight line through P(1,2) cut the given straight line x+y=4 at Q and the straight line is inclined at an angle θ with positive direction of x-axis. Then its equation is x−1cosθ=y−2sinθ=r,PQ=r.
The coordinates of the point Q are (1+rcosθ,2+rsinθ)
Which lies on x+y=4
⇒√23(cosθ+sinθ)=1,r=√23, given
⇒cosθ+sinθ=√32
⇒1√2cosθ+1√2sinθ=1√2√32=√32
⇒cos(θ−π4)=cosπ6
⇒θ−π4=π6or−π6
⇒θ=π4+π6orπ4−π6
⇒θ=5π12or=π12
(ii) Let the equation of straight line passing throug P(−15,−10) be x+15cosθ=y+10sinθ
which cuts the given straight lines
x−y−1=0,x+2y=5andx+3y=7 at A, B

and C respectively.
Any point on this line may be takes as
(−15+rcosθ,−10+rsinθ)
For the coordinates of the point A,(−15rcosθ,−10+rsinθ) lies on x−y−1=0
⇒−15+rcosθ+10−rsinθ−1=0
⇒r(cosθ−sinθ)=6
⇒6PA=cosθ−sinθ
Also for B,l15+rcosθ−20+2rsinθ=5
⇒r(cosθ+2sinθ)=40
⇒40PB=cosθ2sinθ
and for the point C,15+rcosθ−30+3rsinθ=7
⇒r(cosθ+2sinθ)=52
⇒cosθ+3sinθ=52PC
Now from the given condition,
12PA+40PB=52PC
⇒2(cosθ−sinθ)+cosθ+2sinθ=cosθ+3sinθ
⇒2cosθ=3sinθ⇒cosθ3=sinθ2
Hence the required equation of the straight line is
x+153=y+102
⇒2x+30=3y+30
⇒2x−3y=0
which passes through origin.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - STRAIGHT LINESEXERCISE - TRY YOURSELF23videos
STRAIGHT LINES
- BOOK - AAKASH INSTITUTECHAPTER - STRAIGHT LINESEXERCISE - ASSIGNMENT (SECTION A) (OBJECTIVE TYPE QUESTIONS) (ONLY ONE ANSWER)50videos
STRAIGHT LINES
- BOOK - AAKASH INSTITUTECHAPTER - STATISTICSEXERCISE - Section-C Assertion-Reason15videos
STATISTICS
- BOOK - AAKASH INSTITUTECHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - ASSIGNMENT SECTION - J10videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
Find the direction in which a straight line must be drawn through the point (1,2) so that its point of intersection with the line x+y=4 may be at a distance 13√6 from this point
View SolutionFind the direction in which a straight line must be drawn through the point (−1,2) so that its point of intersection with the line x+y=4 may be at a distance of 3 units from this point.
View SolutionThe direction in which a straight line must be drawn through the point (1,2) so that its point of intersection with the x+y=4 may be at a distance √63 from theis point is
View SolutionFind the direction in which a straight line must be drawn through the point (−1,2)so that its point of intersection with the line x+y=4 may be at a distance of 3 units from this point.
View SolutionA straight line through A(−15−10) meets the lines x−y−1=0, x+2y=5 and x+3y=7 respectively at A, B and C. If 12AB+40AC=52AD prove that the line passes through the origin.
View SolutionFind the equation of the straight line whichpasses through the point (1,1) and the point of intersection of the lines 3x+2y=0andx−2y=0
View SolutionFind the equation of the straight line passing through the point (2,1) and through the point of intersection of the lines x+2y=3and2x−3y=4
View SolutionFind the equation to the straight line passing through (i) The point (3,2) and the point of intersection of the lines 2x+3y=1 and 3x−4y=6
View SolutionThe straight line 2x+3y=12 passes through :
View SolutionFind the equation of the straight line passing through the point (2,−6) and the point of intersection of the lines 5x−2y+14=0and2y=8−7x.
View Solution