Find the number of arrangements of the letters of the INDEPENDENCE. In how many of these arrangements, (i) do the words start with P (ii) do all the vowels always occur together (iii) do the vowels never occur together (iv) do the words begin wit
I. The given word contains 12 letters out of which N occurs 3 times, E occurs 4 times, D occurs 2 times and the rest are all different.
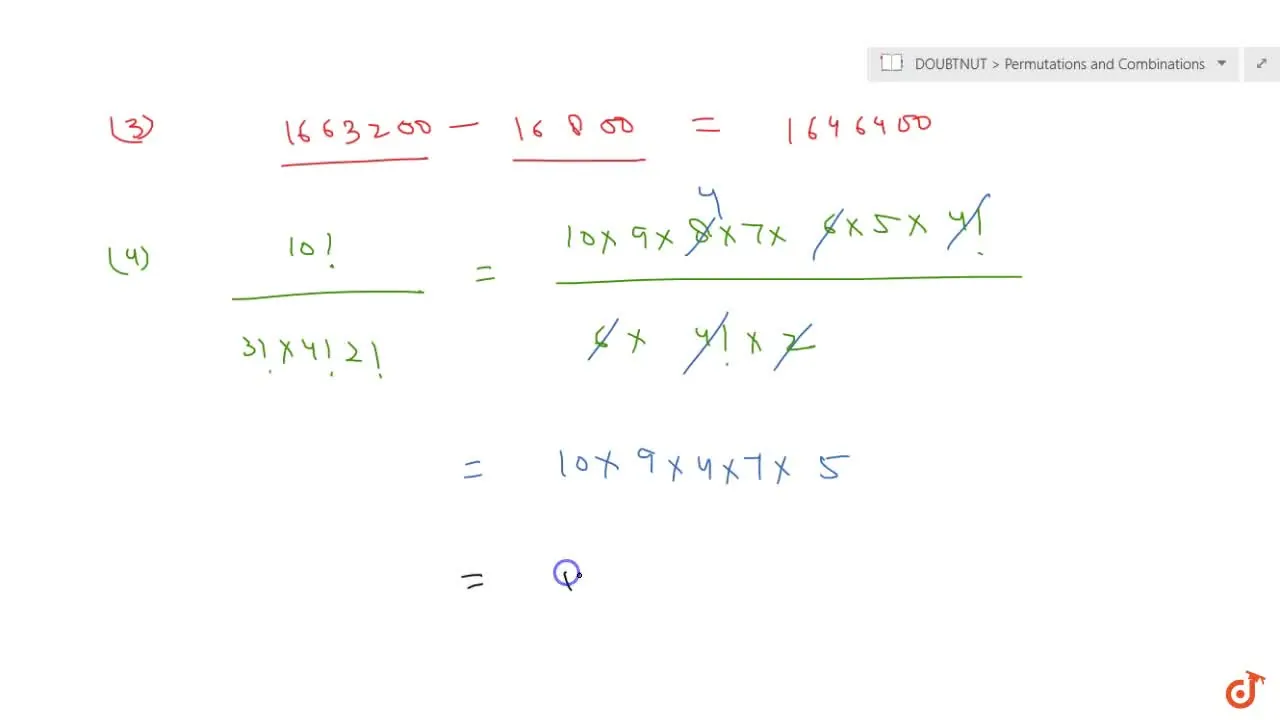
Hence, the required number of arrangements
=12!(3!)×(4!)×(2!)=1663200.
II. (i) After fixing P at the extreme left position, there are 11 letters consisting of 3 N's, 4E's, 2D's, 11 and 1 C.
So, the number of words beginning with P
= number of arrangements of 11 letters out of which there are 3N's, 4E's, 2D's, 11 and 1C
=11!(3!)×(4!)×(2!)=138600.
(ii) There are 5 vowels in the given word, namely 4E's and 11.
Let us treat them as a single letter EEEEI.
This letter with 7 remaining letters will give us 8 letters in which there are 3 N's, 2 D's, 1 P, 1 C and 1 letter EEEEI.
Number of all such arrangements =8!(3!)×(2!)=3360.
Number of arrangements of 5 vowels, namely 4E' s and 11
=5!4!=5.
Hence, the number of words in which vowels are together
=(3360×5)=16800.
(iii) Number of arrangements in which vowels do not occur together
=(→talνmberofarran≥ments)−(νmberofarran≥ments∈whichvowelsour→≥ther)<b>=(1663200-16800) =1646400.<br>(iv)LetusfixIatthe≤ftendandPattherightendofeacharran≥ment.<br>Then,weare≤ftwith10≤ers,outofwhichthereare3N's,4E's,2D'sand1C.<br>Hence,theνmberofwordswhichbeg∈withIandend∈P=(10!)/((3!)xx(4!)xx(2!))=12600.
Related Playlists
- BOOK - RS AGGARWALCHAPTER - PERMUTATIONSEXERCISE - EXERCISE 8A14videos
PERMUTATIONS
- BOOK - RS AGGARWALCHAPTER - PERMUTATIONSEXERCISE - EXERCISE 8B36videos
PERMUTATIONS
- BOOK - RS AGGARWALCHAPTER - PERMUTATIONSEXERCISE - ILLUSTRATIVE EXAMPLES25videos
PERMUTATIONS
- BOOK - RS AGGARWALCHAPTER - PARABOLAEXERCISE - EXERCISE-2210videos
PARABOLA
- BOOK - RS AGGARWALCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - EXERCISE 423videos
PRINCIPLE OF MATHEMATICAL INDUCTION
Similar Questions
Find the numbers of arrangements of the letters of the word INDEPENDENCE. In how many of these arrangements. Do the words start with P? Do all the vowels never occur together? Do all the vowels always occur together? Do the words begin with I and end in P?
View SolutionFind the number of arrangements of the letters of the word MADHUBANI . In how many of these arrangements (i) Do the words starts with B ? (ii) Do the vowels occur together? (iii) Do you words begin with D and end in H ?
View SolutionFind the number of arrangements of the letters of the word SALOON, if the two O’s do not come together.
View SolutionHow many arrangements of the letters of the word 'BENGALI' can be made if the vowels are never together.
View SolutionNumber of arrangements of the letters of the word ENGINEER so that all vowels are together but all Es are not together is
View SolutionFind the number of words formed by permuting all letters of the word INDIA. In how many of them vowels are together ?
View SolutionFind the number of arrangements of the letters of the word PARALLEL so that all Ls do not come together but all As come together.
View SolutionIn how many arrangements of the word 'GOLDEN' will the vowels never occur together ?
View SolutionIn how many ways can the letters of the word 'PERMUTATIONS' be arranged, if
(i) the words start with P and end with S?
(ii) the vowels are all together?View SolutionHow many words can be formed form the letters of the word DAUGHTER so that The vowels always come together? The vowels never come together?
View Solution