If $(- 2, 5)$ and (3,7) are the points of intersection of the tangent and normal at a point on a parabola with the axis of the parabola, then the focal distance of that point is

A

$\frac{\sqrt{29}}{2}$

B

$\frac{5}{2}$

C

$\sqrt{29}$

D

$\frac{2}{5}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

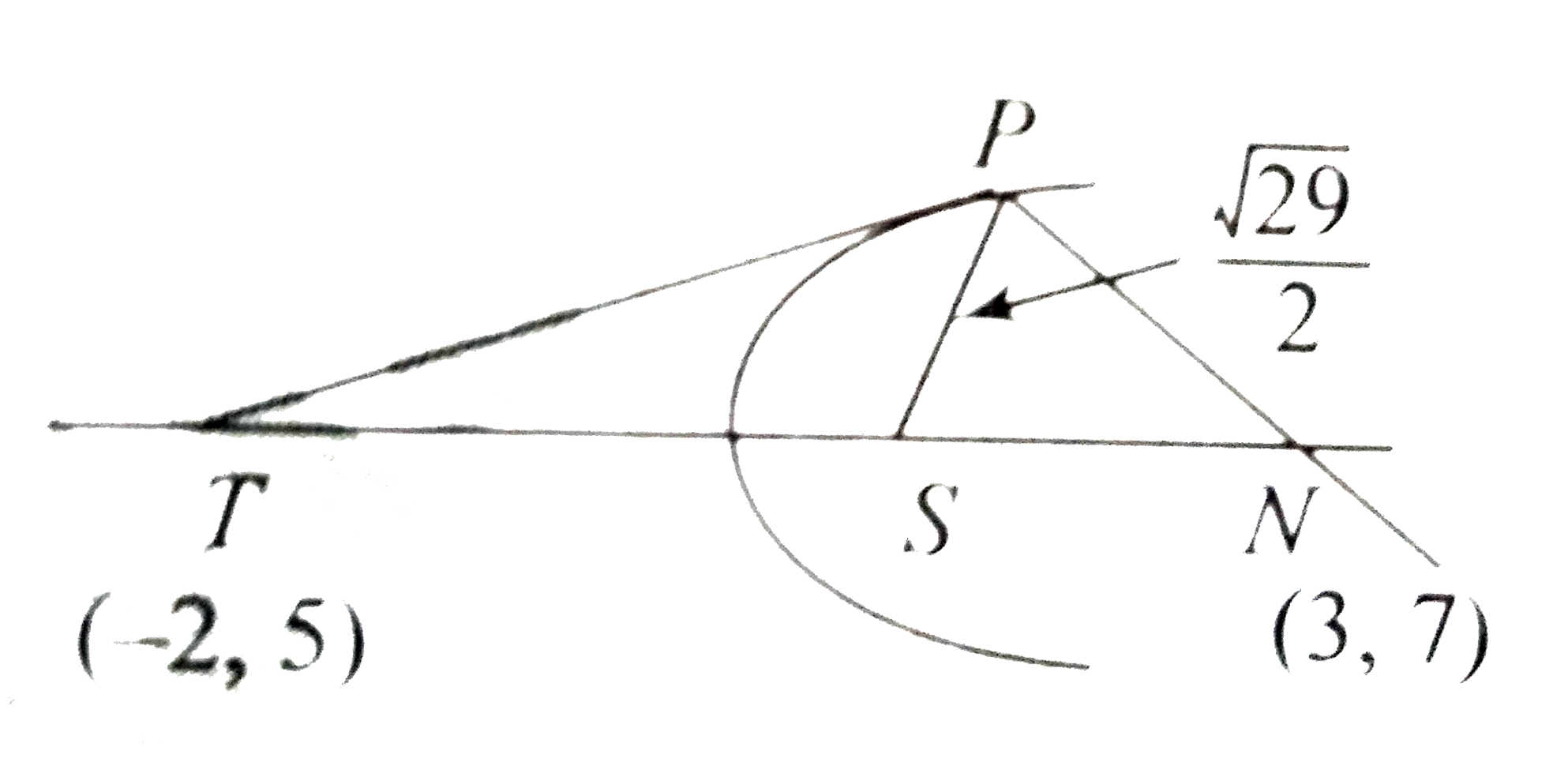
For parabola $y^{2} = 4 a x$ , tangent and normal at point $P (a t^{2}, 2 a t)$ meets x-axis at $T (- a t^{2}, 0)$ and $N (2 a + a t^{2}, 0)$ Thus, focus S is the mid-point of TN.
Also, $S P = S T = S N = a + a t^{2}$
$∴ S P = T N / 2$
For given data, $T N = \sqrt{29}$
$∴ S P = \frac{\sqrt{29}}{2}$

|

Updated on:21/07/2023

Class 12 MATHS PARABOLA

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
One of the points on the parabola $y^{2} = 12 x$ with focal distance 12 is
A(3,6)
B $(9, 6 \sqrt{3})$
C $(7, 2 \sqrt{21})$
D $(8, 4 \sqrt{6})$
Question 2 - Select One
The point of intersection of the tangents of the parabola $y^{2} = 4 x$ drawn at the end point of the chord x+y=2 lies on
Ax-2y=0
Bx+2y=0
Cy-x=0
Dx+y=0

CENGAGE-PARABOLA-Single Correct Answer Type

Home
Ask Doubt
Profile