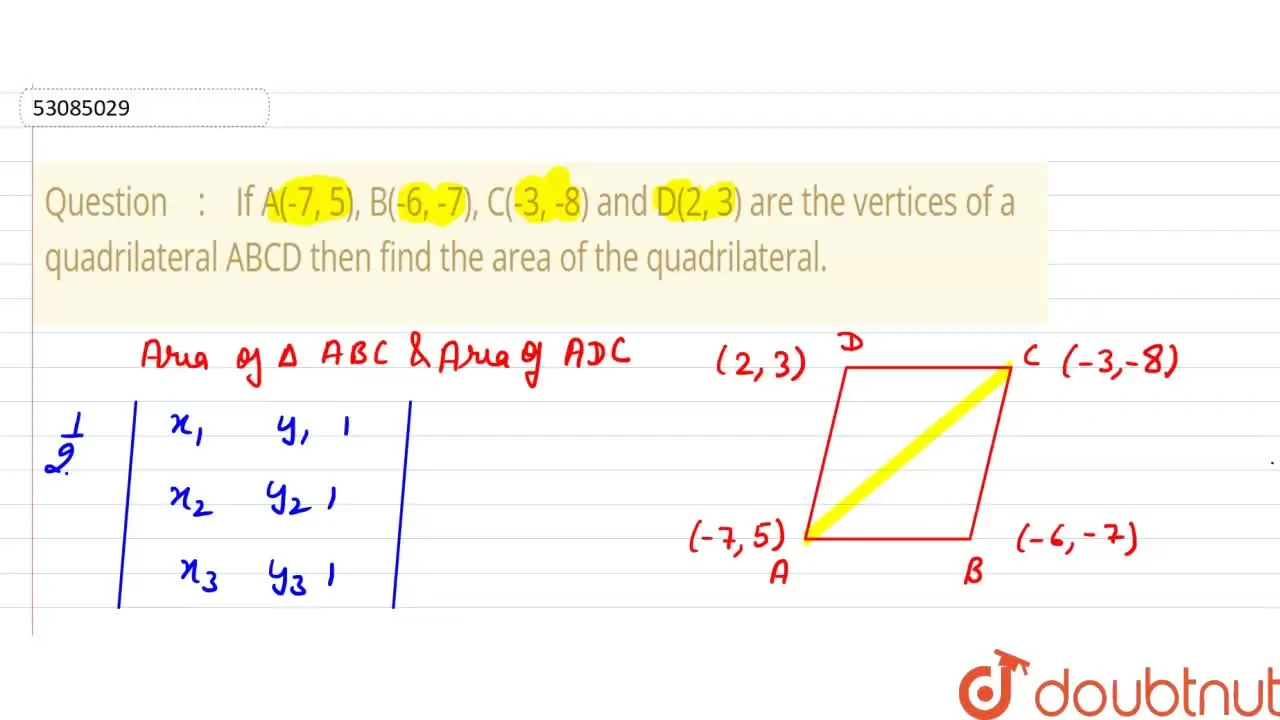
If A(-7, 5), B(-6, -7), C(-3, -8) and D(2, 3) are the vertices of a quadrilateral ABCD then find the area of the quadrilateral.
Step by step video, text & image solution for If A(-7, 5), B(-6, -7), C(-3, -8) and D(2, 3) are the vertices of a quadrilateral ABCD then find the area of the quadrilateral. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 6D17videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)34videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 6B38videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself10videos
CONSTRUCTIONS
- BOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)25videos
HEIGHTS AND DISTANCES
Similar Questions
If A(−3,5),B(−2,−7),C(1,−8)andD(6,3) are the vertices of a quadrilateral ABCD find its area.
View Solutionlf A(-4, 8), B(-3,-4), C(0,-5) and D(5, 6) are the vertices of a quadrilateral ABCD, find its area.
View SolutionIf A (-5, 7), B (-4, - 5), C (-1, - 6) and D (4, 5) are vertices of a quadrilateral, find the area of the quadrilateral ABCD.
View SolutionIf A(−5, 7), B(−4, −5), C(−1, −6) and D(4, 5)are the vertices of a quadrilateral, find the area of the quadrilateral ABCD.
View SolutionIf A(15,14), B(30,15), C(27,5) and D(10,5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD ( in terms of sq. units)
View SolutionLet A(3,2),B(3,2),B(−4,1),C(3,1) and D(2,−4) be the vertices of a quadrilateral ABCD CD Find area of the quadrilateral formed by the mid-points of the sides of quadrilatdateral
View Solution(1, 1), (3, 4), (5, –2) and (4, –7) are vertices of a quadrilateral then find its area.
View SolutionPoints A(4,−1),B(6,0),C(7,2)andD(5,1) are the vertices of the following quadrilateral then the quadrilateral is -
View SolutionPoints A(4,-1) , B(6,0) ,C(7,2) and D(5,1) are the vertices of the following quadrilateral which is a :
View SolutionIf A(−3,4),B(1,−2),C(5,6) and D(x,−4) are the vertices of a quadrilateral such that area of ΔABC=ΔACD then x=
View Solution