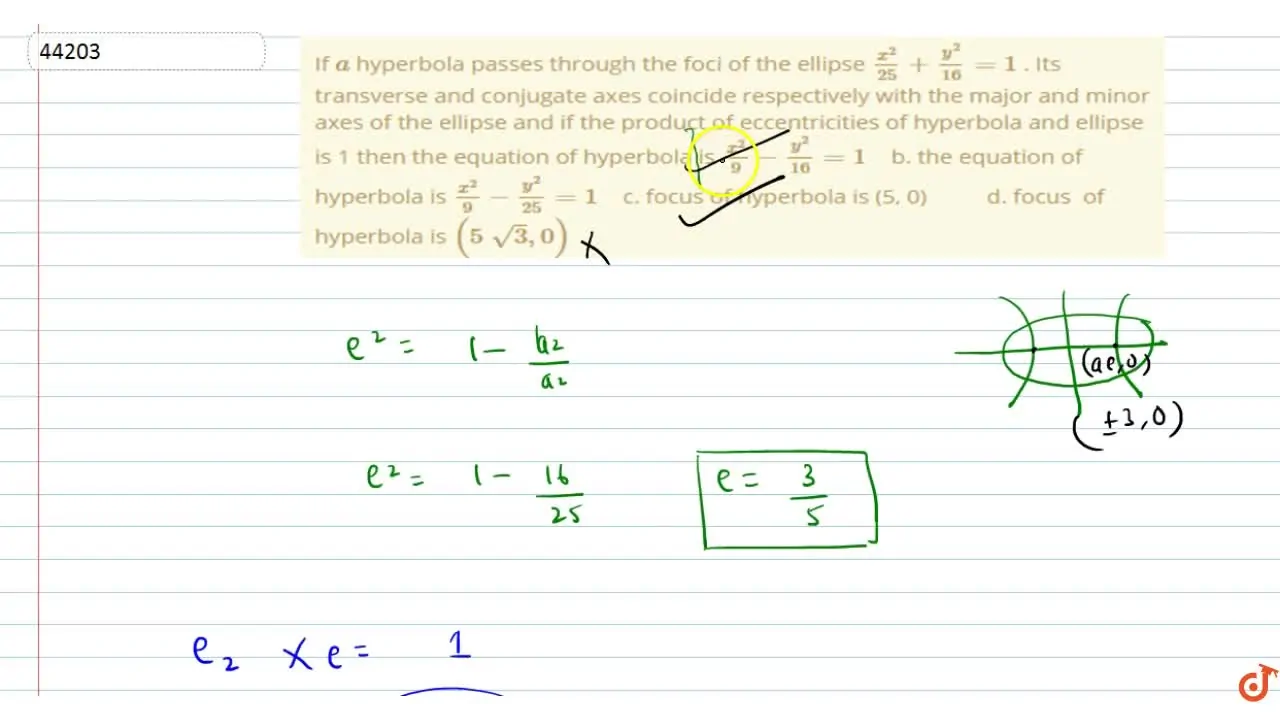
If a hyperbola passes through the foci of the ellipse x225+y216=1 . Its transverse and conjugate axes coincide respectively with the major and minor axes of the ellipse and if the product of eccentricities of hyperbola and ellipse is 1 then the equation of a. hyperbola is x29−y216=1 b. the equation of hyperbola is x29−y225=1 c. focus of hyperbola is (5, 0) d. focus of hyperbola is (5√3,0)
Here, equation of ellipse is,
x225+y216=1
So, if e is the essentricity of ellipse, then,
e2=1−b2a2=1−1625=925
e=35
We know, foci of ellipse is (ae,0). So, focus of given ellipse will be (3,0).
Now, let equation of hyperbola is,
x2a2−y2b2=1
Now, as hyperbola passes through the foci of the given ellipse(3,0),
We can say that.,9a2−0=1⇒a2=9
Also, we are given product of eccentricity of ellipse and hyperbola is 1. If eccentricity of hyperbola is eh, then,
35∗eh=1⇒eh=53
As we know, e2h=1+b2a2
Putting, values of ehanda2, we get,
b2=16
So, equation of hyperbola is x29−y216=1
Now, focus of hyperbola is (±aeh,0)=(±5,0)
That means, option B and option C are correct.
Related Playlists
Similar Questions
A hyperbola passes through a focus of the ellipse x2169+y225=1. Its transverse and conjugate axes coincide respectively with the major and minor axes of the ellipse.The product of eccentricities is 1. Then the equation of the hyperbola is
View SolutionIf a hyperbola passes through the focus of the ellipse x225+y216=1 and its transverse and conjugate gate axis coincides with the major and minor axis of the ellipse, and product of their eccentricities is 1, then
View SolutionLet a hyperbola passes through the focus of the ellipse x225+y216=1. The transverse and conjugate axes of this hyperbola coincide with the major and minor axes of the given ellipse, also the product of eccentricities of given ellipse and hyperbola is 1, then
View SolutionIf a hyperbola passes through foci of the ellipse x252+y232=1 and its transverse and conjugate axes coincide with the major and minor axes of the ellipse and the product of their eccentricities is 1, then the product of length of semi transverse and conjugate axes of hyperbola is...
View SolutionThe foci of the hyperbola x29+y216=1 are-
View SolutionThe foci of the hyperbola x216−y29=1 is :
View SolutionThe eccentricity of the hyperbola x225−y216=1 is
View SolutionIf the foci of the ellipse x216+y2b2=1 and the hyperbola coincide then b2 equals
View SolutionIf the equation to the hyperbola is 3x2−5xy−2y2+5x+11y−8=0 then equation to the conjugate hyperbola is
View SolutionLet a hyperbola passes through the focus of the ellipse x225−y216=1. The transverse and conjugate axes of this hyperbola coincide with the major and minor axes of the given ellipse, also the product of eccentricities of given ellipse and hyperbola is 1, then
View Solution