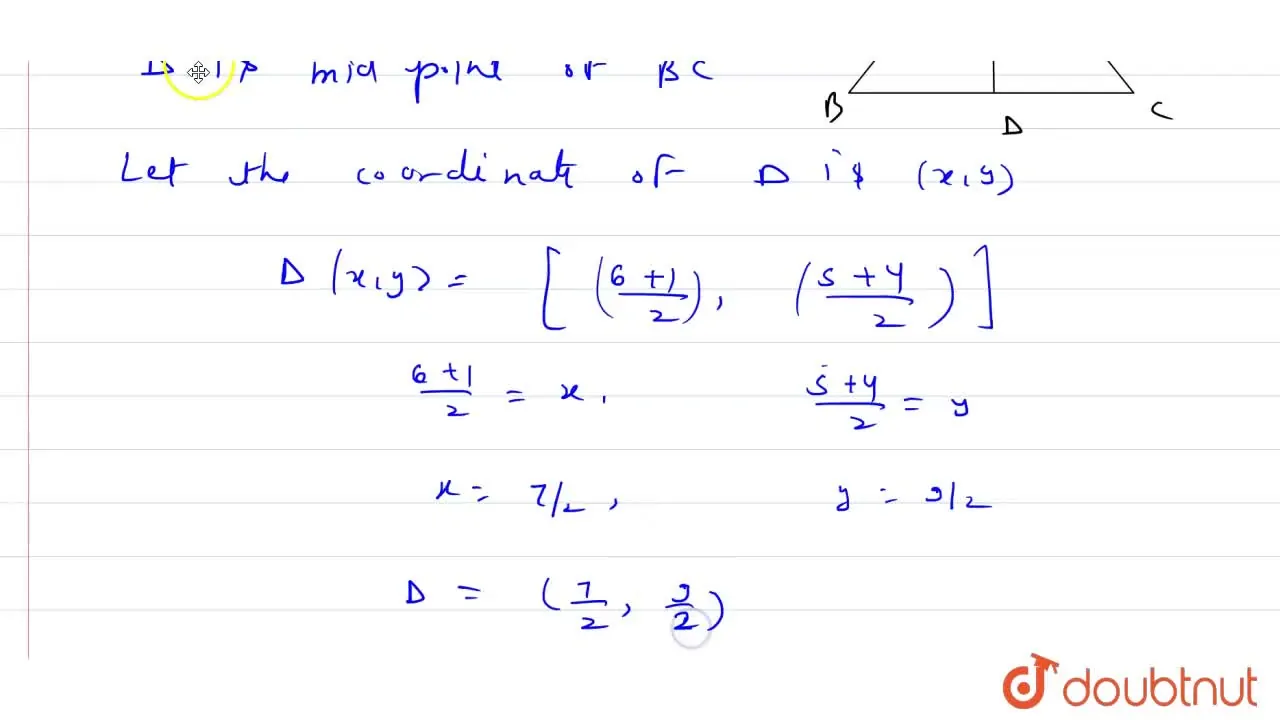
If A(4, 2), B(6, 5) and C(1, 4) be the vertices of ΔABC and AD is a median, then the coordinates of D are
(52,3)
(5,72)
(72,92)
none of these
The correct Answer is:C
Midpoint of BC is D(6+12,5+42)=D(72,92)
Related Playlists
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 6D17videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself10videos
CONSTRUCTIONS
- BOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)25videos
HEIGHTS AND DISTANCES
Similar Questions
If A (4, 2), B (7, 6) and C (1, 4) are the vertices of a ΔABC and AD is its median, prove that the median AD divides ΔABC into two triangles of equal areas.
View SolutionLet A (4,2) , B (6,5) and C (1,4) be the vertices of ΔABC.
What do you observe?View SolutionA(7, -3), B(5, 3) and C(3, -1) are the vertices of a ΔABC and AD is its median. Prove that the median AD divides ΔABC into two triangles of equal areas.
View SolutionIf A(4,−6),B(3,−2)andC(5,2) are the vertices of a ΔABCandAD is its median, prove that the median AD divides ΔABC into two triangles of equal areas.
View SolutionA(4, 2), B(6,5) and C(1, 4) are the vertices of ABC . The median from A meets BC in D . Find the coordinates of the point D .
View SolutionIf A(0,0),B(2,4)andC(6,4) are the vertices of a ΔABC, find theof its sides.
View SolutionLet A (4, 2), B(6, 5) and C(1, 4) be the vertices of ΔABC.
(i) The median from A meets BC at D. Find the coordinates of the point D.
(ii) Find the coordinates of the point P on AD such that AP : PD = 2 : 1
(iii) Find the coordinates of points Q and R on medians BE and CF respectively such that BQ : QE = 2 : 1 and CR : RF = 2 : 1.
(iv) What do you observe?
(v) If A(x1,y1),B(x2,y2) and C(x3,y3) are the vertices of ∆ ABC, find the coordinates of the centroid of the triangle.View SolutionA(4,2), B(6,5) and C(1,4) are the vertices of △ABC Using centroid formula , find coordinates of centroid G .
View Solution7. Let A (4,2) B(6.,5) and C(1.,4). be the vertices of ΔABC. (1) The median from A meets BC at D. Find the coordinates of the point D. (2) the coordinates of the point P on AD such that AP:PD=2:1. (3) Find the coordinates of points Q and R on medians BE and CF respectively such that BQ:QE=2:1 and CR:RF=2:1. (4) what do yo observe?
View SolutionLet A(5,6), B(-2,3) and C(6,-1) be the vertices of ΔABC. Find the coordinates of the centroid of the triangle.
View Solution