If D, E and F be the middle points of the sides BC,CA and AB of the ΔABC, then AD+BE+CF is
0
0
2
None of these
The correct Answer is:A
Let the position vectors A, B, C be a, b and c respectively . Then the position vectors of mid-points D, E and F are
b+c2 ,(c+a)/( 2) and (a+b) /(2)repectively.<br>NowAD +BE +CF<br>={(b+c)/(2)-a}+{(c+a)/(2)-b}+{(a+b)/(2)-c )}=0
Topper's Solved these Questions
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - VECTORS EXERCISE - (VECTORS ) Exercise 2 ( Topical problems )88videos
VECTORS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - VECTORS EXERCISE - ( MHT CET Corner)66videos
VECTORS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - TRIGONOMETRIC FUNCTIONS OF COMPOUND ANGLESEXERCISE - Exercise 2 (MISCELLANEOUS PROBLEMS)20videos
TRIGONOMETRIC FUNCTIONS OF COMPOUND ANGLES
Similar Questions
Knowledge Check
- Question 1 - Select One
Let D, E and F be the middle points of the sides BC,CA and AB respectively of a triangle ABC. Then, AD + BE + CF equals to
A0B0C2DNone of these - Question 2 - Select One
If D,E and F are the mid-points of the sides BC,CA and AB, respectively of a ΔABC and O is any point, show that
(i) AD+BE+CF=0
(ii) OE+OF+DO=OAAA. a zero vectorBB. a unit vectorCC. 0DD. none of these - Question 3 - Select One
In the given figure, D, E and F are the midpoints of the sides BC, CA and AB of ΔABC.BE bisect DF at X while CF bisect DE at Y, then BC = a XY. Find a.
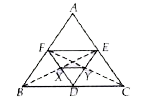 A3B4C2D1/4
A3B4C2D1/4
Let D,EandF be the middle points of the sides BC,CAandAB, respectively of a triangle ABC. Then prove that →AD+→BE+→CF=→0 .
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, CA and AB of the equilateral ΔABC. Prove that ΔDEF is also an equilateral triangle.
 View Solution
View SolutionD, E and F are the mid-points of the sides BC, CA and AB of a triangle ABC. Show that
BDEF is a parallelogramView SolutionIf D,E,andF are three points on the sides BC,AC,andAB of a triangle ABC such that AD,BE,andCF are concurrent, then show that BD⋅CE⋅AF=DC⋅EA⋅FB .
View SolutionIf D, E and F are respectively the mid - points of sides BC, AC and AB of a ΔABC. If EF=3cm,FD=4cm and AB=10cm, then DE,BCandCA respectively will be equal to :
View Solution

