IF n is a constant equal to the number of degrees in an angle measuring 3π radians, what is the value of n?
240
320
270
540
The correct Answer is:540
To find the value of n, which represents the number of degrees in an angle measuring 3π radians, we will follow these steps:
Step 1: Understand the conversion from radians to degrees
To convert radians to degrees, we use the conversion factor:
1 radian=180π degrees
Step 2: Set up the conversion for 3π radians
We need to convert 3π radians into degrees. Using the conversion factor, we can express this as:
n=3π×180π
Step 3: Simplify the expression
Now, we can simplify the expression:
n=3×180
Step 4: Calculate the value of n
Now we perform the multiplication:
n=540
Conclusion
Thus, the value of n is:
n=540 degrees
Final Answer
The answer is n=540.
---
To convert radians to degrees, we use the conversion factor:
1 radian=180π degrees
We need to convert 3π radians into degrees. Using the conversion factor, we can express this as:
n=3π×180π
Now, we can simplify the expression:
n=3×180
Now we perform the multiplication:
n=540
Thus, the value of n is:
n=540 degrees
The answer is n=540.
Topper's Solved these Questions
Similar Questions
Knowledge Check
What is the angle measure of 4 radians ?
A114.591∘B141.372∘C229.183∘D282.743∘What is the measure in degrees of an angle that is π4 radians?
A4∘B25∘C45∘D90∘What is the degree measure of an angle that measures 7π15 radians ?
A(360−7π15)∘B(180−7π15)∘C252∘D84∘
The angles of a triangle are in A.P. and the number of degrees in the least angle is to the number of degrees in the mean angle as 1:120. Find the angles in radians.
View SolutionThe angles of a triangle are in A.P. The number of degrees in the least is to the number of radians in the greatest as 60:π. Find the angles in degrees.
View Solution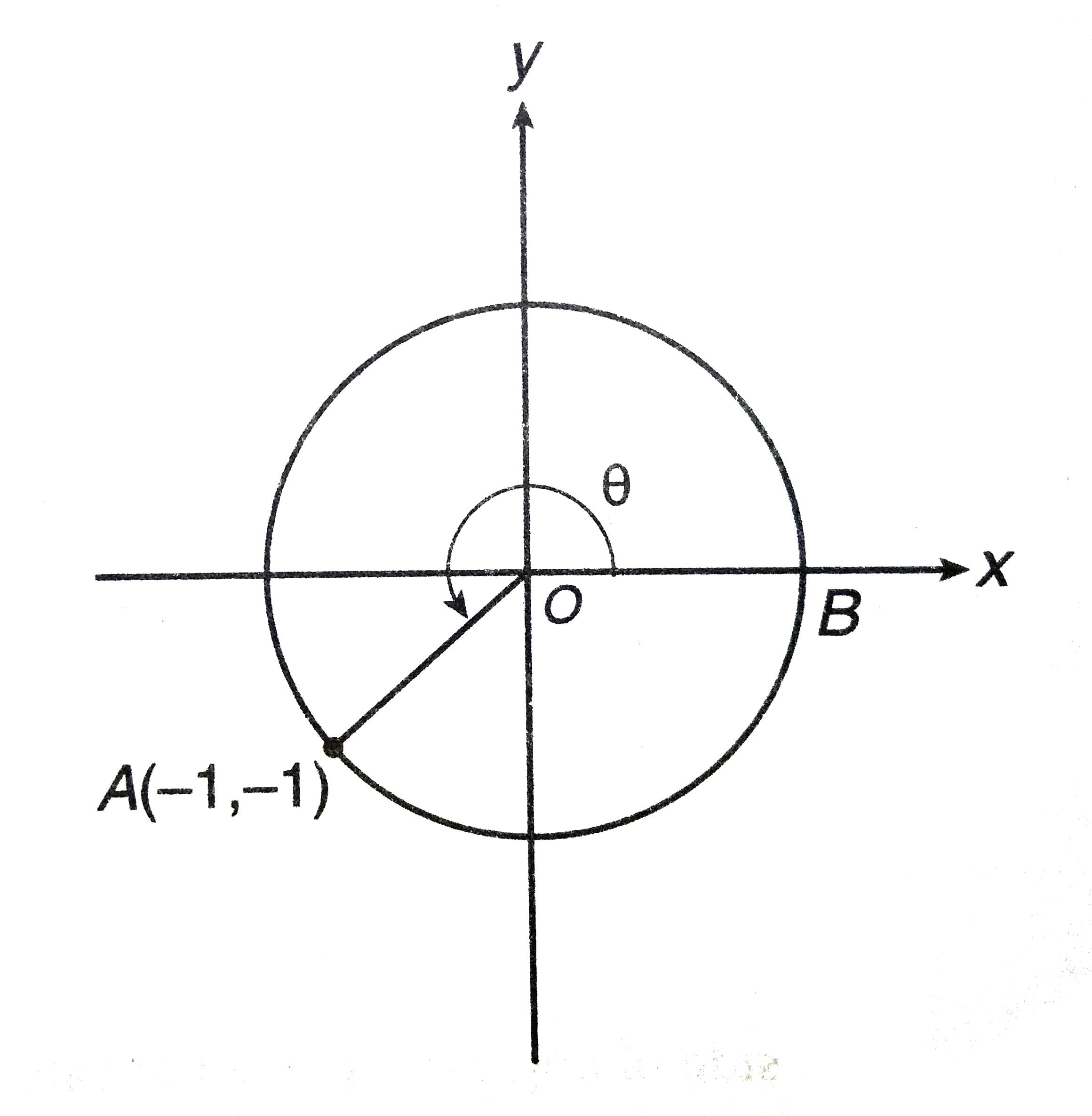
In the xy-plane above, O is the center of the circle, and the measure of angle θ is kπ radians. If 0≤θ≤2π, what is the value of k?
View Solution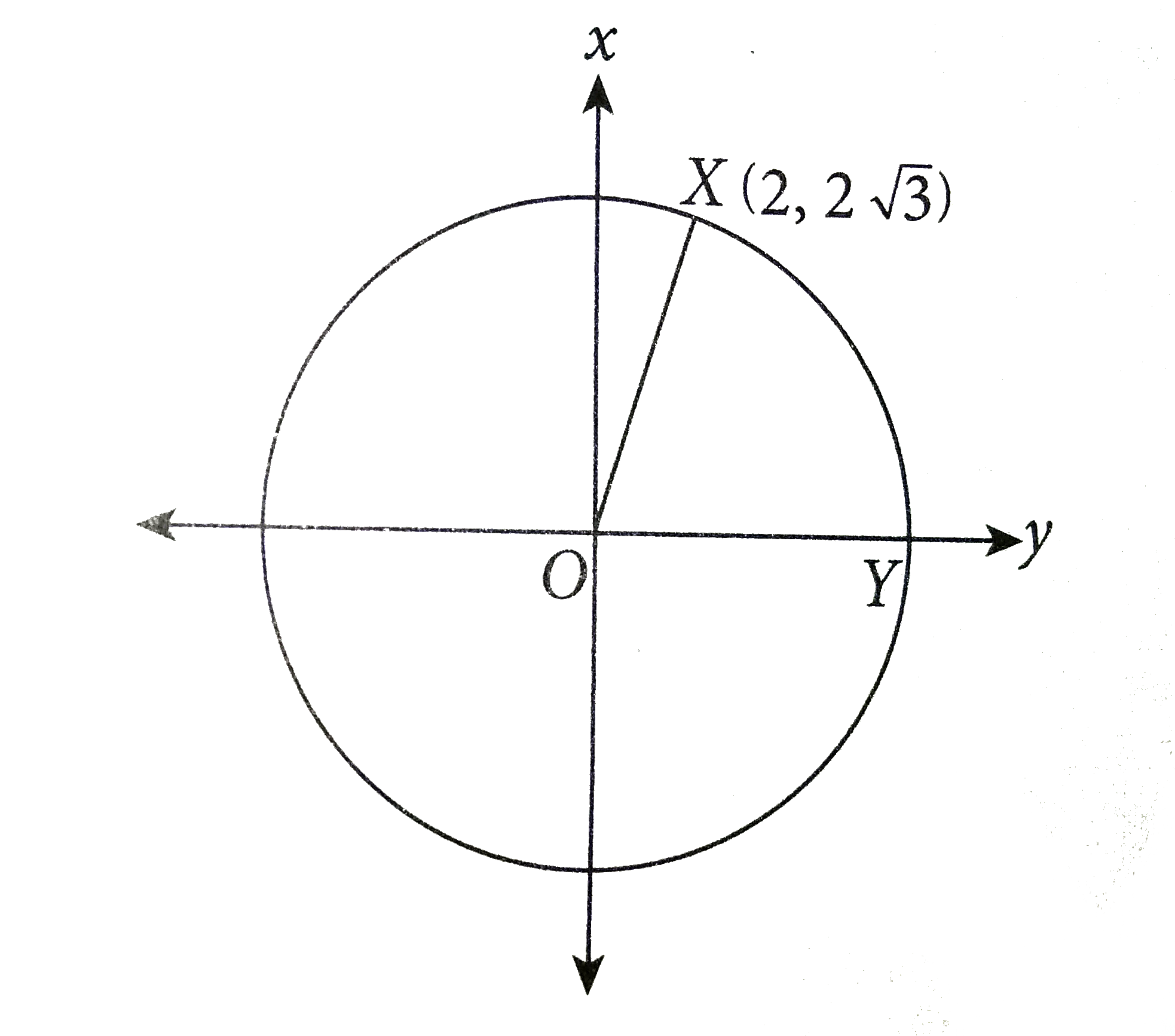
In the xy-plane above, the circle has center O, and the measure of ∠XOY is πn radians. What is the value of n?
View Solution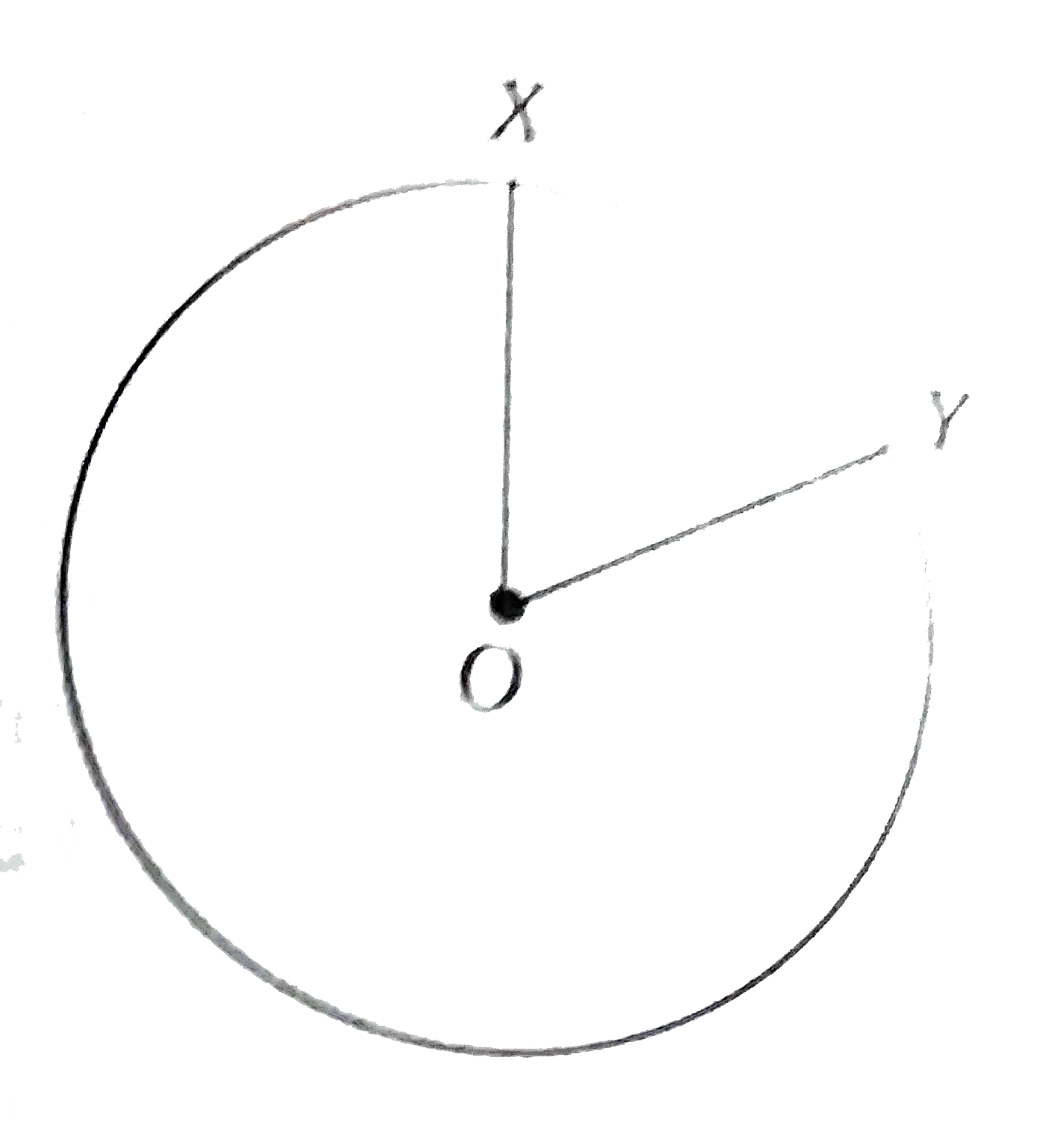
In the figure above, circle O has a radius of 8, and angle XOY measures 516π radians. What is the measure of minor are XY?
View Solution