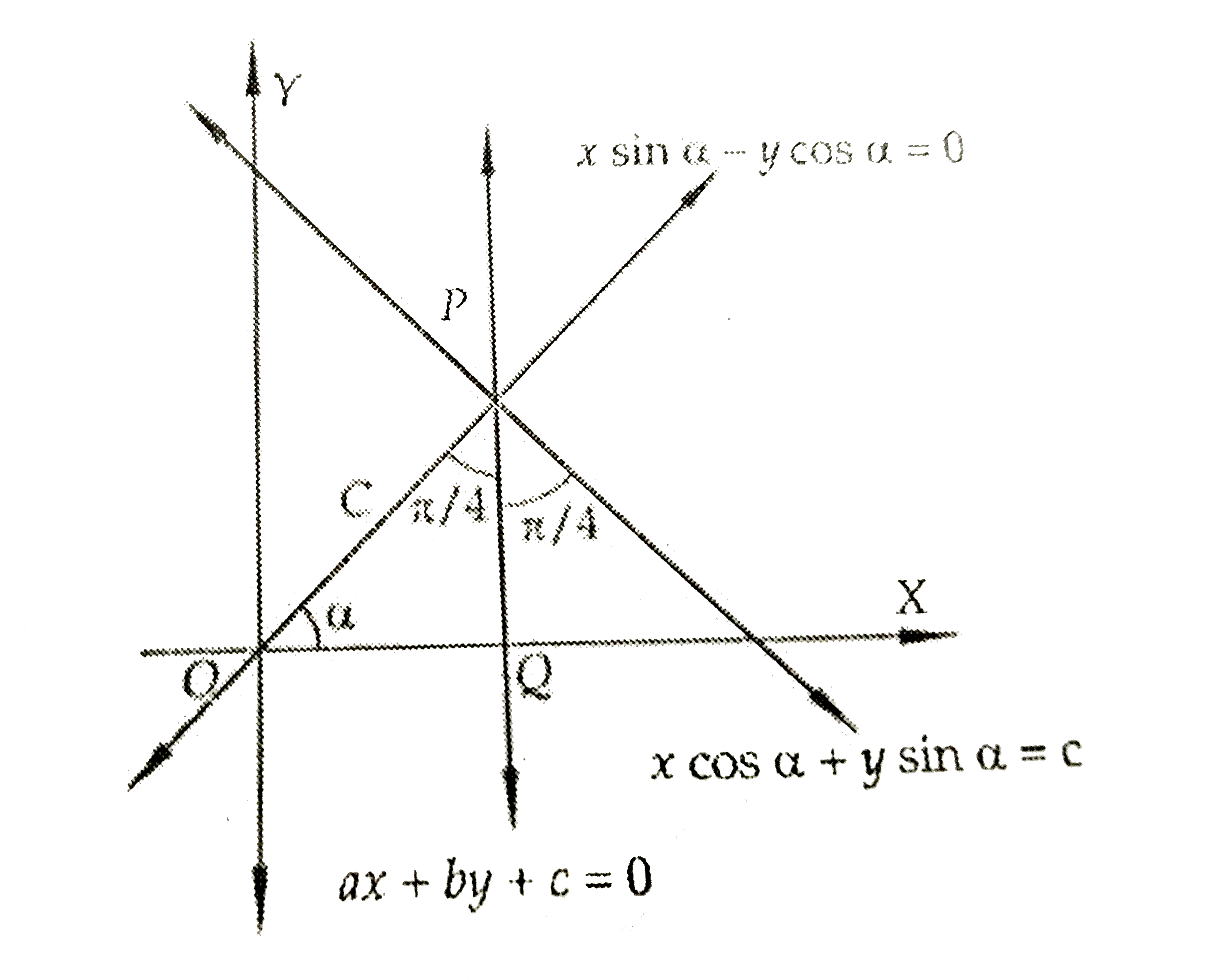
If the straight lines $a x + b y + p = 0 and x cos α + y sin α = c$ enclose an angle $π / 4$ between them and meet the straight line $x sin α - y cos α = 0$ in the same point , then

A

$a^{2} + b^{2} = c^{2}$

B

$a^{2} + b^{2} = c^{2}$

C

$a^{2} + b^{2} = 2 c^{2}$

D

$a^{2} + b^{2} = 4$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

The straight lines $x cos α + y sin α = c$ and $x sin α - y cos α = 0$ are mutually perpendicular . Line $x cos α + y sin α = c$ is in normal form.So , perpendicular from the orgin as this line is of length c and makes angle $π / 4$ with OX.
The line sin $α - y cos α = 0$ passes through the origin and makes angle $α$ with x-axis. So,it is along the perpendicular from the origin on $x cos α + y sin α = c$ . Line $a x + b y + c = 0$ makes angle $π / 4$ with $x cos α + y sin α = c$ and $x cos α + y sin α = c$ and $x sin α - y cos α = 0$ are mutually perpendicular. So , $a x + b y + c = 0$ is a bisector of the angle between them.
In $D e t l a O P Q$ , we have
$O Q = P O sin . \frac{π}{4} = \frac{c}{\sqrt{2}}$
Also,
OQ= Lenght of the perpendicular from (0,0) on
$a x + b y + c = 0$
$\Rightarrow O Q = \frac{c}{\sqrt{a^{2} + b^{2}} \Rightarrow \frac{c}{\sqrt{2}} = \frac{c}{\sqrt{a^{2} + b^{2}} \Rightarrow a^{2} + b^{2} = 2}}$

Updated on:21/07/2023

|

Class 11 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

OBJECTIVE RD SHARMA ENGLISH-STRAIGHT LINES-Section I - Solved Mcqs

Home
Ask Doubt
Profile