Question

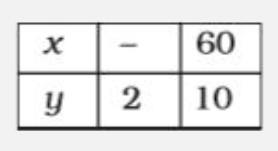
If x varies inversely as y and x = 4 when y = 6, then when x = 3 the value of y is _______.

Options

A. 4
B. 8
C. 5
D. 6

Answer

B

Explanation

To solve the problem where $$x$$ varies inversely as $$y$$, we can follow these steps:

Step 1: Understand the relationship
Since \( x \) varies inversely as \( y \), we can express this relationship mathematically as:
\( x \cdot y = k \)
where \( k \) is a constant.

Step 2: Find the constant \( k \)
We are given that \( x = 4 \) when \( y = 6 \). We can substitute these values into the equation to find \( k \):
\( k = x \cdot y = 4 \cdot 6 = 24 \)

Step 3: Use the constant to find \( y \) when \( x = 3 \)
Now we need to find the value of \( y \) when \( x = 3 \). We can use the constant \( k \) we found in the previous step:
\( x \cdot y = k \implies 3 \cdot y = 24 \)

Step 4: Solve for \( y \)
To find \( y \), we can rearrange the equation:
\( y = \frac{24}{3} = 8 \)

Conclusion
Thus, when \( x = 3 \), the value of \( y \) is:
\( \boxed{8} \)