In a triangle ABC, if acosA=bcosB, show that the triangle is either isosceles or right angled.
From sine law, we have,
asinA=bsinB=csinC=k
⇒a=ksinA,b=ksinB,c=ksinC
Now, it is given that ,
acosA=bcosB
⇒ksinAcosA=ksinBcosB
⇒sinAcosA=sinBcosB
⇒2sinAcosA=2sinBcosB
⇒sin2A=sin2B
⇒sin2A−sin2B=0
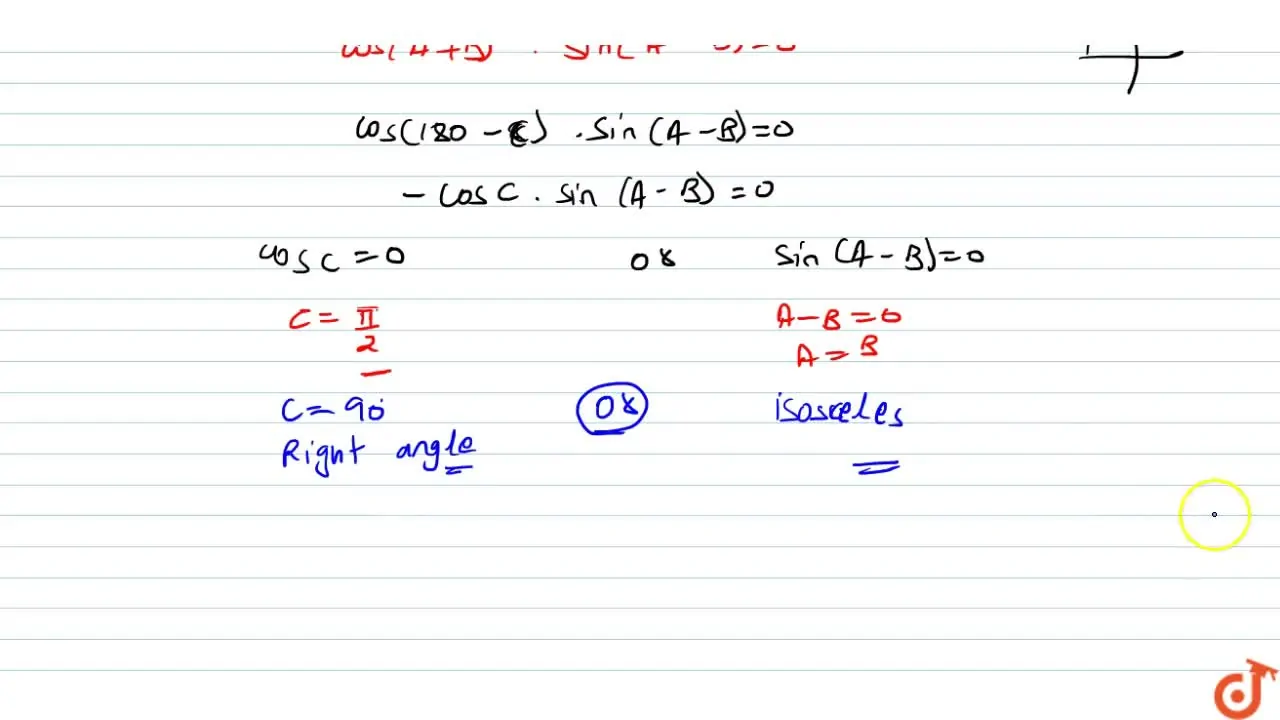
⇒2sin(A−B)cos(A+B)=0
⇒sin(A−B)cos(π−C)=0...[AsA+B+C=π]
⇒−sin(A−B)cosC=0
⇒cosC=0orsin(A−B)=0
⇒C=π2orA−B=0
⇒C=π2orA=B
It means, either the ΔABC is a right angle triangle or a isoceles triangle.
Related Playlists
Similar Questions
In a triangle ABC, if acosA=bcosB, show that the triangle is either isosceles or right angled.
View SolutionIf acosA=bcosB, prove that the ΔABC is either isosceles or right angled.
View SolutionIn a triangle ABC , if cosA=sinB2sinC , show that the triangle is isosceles.
View SolutionIn △ABC, if acosA=bcosB, then the triangle is
View SolutionThe angles A, B, C of a triangle ABC satisfy 4cosAcosB+sin2A+sin2B+sin2C=4, Then which of the following statements is/are correct? (1) The triangle ABC is right angled (2) The triangle ABC is isosceles (3) The triangle ABC is neither isosceles nor right angled (4) The triangle ABC is equilateral
View SolutionIn a ΔABC,ifcosAa=cosBb,show that the triangle is isosceles.
View SolutionIn any triangle ABC, if cosA+2cosCcosA+2cosB=sinBsinC then prove that, the triangle is either isosceles or right angled.
View SolutionIn any triangle ABC if 2cosB=ac, then the triangle is(A) Right angled (C) Isosceles (B) Equilateral (D) None of these
View SolutionIn ΔABC, if cosAa=cosBb, then show that it is an isosceles triangle.
View SolutionJoinA→D
Hence,AD∥BC
∠ACB=∠CAD(alternateangle
∠BAC=∠ACD(alternateangle)
HenceAB=AC (by ASA congurency )so, it is isosceles triangle.View Solution