In in another triangle ABC, and AC = 6 cm. find XZ, given that .
The correct Answer is:6 cm
To solve the problem, we need to find the length of side XZ in triangle XYZ, given that triangle XYZ is congruent to triangle ABC.
1. Identify the Angles in Both Triangles:
- In triangle XYZ:
- Angle X = 45°
- Angle Y = 75°
- Angle Z can be calculated as:
Angle Z=180°−Angle X−Angle Y=180°−45°−75°=60°
- In triangle ABC:
- Angle A = 45°
- Angle C = 60°
- Angle B can be calculated as:
Angle B=180°−Angle A−Angle C=180°−45°−60°=75°
2. Establish the Congruence:
- Since triangle XYZ is congruent to triangle ABC (∆XYZ ≅ ∆ABC), the corresponding angles and sides are equal.
- Therefore, we have:
- Angle X corresponds to Angle A
- Angle Y corresponds to Angle B
- Angle Z corresponds to Angle C
3. Use Corresponding Parts of Congruent Triangles (CPCT):
- According to the properties of congruent triangles, the corresponding sides are equal.
- We know that AC = 6 cm in triangle ABC.
- Thus, by CPCT:
XZ=AC
- Therefore:
XZ=6 cm
4. Final Answer:
- The length of side XZ is 6 cm.
Summary:
Thus, the length of XZ in triangle XYZ is 6 cm.
---
- In triangle XYZ:
- Angle X = 45°
- Angle Y = 75°
- Angle Z can be calculated as:
Angle Z=180°−Angle X−Angle Y=180°−45°−75°=60°
- In triangle ABC:
- Angle A = 45°
- Angle C = 60°
- Angle B can be calculated as:
Angle B=180°−Angle A−Angle C=180°−45°−60°=75°
- Since triangle XYZ is congruent to triangle ABC (∆XYZ ≅ ∆ABC), the corresponding angles and sides are equal.
- Therefore, we have:
- Angle X corresponds to Angle A
- Angle Y corresponds to Angle B
- Angle Z corresponds to Angle C
- According to the properties of congruent triangles, the corresponding sides are equal.
- We know that AC = 6 cm in triangle ABC.
- Thus, by CPCT:
XZ=AC
- Therefore:
XZ=6 cm
- The length of side XZ is 6 cm.
Thus, the length of XZ in triangle XYZ is 6 cm.
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Revision Exercise (short Answer Type Question)
10 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Revision Exercise (long Answer Type Question)
8 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7b
17 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
In △ABC,AB=10cm,∠A=45∘ and ∠C=60∘. Find BC.
View SolutionIn ΔABC,∠A=600,∠B=45∘, find a:b.
View SolutionConstruct a triangle ABC, in which ∠B=60∘ , ∠C=45∘ and AB + BC + CA = 11 cm.
View SolutionIn a ΔABC, if ∠A=45∘.∠B=60∘and∠C=75∘, find the ratio of its sides.
View SolutionFind the area of ΔABC, if a=10cm, ∠B=45∘ and ∠C=45∘
View SolutionFind the area of ΔABC, if a=10cm, ∠B=45∘ and ∠C=45∘
View SolutionConstruct a triangle ABC in which BC = 8cm, ∠B=45∘ and AB - AC = 3.5 cm.
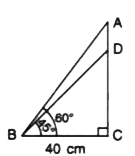
View SolutionFind the length of AD.
Given : ∠ABC=60∘,
∠DBC=45∘
and BC=40 cm.
View SolutionIn triangle ABC, ∠B=90∘ and tan A = 0.75 If AC = 30 cm, find the lengths of AB and BC.
View SolutionConsider a ΔXYZ such
XY=4.5cm,∠X=60∘and∠Y=45∘.Find angle Z.
View Solution
