In the adjoining figure, AB = CD and AB||CD prove that
(i) ΔAOB≅ΔDOC
(ii) AD and BC bisect each other at the point O.
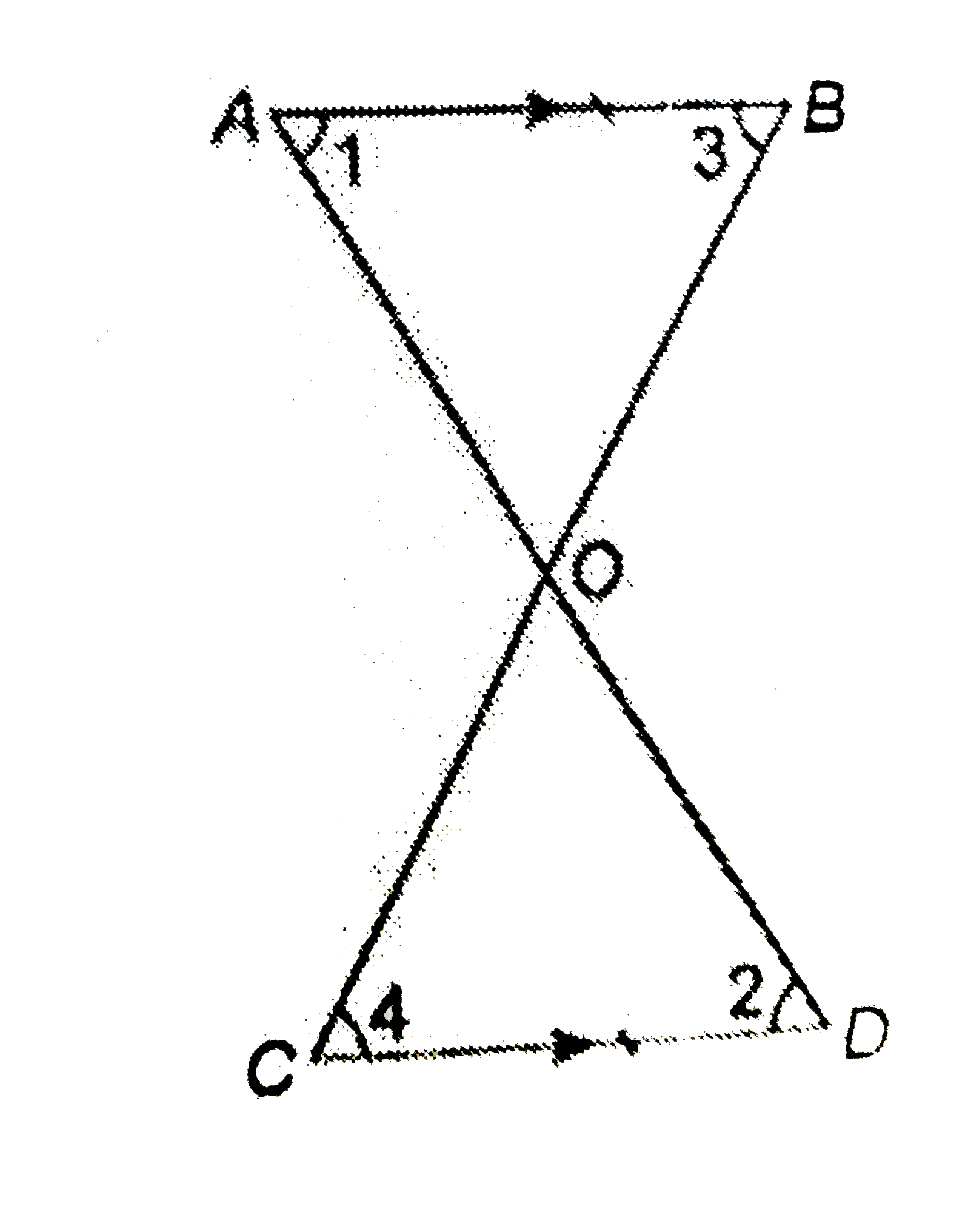
(i) since AB||CD (given)
⇒ ∠1=∠2 and ∠3=∠4 (alternate angles)
Now, in ΔAOB and ΔDOC,
∵⎧⎪⎨⎪⎩∠1=∠2(alternate angles)AB=DC(given)∠3=∠4(alternate angle)
Therefore, ΔAOB≅ΔDOC (ASA) Hence Proved.
(ii) Therefore, AO=DO (c.p.c.t.)
⇒BC bisects AD.
Also BO=CO (c.p.c.t)
⇒AD bisects BC.
Hence, AD and BC bisect each other. Hence Proved.
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7a
35 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
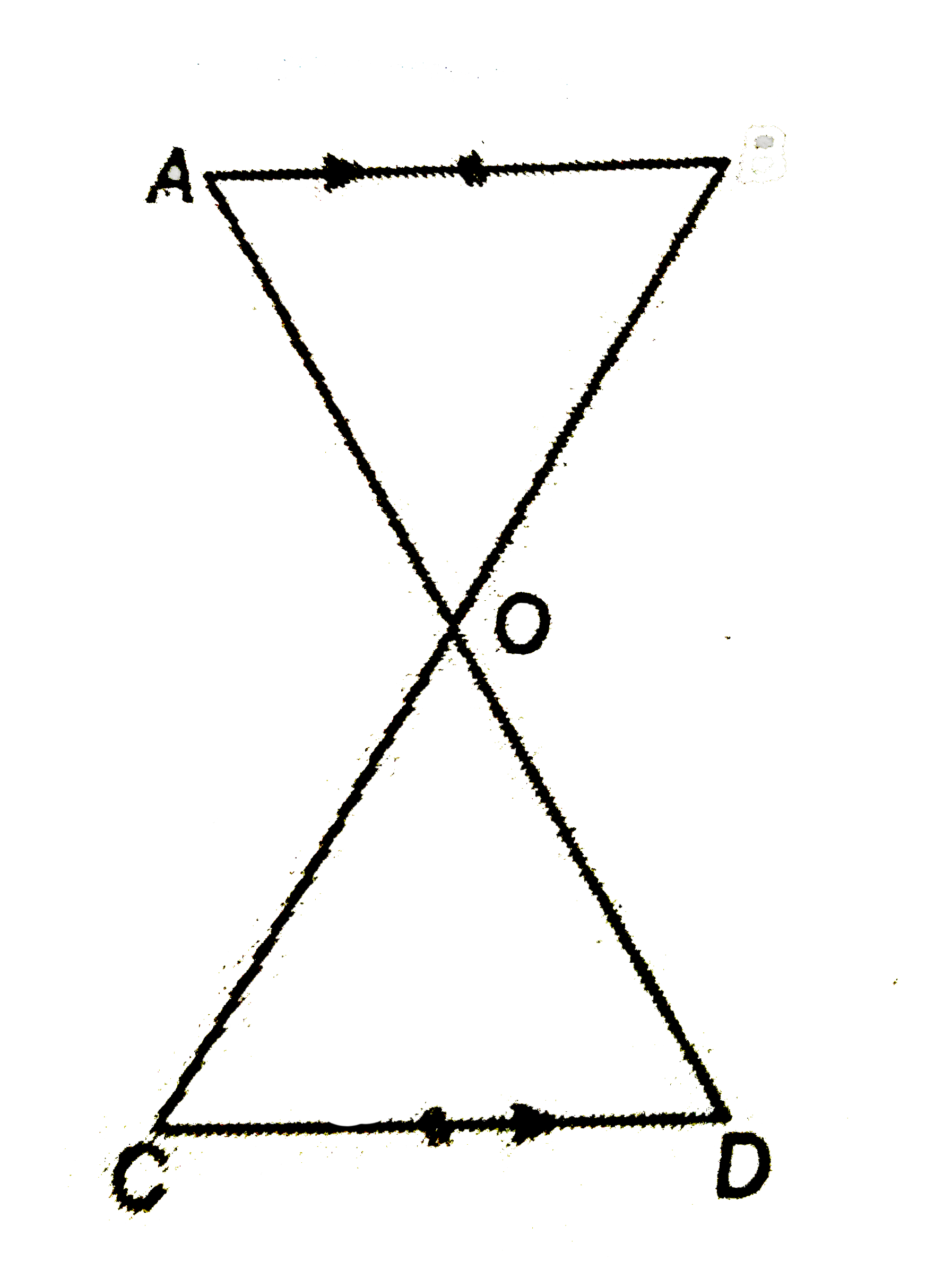
In the adjoining figure AB=CD and AB||CD prove that :
(i) ΔAOB≅ΔDOG
(ii) AD and BC bisect each other at point O.
View SolutionIn the adjoining figure AB||CD, find x and y

View SolutionIn the given figure, AB∣∣CD and AB=CD, prove that: ΔAOB≅ΔDOC

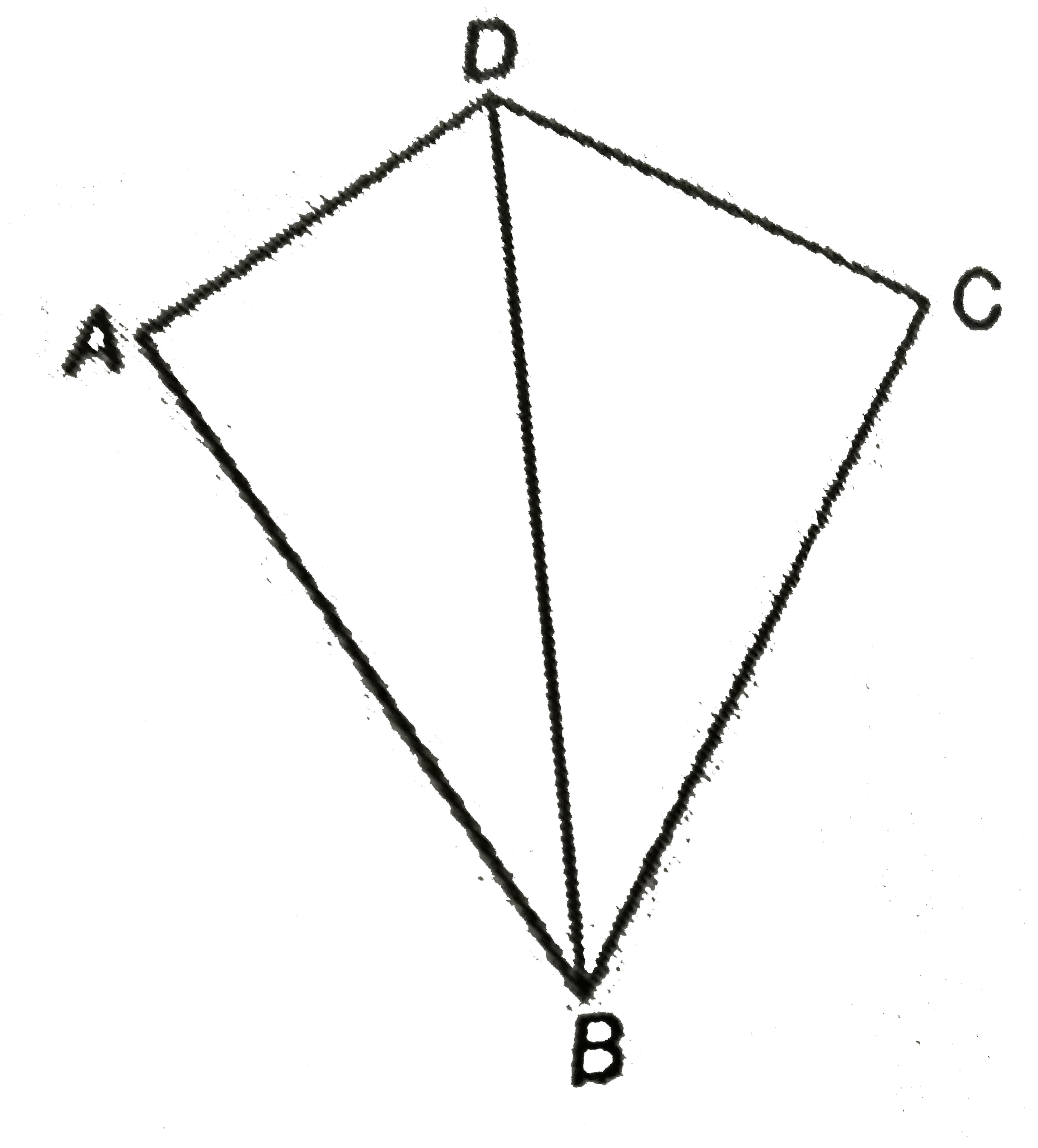
View SolutionIn the adjoining figure, AD=DC and DB bisects ∠ADC. Prove that ΔADB≅ΔCDB.
View SolutionIn the given figure, AB⊥BD,CD⊥BD and AB=CD. Prove that :
(i) ΔABD≅ΔCDB
(ii) AD-CB

View SolutionIn the given figure, AB=CD and AD=CB. Prove that :
(i) ΔABD=ΔCDB
(ii) ∠A=∠C

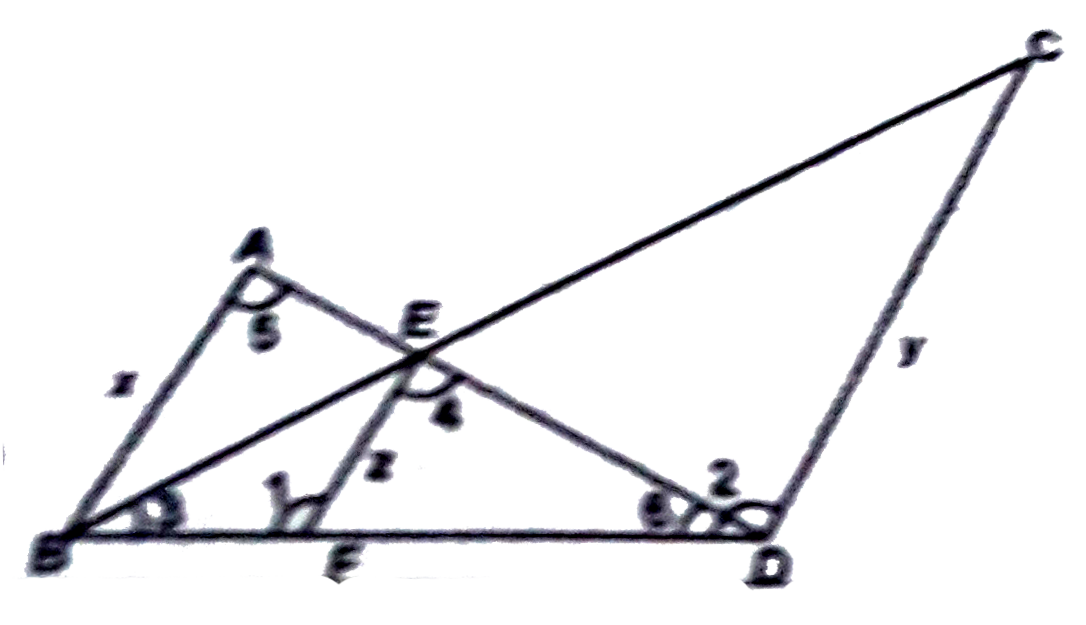
View SolutionIn the adjoining figure , AB||CD||EF.
prove that 1x+1y=1z.
View SolutionIn the following figure, OA=OC and AB=BC.
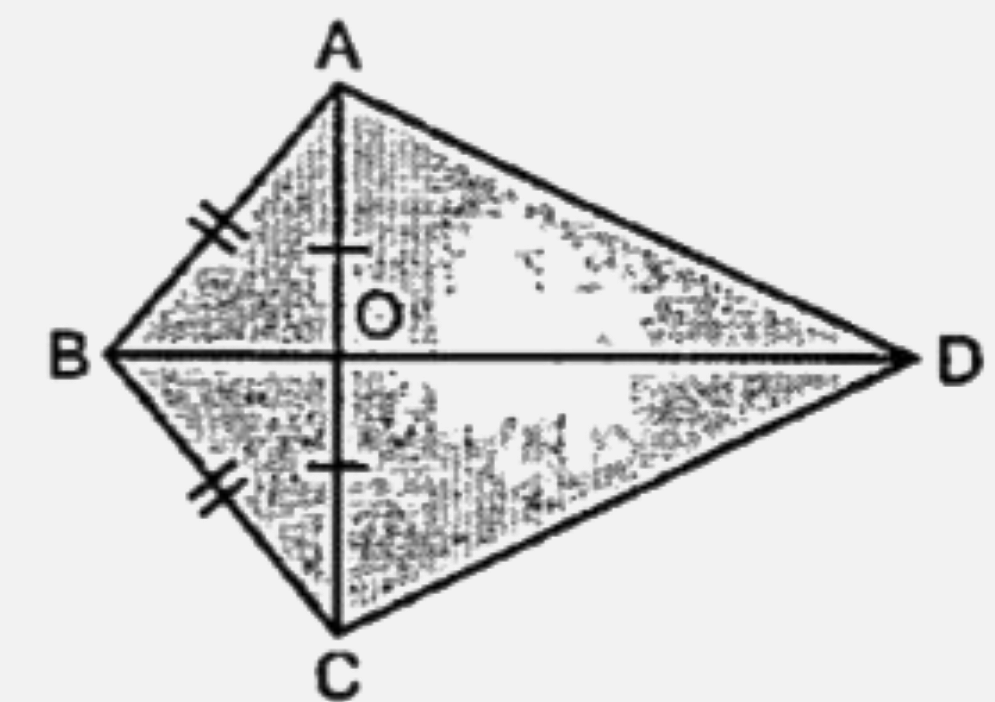
Prove that :
AD=CD
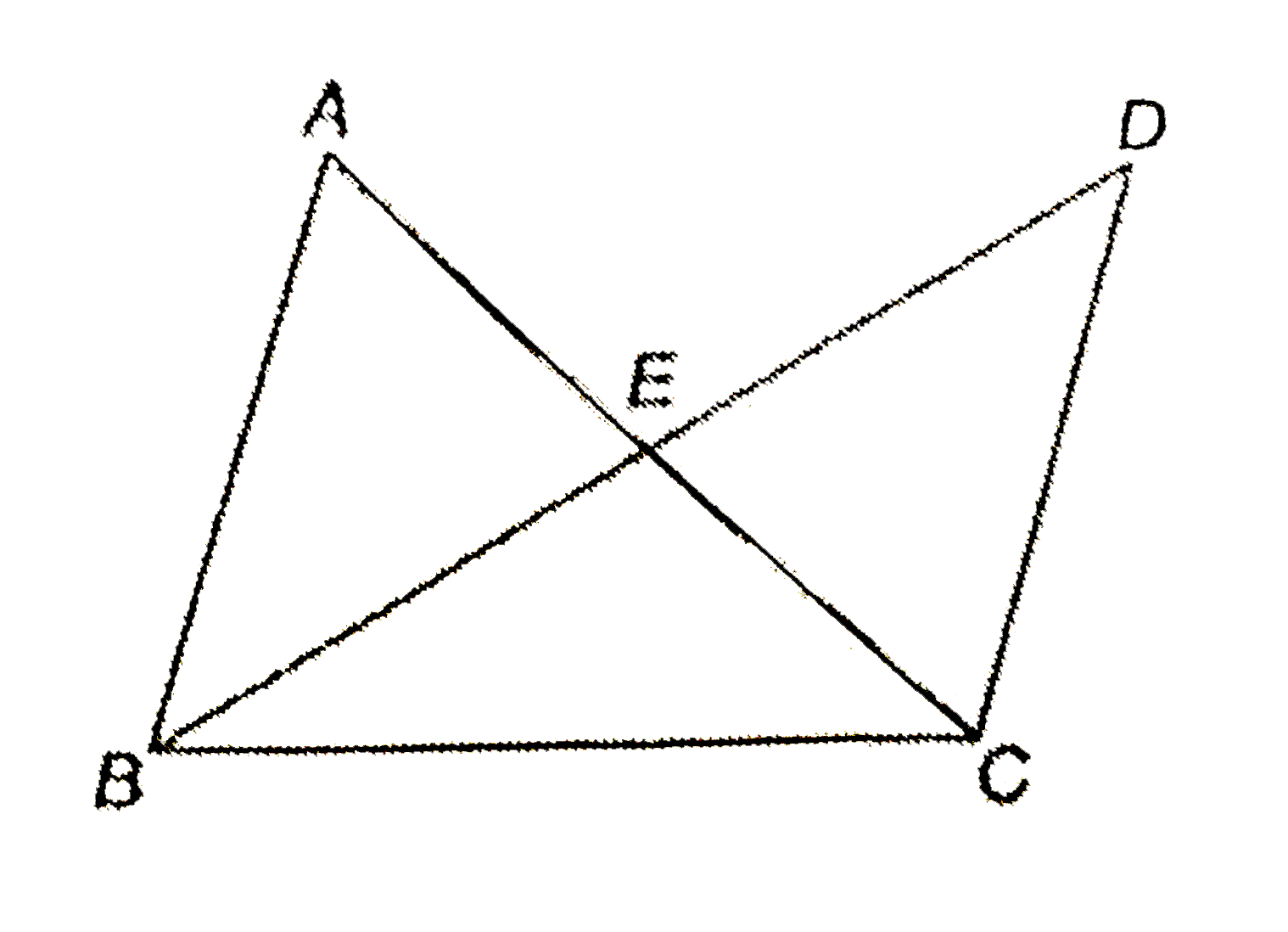
View SolutionIn the adjoining figure, ∠BAC=∠BDC and ∠ABC=∠BCD. Prove that :
(i) ΔABC≅ΔDCB
(ii) ΔABE≅ΔDCF.
View SolutionIn the given figure, AB∣∣CD and AB=CD, prove that:
 triangle AOB is congruent to triangle DOC.
triangle AOB is congruent to triangle DOC.
View Solution
