Question

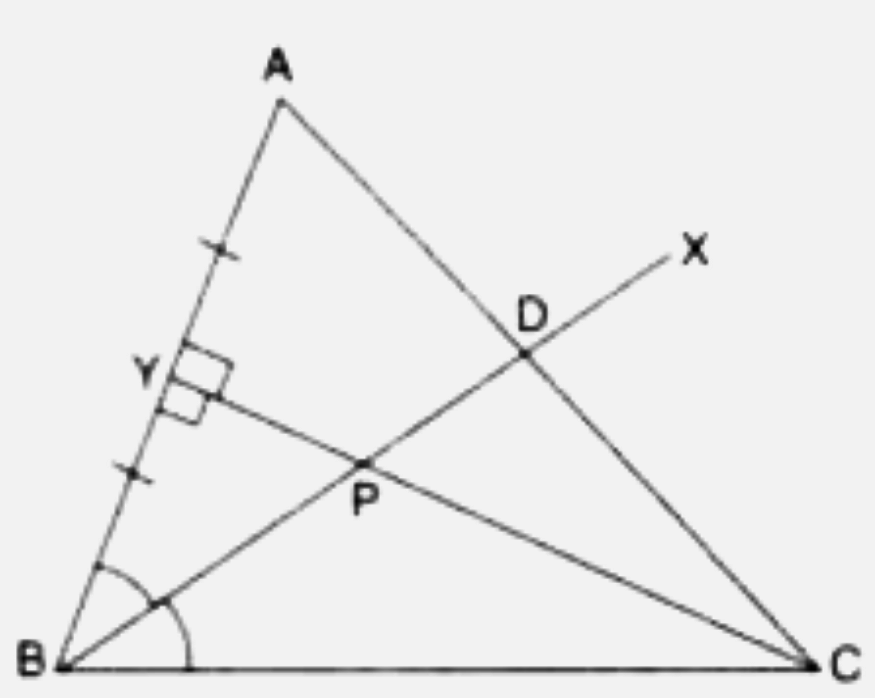
In the figure, BX bisects angle ABC and intersects AC at point D. Line segment CY is perpendicular to AB and intersects BX at point P. If Y is mid-point of AB, prove that : point P is equidistant from A and B.

Explanation

To prove that point P is equidistant from points A and B, we will use the properties of triangles and the concept of congruence.

1. Identify the Given Information:
- BX bisects angle ABC.
- CY is perpendicular to AB.
- Y is the midpoint of AB.
- P is the intersection of BX and CY.

2. Draw Right Triangles:
- Consider triangles PAY and PBY.
- Since CY is perpendicular to AB, angles PYA and PYB are both right angles (90 degrees).

3. Establish Common Sides:
- In triangles PAY and PBY, the segment PY is common to both triangles.

4. Use the Midpoint Property:
- Since Y is the midpoint of AB, we have AY = BY.

5. Apply the Criteria for Congruence:
- Now we have:
- Angle PYA = Angle PYB (both are 90 degrees)
- PY = PY (common side)
- AY = BY (since Y is the midpoint)
- By the criteria of congruence (RHS - Right angle, Hypotenuse, Side), we can conclude that triangle PAY is congruent to triangle PBY.

6. Conclude the Distances:
- Since the triangles are congruent, we can say that PA = PB.
- Therefore, point P is equidistant from points A and B.

Final Statement:
Thus, we have proved that point P is equidistant from points A and B.

---