Is ||gm ABCD a square?
I. Diagonals of ||gm ABCD are equal.
II. Diagonals of ||gm ABCD intersect at right angles.
if the question can be answered by one of the given statements alone and not by the other,
if the quesiton can be answered by either statement alone,
if the question can be answered by both the statements together but not by any one of the two,
if the question cannot be answered by using both the statements together.
The correct Answer is:C
When the diagonals of a ||gm are equal, it is either a rectangle or a square. Also, if the diagonals intersect at right angles then out of rectangle and square, it is a square.
∴ both I and II will give the answer.
Hence, the correct answer is (c ) .
Topper's Solved these Questions
- QUADRILATERALSBOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - MULTIPLE-CHOICE QUESTIONS (MCQ)
9 Videos
- QUADRILATERALSBOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - Short-Answer Questions
1 Videos
- QUADRILATERALSBOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - EXERCISE 10C
18 Videos
- PROBABILITYBOOK - RS AGGARWALCHAPTER - PROBABILITYEXERCISE - Multiple Choice Questions (Mcq)
16 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - MULTIPLE-CHOICE QUESTIONS (MCQ)
10 Videos
Similar Questions
Knowledge Check
The area of ||gm ABCD is
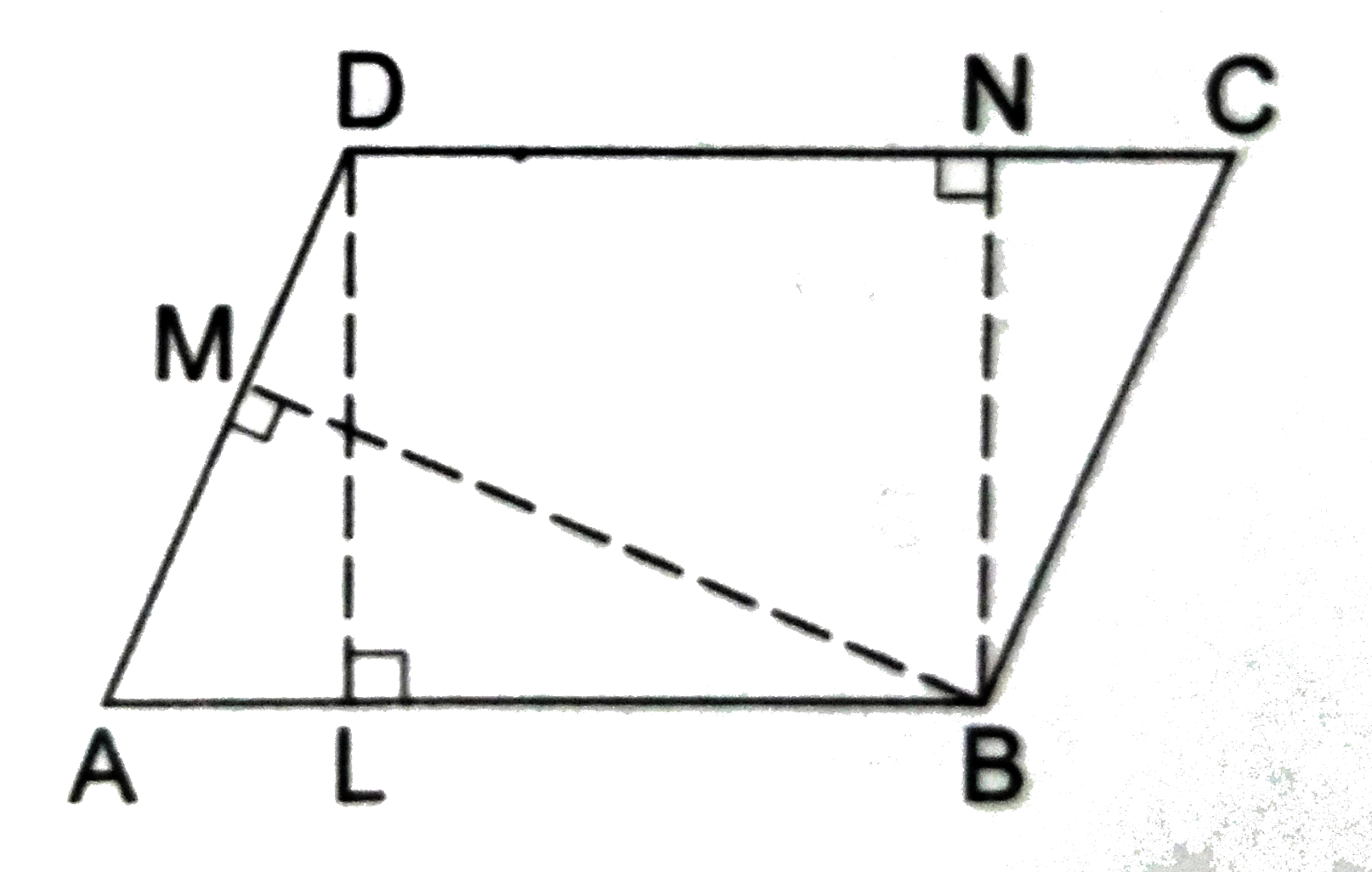 AAB×BMBBC×BNCDC×DLDAD×DL
AAB×BMBBC×BNCDC×DLDAD×DLIn a quadrilateral ABCD, with unequal sides if the diagonals AC and BD intersect at right angles, then
AAB2+BC2=CD2+DA2BAB2+CD2=BC2+DA2CAB2+AD2=BC2+CD2DAB2+BC2=2(CD2+DA2)In a quadrilateral ABCD, with unequal sides if the diagonals AC and BD intersect at right angles, then
AAB2+BC2=CD2+DA2BAB2+CD2=BC2+DA2CAB2+AD2=BC2+CD2DAB2+BC2=2(CD2+DA2)
If the diagonals of a parallelogram are equal and intersect at right angles, then the parallelogram is a square.
View SolutionIf ACandBD are diagonals of a parellelogram ABCD, show that
View SolutionThe diagonals of quad. ABCD cut at right angle then find AB2+CD2 = ?
View SolutionIs quadrilateral ABCD a ||gm?
I. Diagonals AC and BD bisect each other.
II. Diagonals AC and BD are equal.
View SolutionAssertion : ABCD is a square. AC and BD intersect at O. The measure of ∠AOB=90∘
Reason : Diagonals of a square bisect each other at right angles.
View Solution

