Josephine purchase a computer for $4,590. the computer decrease in value at a constant rate for 9 years, after which it is considered not to have any monetary value. How much is the computer worth 6 years after it is purchased?
1530
2295
3060
4080
The correct Answer is:A
To find out how much Josephine's computer is worth 6 years after it was purchased, we can follow these steps:
Step 1: Determine the initial value of the computer
The initial value of the computer is given as:
Initial Value=4590 dollars
Step 2: Determine the final value of the computer after 9 years
According to the problem, the computer is considered to have no monetary value after 9 years. Therefore:
Final Value after 9 years=0 dollars
Step 3: Calculate the total depreciation over 9 years
The total depreciation (the amount the computer loses in value) is the difference between the initial value and the final value:
Total Depreciation=Initial Value−Final Value=4590−0=4590 dollars
Step 4: Calculate the annual depreciation rate
Since the depreciation occurs at a constant rate over 9 years, we can find the annual depreciation rate by dividing the total depreciation by the number of years:
Annual Depreciation Rate=Total DepreciationNumber of Years=45909=510 dollars per year
Step 5: Calculate the total depreciation after 6 years
To find out how much value the computer has lost after 6 years, we multiply the annual depreciation rate by the number of years:
Depreciation after 6 years=Annual Depreciation Rate×6=510×6=3060 dollars
Step 6: Calculate the worth of the computer after 6 years
Now, we can find the worth of the computer after 6 years by subtracting the depreciation after 6 years from the initial value:
Worth after 6 years=Initial Value−Depreciation after 6 years=4590−3060=1530 dollars
Final Answer
Thus, the worth of the computer 6 years after it is purchased is:
Worth of the computer=1530 dollars
---
The initial value of the computer is given as:
Initial Value=4590 dollars
According to the problem, the computer is considered to have no monetary value after 9 years. Therefore:
Final Value after 9 years=0 dollars
The total depreciation (the amount the computer loses in value) is the difference between the initial value and the final value:
Total Depreciation=Initial Value−Final Value=4590−0=4590 dollars
Since the depreciation occurs at a constant rate over 9 years, we can find the annual depreciation rate by dividing the total depreciation by the number of years:
Annual Depreciation Rate=Total DepreciationNumber of Years=45909=510 dollars per year
To find out how much value the computer has lost after 6 years, we multiply the annual depreciation rate by the number of years:
Depreciation after 6 years=Annual Depreciation Rate×6=510×6=3060 dollars
Now, we can find the worth of the computer after 6 years by subtracting the depreciation after 6 years from the initial value:
Worth after 6 years=Initial Value−Depreciation after 6 years=4590−3060=1530 dollars
Thus, the worth of the computer 6 years after it is purchased is:
Worth of the computer=1530 dollars
Topper's Solved these Questions
Similar Questions
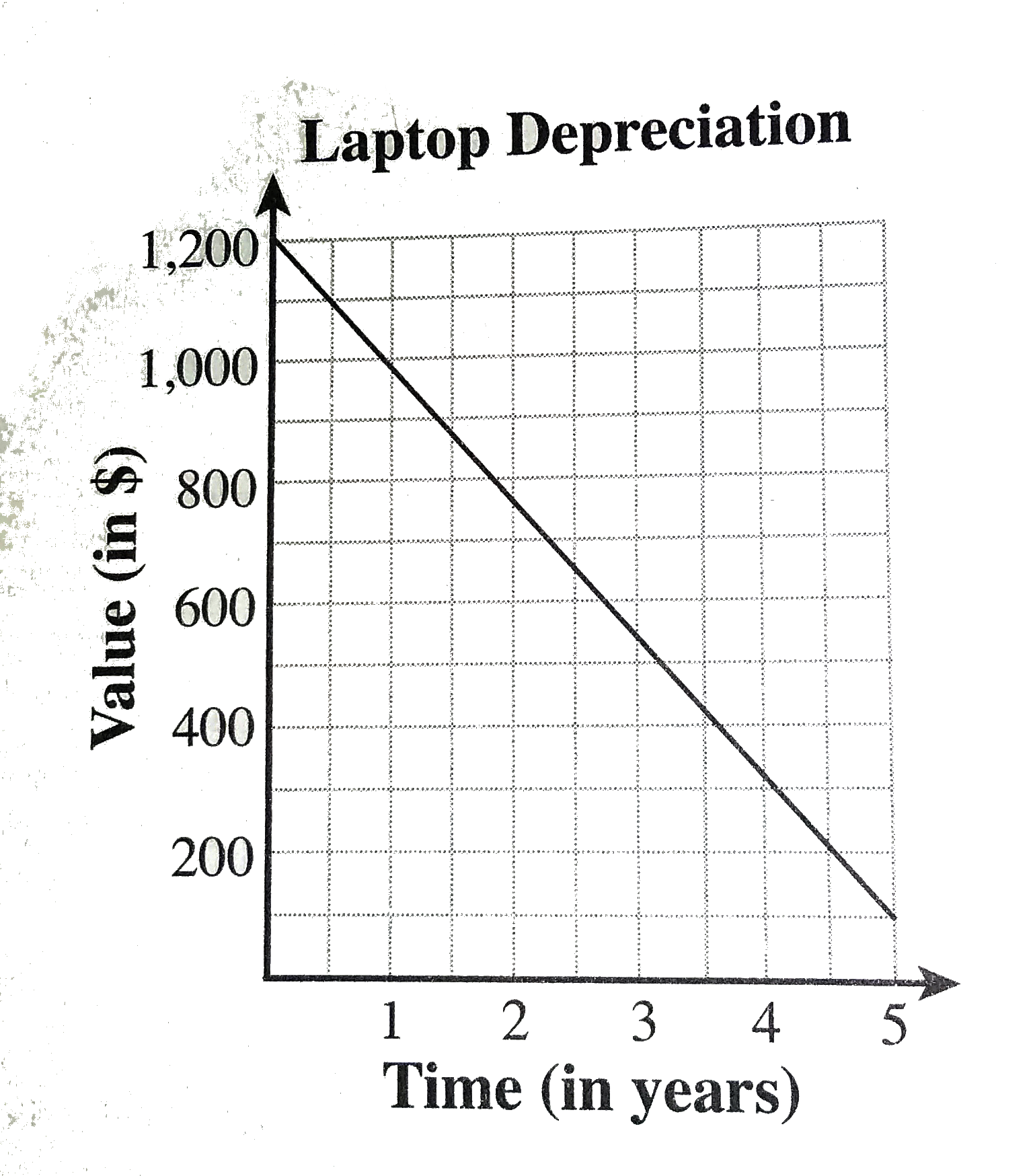
The figure above shows the straight-line depreciation of a laptop computer over the first five years of its use. According to the figure, what is the average rate of change in dollars per year of the value of the computer over the five-year period?
View SolutionMohan purchased a house for Rs 30000 and its value is depreciating at the rate of 25% per year. Find the value of the house after 3 years.
View Solution
Knowledge Check
A company that makes wildlife videos purchases camera equipment for $32,400. The equipment depreciates in value at a constant rate for 12 years, after which it is considered to have no monetary value. How much is the camera equipment worth 4 years after it is purchased?
A10800B16200C21600D29700An artist commissioned by a particular city displays an ice scultpture weighing 260 pounds. Once put on display, the scutpture melts at a constant rate for 20 days, at which point the sculpture will have completely melted. How much does the sculpture weigh, in pounds, 5 days after it is first displayed?
A247B195C130D65A company purchased a machine valued at $120,000. The value of the machine depreciates by the same amount each year so that after 10 years the value will be $30,000. Which of the following equations gives the value, v, of the machine, in dollars, t years after it was purchased for 0≤t≤10 ?
Av = 30,000 − 9,000tBv = 120, 000 − 9, 000tCv = 120,000 + 9,000tDv = 120,000 − 30,000t
A new color copier purchased for $8,500 is expected to depreciate(lose value) according to the equation y=−1,250x+8,500 where y is the value of the copier x years after it was purchased. The company that bought the copier plans to sell it when the value is $1,000 and upgrade to a new one. How many years after the copier is purchased will the company sell it?
View SolutionJuan purchased an antique that had a value of $200 at the time of purchase. Each year , the value of the antique is estimated to increase 10% over its value the previous year. The estimated value of the antique , in dollars, 2 years after purchase can be represented by the expression 200a , where a is a constant . What is the value of a ?
View SolutionThe value of a refrigerator which was purchased 2 years ago, depreciates at 12% per annum. If its present value is Rs 9680, for how much was it purchased?
View SolutionA car loses it value at a rate of 4.5% annually. If a car is purchased for $24,500, which equation can be used to determine the value of the car, V, after 5 years?
View SolutionA certain car costs $20,000. If the car loses 15 percent of its value each year, approximately how much will the car be worth after 5 years ?
View Solution
