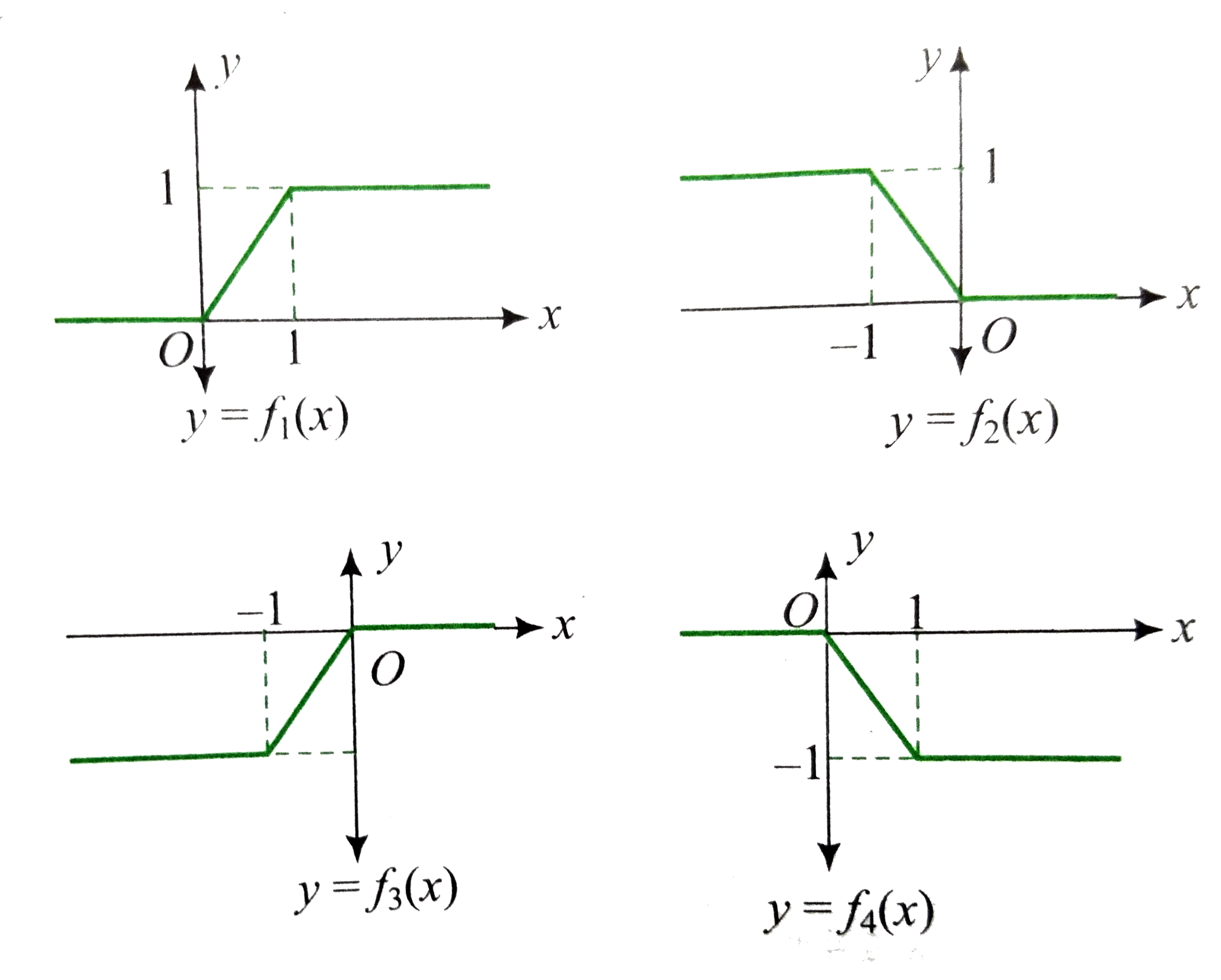
Let f1(x)={x,x≤x≤1and1x>1and0,otherwise f2(x)=f1(−x) for all x abd f3(x)=−f2(x) for all x and f4(x)=−f3(−x) for all x Which of the following is necessarily true?
A
f4(x)=f1(x) for all x
B
f1(x)=−f3(−x) for all x
C
f2(−x)=f4(x) for all x
D
f1(x)+f3(x)=0 for all x
Video Solution
Text Solution
The correct Answer is:B
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise (Multiple)
27 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise (Comprehension)
32 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.15
8 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank
31 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Knowledge Check
If f'(x)>f(x) for all x≥1andf(1)=0, then
Aexf(x) is a decreasing functionBe−xf(x) is an increasing functionCf(x)>0 for all x∈(1,∞)Df(x)<0 for ll x∈[1,∞)Let f(x) be defined for all x>0 and be continuous. Let f(x) satisfy f(x/y)=f(x)−f(y) for all x, y and f(e)=1. Then which of the following may be true
Af(x) is boundedBf(1/x)→0 as x→∞+Cxf(x)→1 as x→0+Df'(x)=1/xIf f(6-x)=f(x), for all x, then 15∫32x[f(x)+f(x+1)]dx is equal to :
A∫43f(x+2)dxB∫43f(x+1)dxC∫21f(x+1)dxD∫31f(x)dx
Let f(x+y)=f(x)+f(y) for all real x,yandf'(0) exists. Prove that f'(x)=f'(0) for all x∈Rand2f(x)=xf(2).
View SolutionIf f(1−x)=f(x) for all x,f′(1)=0andf′(0) exists, find its value.
View SolutionLet f(x)=x2+4x+1. Then (A) f(x)> 0 for all x (B) f(x)> 1 when x≥0 (C) f(x)≥1 when x≤−4 (D) f(x)=f(-x) for all x
View SolutionLet f(x)={1−|x|,|x|≤1and0,|x|<1andg(x)=f(x−)+f(x+1), for all x∈R.Then,the value of ∫3−3g(x)dx is
View SolutionLet g(x)=f(x)−2{f(x)}2+9{f(x)}3 for all x∈R Then
View Solution