Let f(x) be a polynomial of degree 5 such that f(|x|)=0 has 8 real distinct , Then number of real roots of f(x)=0 is ________.Solution in Tamil
The correct Answer is:5
Since f(|x|)=0 has 8 roots
⇒f(x)=0 has 4 real positive roots but since f(x) is polynomial of degree 5
⇒ fifth root is also real.
∴ Number of real roots of f(x)=0 is 5
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - JEE Previous Year12videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise (Matrix)9videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank28videos
Quadratic Equations, Inequalities, Modulus and Logarithms
- BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.311videos
SCALER TRIPLE PRODUCTS
Similar Questions
For real x, let f(x)=x3+5x+1 , then (1) f is oneone but not onto R (2) f is onto R but not oneone (3) f is oneone and onto R (4) f is neither oneone nor onto R
04:07View SolutionThe function f(x) is defined on the interval [0, 1]. Now, match the following lists:
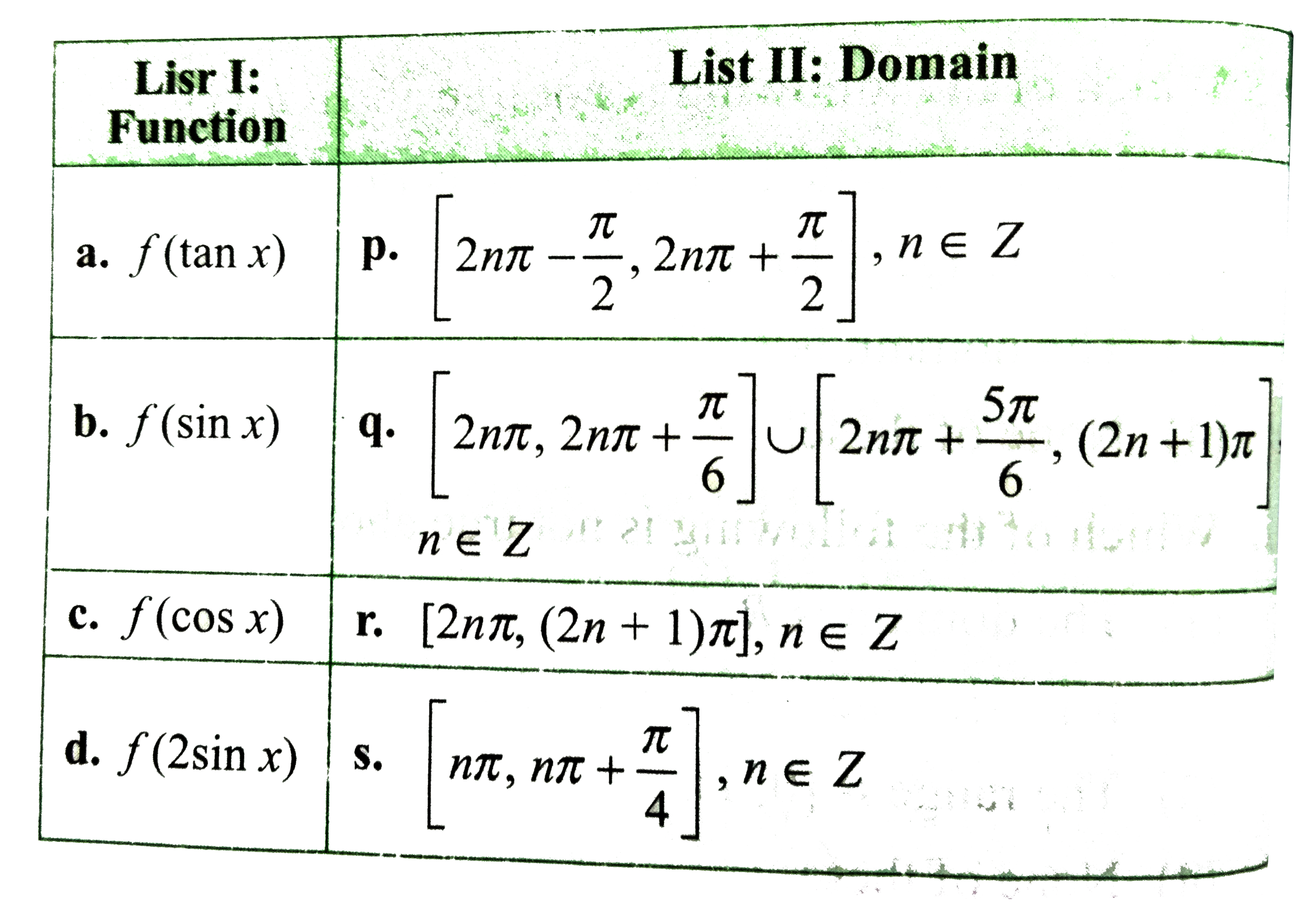 04:00View Solution
04:00View SolutionLet a,b,c,d be positive integers such that (log)ab=32and(log)cd=54. If (a−c)=9, then find the value of (b−d).
02:34View SolutionNumber of integral value(s) of λ for which vectors x2ˆi−ˆj+xˆk,(λ−1)ˆi−2λˆj+ˆk and ˆi−ˆj+ˆk, in the order from right-handed system ∀x ∈R, is
04:20View SolutionLet f(x) be a polynomial with real coefficients such that f(0)=1 and for all x ,f(x)f(2x^(2))=f(2x^(3)+x) The number of real roots of f(x) is:
01:38View SolutionLet f(x)=(e^(x))/(x^(2))x in R-{0}. If f(x)=k has two distinct real roots,then the range of k, is
03:17View SolutionIf f(x) is a polynomial of degree 5 with real coefficientssuch that f(|x|)=0 has 8 real roots,then the number of roots of f(x)=0
02:57View SolutionIf f(x)=x^(3)-3x+1, then the number of distinct real roots of the equation f(f(x))=0 is
View SolutionLet f(x)=ax^(3)+bx^(2)+cx+d,a!=0 If x_(1) and x_(2) are the real and distinct roots of f'(x)=0 then f(x)=0 will have three real and distinct roots if
03:54View SolutionIf f(x) is a polynomial of degree 5 with real coefficients such that f(|x|)=0 has 8 real roots, then f(x)=0 has
06:53View SolutionA polynomial of 6th degree f(x) satisfies f(x)=f(2−x),∀x∈R, if f(x)=0 has 4 distinct and 2 equal roots, then sum of the roots of f(x)=0 is
View SolutionIf f (x)= 0 be a polynomial whose coefficients are all and whose roots are all real, then degree of f (x) can be :
09:13View SolutionIf f(x) is a real valued polynomial and f (x) = 0 has real and distinct roots, show that the (f'(x)^(2)-f(x)f''(x))=0 can not have real roots.
05:56View SolutionLet f(x)=0 be a polynomial equation with real coefficients. Then between any two distinct real roots of f(x)=0, there exists at least one real root of the equation f'(x)=0. This result is a consequence of the celebrated Rolle's theorem applied to polynomials. Much information can be extracted about the roots of f(x)=0 from the roots of f'(x)=0.
The range of values of k for which the equation x4−14x2+24x−k=90 has four unequal real roots isView SolutionLet f(x)=0 be a polynomial equation with real coefficients. Then between any two distinct real roots of f(x)=0, there exists at least one real root of the equation f'(x)=0. This result is a consequence of the celebrated Rolle's theorem applied to polynomials. Much information can be extracted about the roots of f(x)=0 from the roots of f'(x)=0.
If the roots of x3−12x+k=0 lie in (−4,−3),(0,1) and (2, 3), then the range of values of k is03:56View SolutionLet f(x)=0 be a polynomial equation with real coefficients. Then between any two distinct real roots of f(x)=0, there exists at least one real root of the equation f'(x)=0. This result is a consequence of the celebrated Rolle's theorem applied to polynomials. Much information can be extracted about the roots of f(x)=0 from the roots of f'(x)=0.
If the three roots of x3−12x+k=0 lie in intervals (−4,−3),(0,1) and (2,3) ,then the exhaustive range of values of k is02:24View Solution

