Let p, q, r be three mutually perpendicular vectors of the same magnitude. If a vector x satisfies the equation p x ((x-q) x p) + q x ((x-r) x q) + r x ((x-p) x r)=0 Then x is given by :
The correct Answer is:x=a+b+c2
To solve the problem, we start with the equation given in the question:
p×x+(x−q)×p+q×(x−r)×q+r×(x−p)×r=0
Step 1: Rewrite the equation
We can rewrite the equation as follows:
p×x+(x×p−q×p)+(q×x−r×q)+(r×x−p×r)=0
Step 2: Combine like terms
Now, we can combine the terms involving x:
(p×x+x×p+q×x+x×q+r×x+x×r)−(q×p+r×q+p×r)=0
Step 3: Factor out x
Notice that p×x+x×p can be simplified, as can the other terms. Thus, we can factor out x:
(p+q+r)×x−(q×p+r×q+p×r)=0
Step 4: Solve for x
From the equation above, we can isolate x:
(p+q+r)×x=q×p+r×q+p×r
Step 5: Express x
Now, we can express x:
x=(q×p+r×q+p×r)(p+q+r)
Step 6: Recognize the vectors are mutually perpendicular
Since p, q, and r are mutually perpendicular vectors of the same magnitude, we can denote their magnitudes as k. Thus, we have:
|p|=|q|=|r|=k
Step 7: Final expression for x
Given that p, q, and r are mutually perpendicular, we can conclude that:
x=p+q+r
Final Answer
Thus, the vector x is given by:
x=p+q+r
---
We can rewrite the equation as follows:
Now, we can combine the terms involving x:
Notice that p×x+x×p can be simplified, as can the other terms. Thus, we can factor out x:
From the equation above, we can isolate x:
Now, we can express x:
Since p, q, and r are mutually perpendicular vectors of the same magnitude, we can denote their magnitudes as k. Thus, we have:
Given that p, q, and r are mutually perpendicular, we can conclude that:
Thus, the vector x is given by:
Topper's Solved these Questions
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)51videos
PRODUCT OF VECTORS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Single Integer Answer Type Questions)15videos
PRODUCT OF VECTORS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)54videos
PROBABILITY
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)21videos
PROPERTIES AND SOLUTION OF TRIANGLES
Similar Questions
Knowledge Check
- Question 1 - Select One
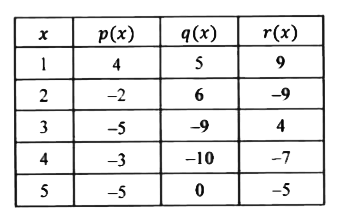
The table abvoe gives some value of the functions p, q, and r. At which value x does q(x)=p(x)+r(x)?A1B2C3D4
If p,q,r,s∈R, then equaton (x2+px+3q)(−x2+rx+q)(−x2+sx−2q)=0 has
View SolutionIf p,q,rands are real numbers such that pr=2(q+s), then show that at least one of the equations x2+px+q=0 and x2+rx+s=0 has real roots.
View Solution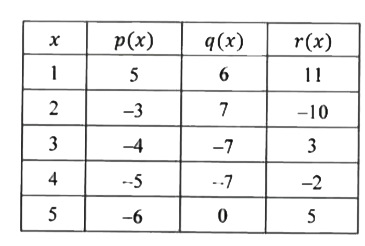
The table gives some values of the functions p, q and r. At which value of x does q(x)=p(x)+r(x)?View SolutionFor p, q∈R, the roots of (p2+2)x2+2x(p+q)−2=0 are
View SolutionProve that pxq−r+qxr−p+rxp−q>p+q+r,wherep,q,r are distinct and x≠1.
View SolutionIf P={x∈R:f(x)=0} and Q={x∈R:g(x)=0}, then P∪Q is
View SolutionLet p,q∈R. If 2−√3 is a root of the quadratic equation, x2+px+q=0, then
View Solution
