Let X is a continuous random variable with probability density function
f(x)={x6+k0≤x≤30 otherwise
The value of k is equal to
112
13
14
16
The correct Answer is:A
To find the value of k in the given probability density function f(x)=x6+k for 0≤x≤3 and f(x)=0 otherwise, we need to ensure that the total area under the probability density function equals 1. This is achieved by integrating the function over its defined interval.
1. Set up the integral of the probability density function:
∫30f(x)dx=1
Substitute f(x):
∫30(x6+k)dx=1
2. Split the integral:
∫30(x6)dx+∫30kdx=1
3. Calculate the first integral:
∫30x6dx=16∫30xdx
The integral of x is:
∫xdx=x22
Evaluating from 0 to 3:
16(322−022)=16(92)=912=34
4. Calculate the second integral:
∫30kdx=k⋅∫301dx=k⋅(x)30=k⋅(3−0)=3k
5. Combine the results:
34+3k=1
6. Solve for k:
3k=1−34=14
k=14⋅13=112
Final Answer:
The value of k is 112.
---
∫30f(x)dx=1
Substitute f(x):
∫30(x6+k)dx=1
∫30(x6)dx+∫30kdx=1
∫30x6dx=16∫30xdx
The integral of x is:
∫xdx=x22
Evaluating from 0 to 3:
16(322−022)=16(92)=912=34
∫30kdx=k⋅∫301dx=k⋅(x)30=k⋅(3−0)=3k
34+3k=1
3k=1−34=14
k=14⋅13=112
The value of k is 112.
Topper's Solved these Questions
- PROBABILITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PROBABILITYEXERCISE - MHT CET Corner
4 Videos
- PROBABILITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PROBABILITYEXERCISE - Exercise 1 (TOPICAL PROBLEMS)
44 Videos
- PRACTICE SET 24BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PRACTICE SET 24EXERCISE - Paper 2 (Mathmatics)
50 Videos
- SEQUENCES AND SERIES BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SEQUENCES AND SERIES EXERCISE - EXERCISE 31
1 Videos
Similar Questions
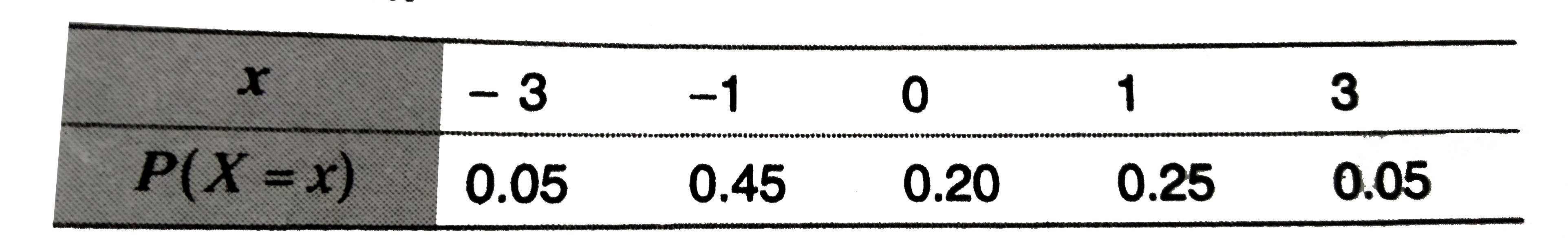
The random variable X has the following probability distribution
Then, F(0) is equal to
View SolutionIf a random variable waiting time in minutes for bus and probability density function of x is given by
f(x)={15,0≤x≤50,otherwise
Then probability of waiting time not more than 4 minutes is equal to
View Solution
Knowledge Check
X is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
A0.11724B0.11224C0.11336D0.11236If a curve X has probability density function (pdf)
f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩ax,0≤x≤1a,1≤x≤23a−ax,2≤x≤30,otherewise
Then, a is equal toA1B13C12D15If a curve X has probability density function (pdf)
f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩ax,0≤x≤1a,1≤x≤23a−ax,2≤x≤30,otherewise
Then, a is equal toA1B13C12D15
If X is the a random variable with probability mass function P(x)=kx, for x = 1,2,3
= 0 , otherwise
then k = ……..
View SolutionThe pdf of a discrete random variable is defined as f(x)={kx2,0≤x≤60, elsewhere
Then the value of F(4) is
View SolutionIf the p.d.f of a random variable X is
F(x)={0.5x0≤x≤20otherwise
Then the value of P(0.5≤X≤1.5) is
View SolutionIf the probability density function of a continuous random variable X is f(x)={3+2x182≤x≤40x<2 orx>4 Then the mathematical expectation of X is
View SolutionIf the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
View Solution
