Look at the adjoining figure. Show that length AH gt sum of lengths of AB + BC + CD
Text Solution
From the given figure , we have
AB+ BC + CD = AD [AB, BC and CD are the parts of AD] Here, AD is also the parts of AH.
According to Euclid's axiom , the whole is greater than the part. i.e., AH>AD.
So, length AH> sum of lengths of AB + BC + CD.
|
Topper's Solved these Questions
- INTRODUCTION TO EUCLID GEOMETRYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO EUCLID GEOMETRYEXERCISE - Exercise 5.4 Long Answer Type Questions
5 Videos
- INTRODUCTION TO EUCLID GEOMETRYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO EUCLID GEOMETRYEXERCISE - Exercise 5.2 Very Short Answer Type Questions
9 Videos
- HERON'S FORMULABOOK - NCERT EXEMPLAR ENGLISHCHAPTER - HERON'S FORMULAEXERCISE - Long Answer Type Questions
8 Videos
- LINEAR EQUATION IN TWO VARIABLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR EQUATION IN TWO VARIABLESEXERCISE - Exercise 4.8
1 Videos
Similar Questions
Knowledge Check
In the adjoining figure AB∣∣CDandPQ=SR, then :
 APQ=PSBSR=RPCPS=QRDAP=RD
APQ=PSBSR=RPCPS=QRDAP=RD
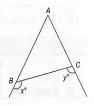
In the adjoining figure, x>y, Prove that AB>AC
View SolutionFrom the following figure, prove that : AB>CD
View SolutionIn given figure, show that AB || CD
View SolutionIn the adjoining figure AB||CD, find x and y

View SolutionIn the adjoining figure AB||CD, find x and y

View SolutionIn the adjoining figure AE||CD and BC||ED, then find Y.
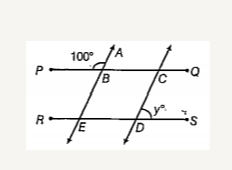
View SolutionIn the given figure, AB || DE. Find the length of CD.
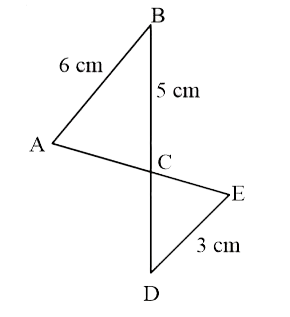
View Solution