Prove that any three points on a circle cannot be collinear.Solution in Marathi
The correct Answer is:∴ Points A,B and C are non collinear points.
Step by step video & image solution for Prove that any three points on a circle cannot be collinear. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLEBOOK - TARGET PUBLICATIONCHAPTER - CIRCLEEXERCISE - Activities for Practice
3 Videos
- CIRCLEBOOK - TARGET PUBLICATIONCHAPTER - CIRCLEEXERCISE - Multiple Choice Question
27 Videos
- CIRCLEBOOK - TARGET PUBLICATIONCHAPTER - CIRCLEEXERCISE - Practice Set 3.5
3 Videos
- CHALLENGING QUESTIONS BOOK - TARGET PUBLICATIONCHAPTER - CHALLENGING QUESTIONS EXERCISE - CHAPTER-1 :Linear Equations in Two Variable
1 Videos
- CO-ORDINATE GEOMETRYBOOK - TARGET PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - CHAPTER ASSESSMENT
15 Videos
Similar Questions
Complete the activity by filling the boxes.
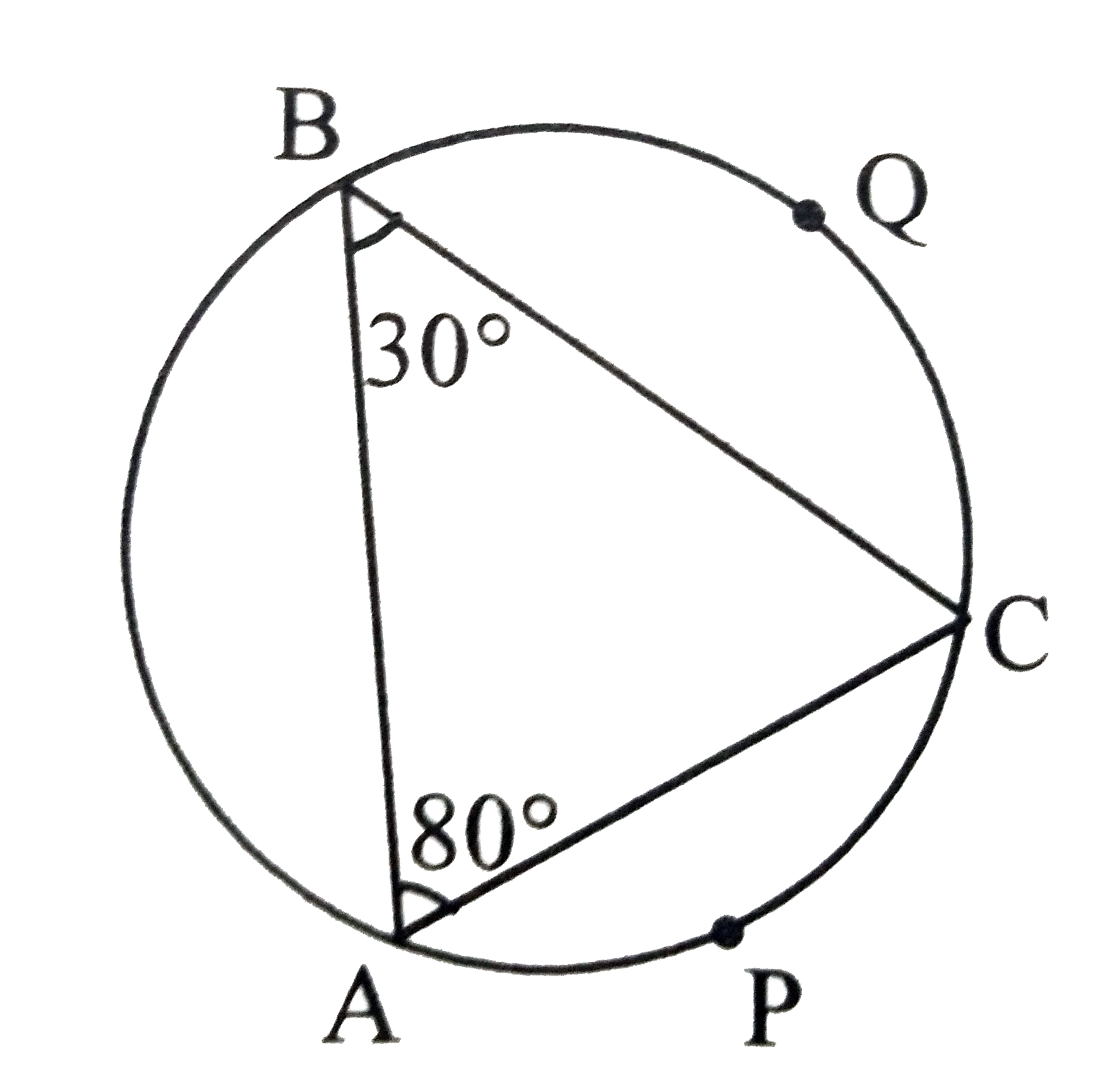
In the given figure , ∠BAC=80∘ ,∠ABC=30∘ and
m(arcBQC)=160∘.
Find (i) m(arcAPC) (ii) m(arcAB).
View SolutionConcentric Circles have the same______.
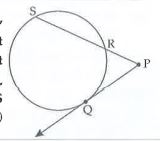
View SolutionIn the adjoining figure, point Q is the point of contact of tangent and the circle. If PQ=12, PR=8, then find PS and RS.
View SolutionSum of two numbers is 97. If the greater number is divided by the the smaller, the quotient is 7 and the remainder is 1. Find the numbers.
View SolutionChoose the correct alternative.
(i) The distance of Q (3,-1) from the origin is
View SolutionBy using the concept of equation of a line, prove that the three points (3,0), (−2,−2)and (8,2)are collinear.
View SolutionThere is one and only circle passing through three non-collinear points.
View SolutionThrough three collinear points a circle can be draw.
View SolutionProve the any three points on a circle cannot be collinear .
View SolutionOnly one circle can be throught three collinear points .
View Solutionतीन असंरेखीय (Non-collinear) बिन्दुओ से गुजरने वाले वृतो की कुल संख्या कितनी है :
View SolutionProve that any three points on a circle cannot be collinear.
View SolutionThe number of circles that can be drawn from three non-collinear points is
View SolutionProve that, through three given points which are not collinear, there is only one circle.
View SolutionThrough three collinear points a circle can be draw.
View SolutionThere is one and only one circle passing through three non-collinear points.
View Solution
