Prove that :
(i) A⊆B,B⊆C⇒A⊆C
(ii) A⊆ϕ,⇔A=ϕ
Step by step video & image solution for Prove that : (i) A sube B,B sube C rArrA sube C (ii) A sube phi, hArr A = phi by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SETSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SETSEXERCISE - Exercise 1D
28 Videos
- SETSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SETSEXERCISE - Exercise 1E
15 Videos
- SETSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SETSEXERCISE - Exercise 1B
10 Videos
- SEQUENCE AND SERIESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SEQUENCE AND SERIESEXERCISE - Miscellaneous Exercise
32 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - MISCELLANEOUS EXERCISE
7 Videos
Similar Questions
Given, A = {Triangles}, B = {Isosceles triangles}, C = {Equilateral triangles}. State whether the following are true or false. Give reasons.
(i) A⊂B, (ii) B⊆A, (iii) C⊆B, (iv) B⊂A, (v) C⊂A, (vi) C⊆B⊆A
View SolutionProve that |b+caa<br>bc+abcca+b∣
View SolutionGiven, A = {Quadrilaterals}, B = {Rectangles}, C = {Squares}, D = {Rhombuses}. State, giving reasons, whether the following are true or false.
(i) B⊂C, (ii) D⊂B, (iii) C⊆B⊆A, (iv) D⊂A, (v) B⊇C, (iv) A⊇B⊇C
View SolutionLet M={letters of the word REAL} and N={letters of the word LARE}. Write sets M and N in roster form and then state whether:
(i) M⊆N is true.
(ii) N⊆M is true.
(iii) M=C is true.
View SolutionIf A⊆BandC⊆D, then prove that:
A×C⊆B×D
View SolutionLet A, B, C and D be four non-empty sets. The contrapositive statement of ''If A⊆B and B⊆D, then A⊆C'' is :
View SolutionIf A⊆BandB⊂X, the the correct option is :
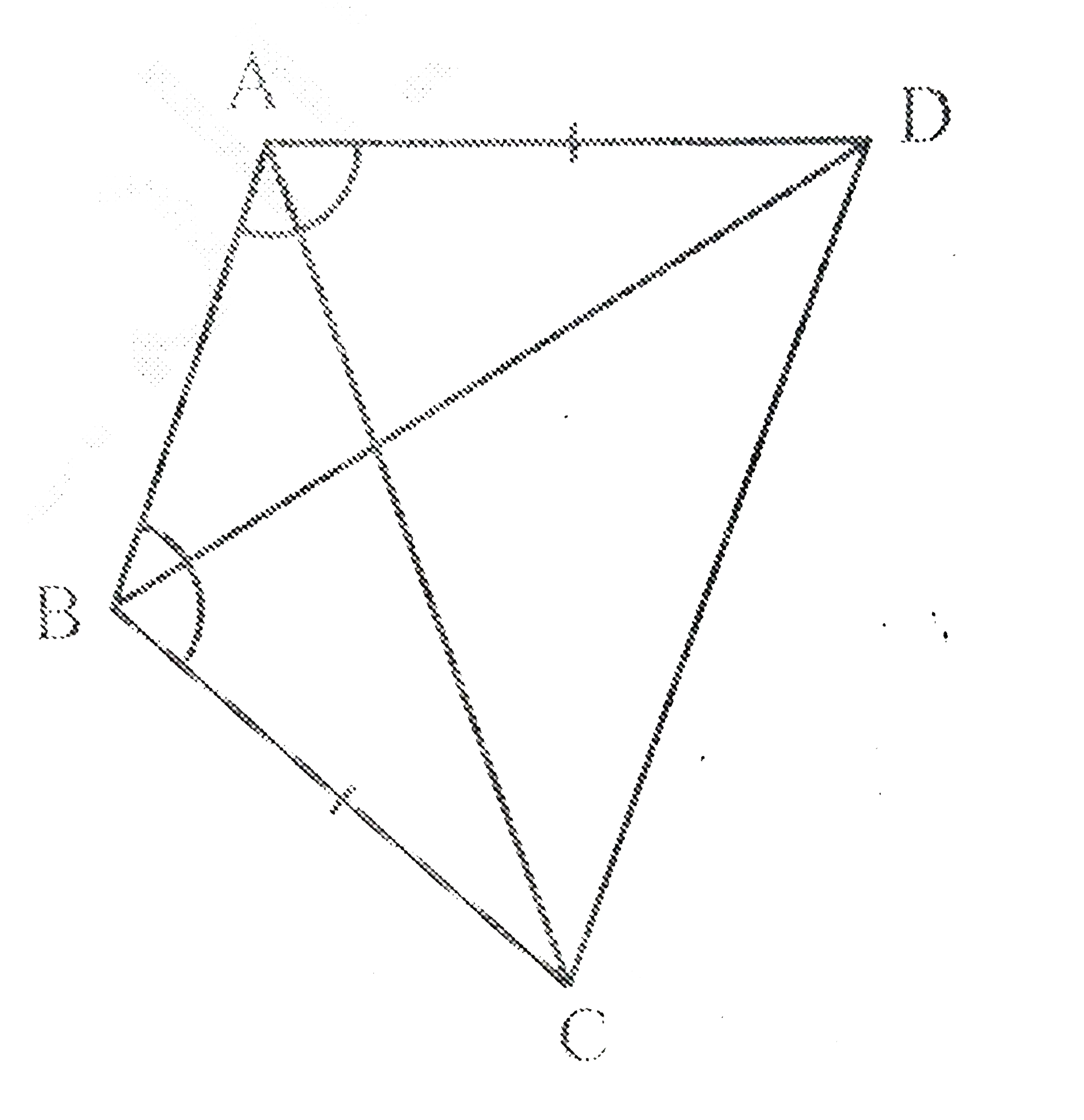
View SolutionABCD is a quadrilateral in which and∠DAB =∠CBA(see Fig. 7.17). Prove that
(i) ΔABD≅ΔBAC
(ii) BD = AC
(iii) ∠ABD =∠BAC
View SolutionDraw a Venn-diagram to show the relationship between sets A and B , such that A⊆B. Now shade the region representing :
(i) A∪B, (ii) B'∩A, (iii) A∩B, (iv) (A∪B)'
View SolutionIf A and B are two sets such that A⊆B, then find
(i) A∪B
(ii) A∩B.
View Solution
