Prove that n−1∑k=1(n−k)cos(2kπ)n=−n2, wheren≥3 is an integer
Video Solution
Text Solution
Let S=n−1∑k=1(n−k)cos(2kπn)
⇒S=(n−1)cos(2πn)+(n−2)cos(2⋅2πn)+...+1⋅cos((n−1)⋅2πn)→(1)
Now, we know, cosθ=cos(2π−θ)
So, replacing 2πn with 2π−2πn in the above equation, we get,
⇒S=(n−1)cos(n−1)(2πn)+(n−2)cos(n−1)(2πn)+...+cos(2πn)→(2)
Now, adding (1) and (2),
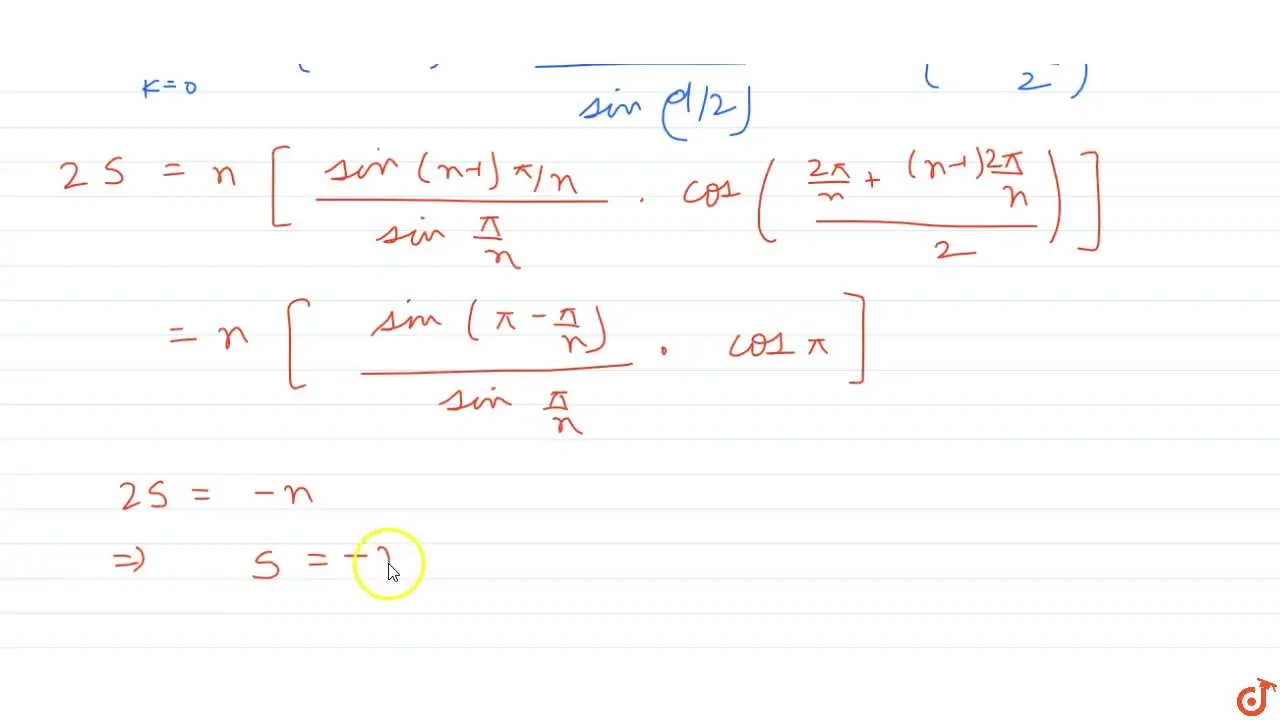
2S=n[cos(2πn)+cos(2⋅2πn)+...+cos(n−1)⋅2πn]
⇒2S=nn−2∑k=0cos((2πn)+k2πn))
Now, n−1∑k=0cos(a+kd)=sin(nd2)sin(d2)⋅cos(a+nd2)
So, using the above formula in our equation
⇒2S=n⎡⎢
⎢⎣sin(n−1)(πn)sin(πn)⋅cos⎛⎜
⎜⎝(2πn)+(n−1)2πn2⎞⎟
⎟⎠⎤⎥
⎥⎦
⇒2S=n[sin(π−πn)sinπ/n⋅cosπ]
⇒2S=−n
⇒S=−n2
∴n−1∑k=1(n−k)cos(2kπn)=−n2.
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Prove that n−1∑k=1(n−k)cos(2kπ)n=−n2, wheren≥3 is an integer
View SolutionProve that n∑k=1k.kPn+1−1.
View SolutionProve that k∑r=1(−3)r−1^(3n)C2r−1=0,wherek=3n/2 andn is an even integer.
View SolutionProve that k∑r=1(−3)r−1 3nC2r−1=0, where k=(3n)/2 and n is an even integer
View Solutionn∑k=1k(1+1n)k−1 =
View Solutionprove that n∑k=1k2−k=2[1−2−n−n.2−(n+1))
View SolutionProve thatk∑r=1(−3)r−1C(3n,2r−1)=0, where k=3n2 and n is an even positive integer.
View SolutionIf n be integer gt1, then prove that n−1∑r=1cos(2rπ)n=−1
View SolutionLet an=n∑k=11k(n+1−k), then for n≥2
View SolutionProve that n∑k=0^nCksinKx.cos(n−K)x=2n−1sinnx
View Solution