Question

Prove that the line segment joining the mid-points of the diagonals of
a trapezium is parallel to each of the parallel sides and is equal to half
the difference of these sides.

Explanation

Given Let ABCD be a trapezium in which AB||DC and let M and N be the mid-points of the diagonals AC and BD, respectively.

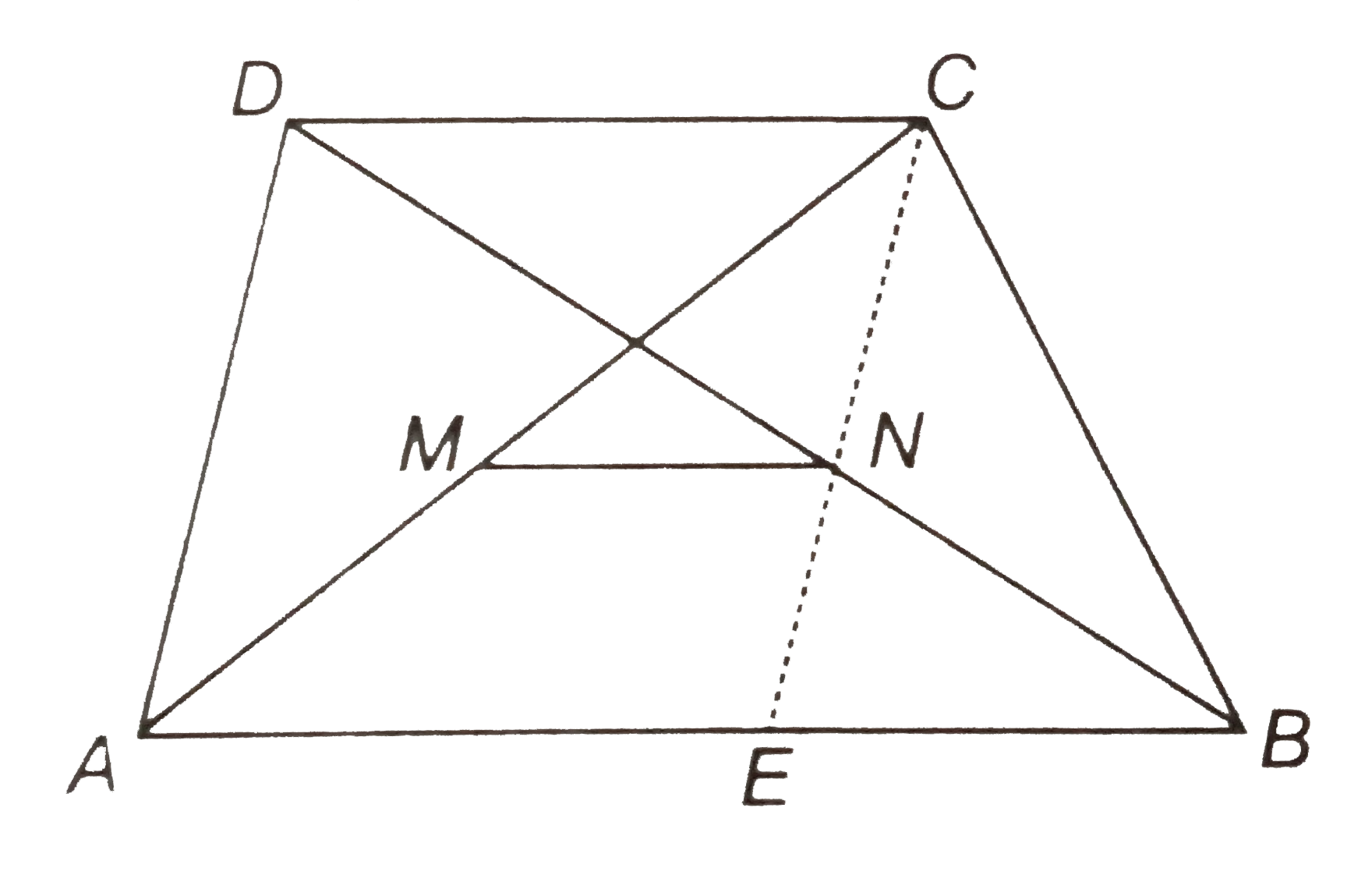
To prove MN||AB||CD
Construction Join CN and produce it to meet AB at E.
In `Delta`CDN and `Delta`EBN, we have
`" "DN=BN" "`[since, N is the mid-point of BD]
`" "angleDCN=angleBEN" "` [alternate interior angles]
and `" "angleCDN=angleEBN" "`[alternate interior angles]
`therefore" "DeltaCDN~=DeltaEBN" "`[by AAS congruence rule ]
`therefore" "DC=EB and CN =NE" "` [by CPCT rule]
Thus, in `Delta`CAE, the points M and N are the mid-points of AC and CE, respectively.
`therefore" "MN||AE" "`[by mid-point theorem]
`rArr" "MN||AB||CD" "` Hence proved.