Prove that the volume of the largest cone, that can be inscribed in a sphere of radius $R .$ is $\frac{8}{27}$ of the volume of the sphere.

Video Solution

Text Solution

Verified by Experts

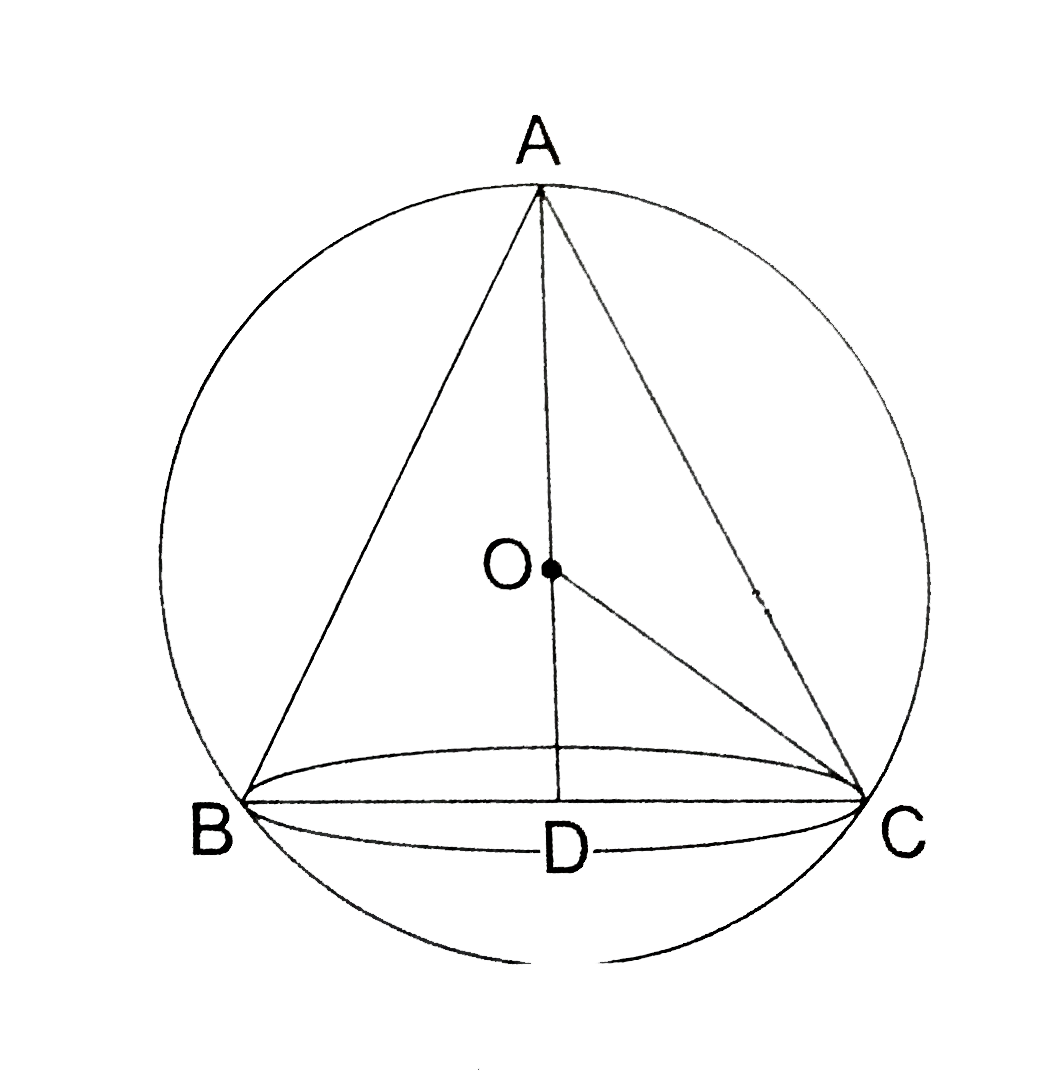
Let the radius of the given sphere be a. Let V be the volume of the inscribed cone, h be its height and r be its radius
Then, $V = \frac{1}{3} π r^{2} h$
Now, $O D = (A D - O A) = (h - a)$
$O C^{2} = O D^{2} + D C^{2} \Rightarrow a^{2} = {(h - a)}^{2} + r^{2}$
$\Rightarrow r^{2} = h (2 a - h)$
$∴ V = \frac{1}{3} π h^{2} (2 a - h) = [\frac{2 π a h^{2}}{3} - \frac{1}{3} π h^{3}]$
$∴ \frac{d V}{d h} = (\frac{4 π a h}{3} - π h^{2}) and \frac{d^{2} V}{d h^{2}} = (\frac{4 π a}{3} - 2 π h)$
So, $\frac{d V}{d h} = 0 \Rightarrow h = \frac{4 a}{3} and [\frac{d^{2} V}{d h^{2}} at h = \frac{4 a}{3}] = - \frac{4 π a}{3} < 0$
$∴$ V is maximum when $h = \frac{4 a}{3} and r^{2} = \frac{8 a^{2}}{9}$
Maximum volume $= \frac{1}{3} π \times \frac{8 a^{2}}{9} \times \frac{4 a}{3} = \frac{8}{27} \times (\frac{4}{3} π a^{3})$

Updated on:21/07/2023