Resolve 2x−3(x−1)(x2+1)2 into partial fractions,
Video Solution
Text Solution
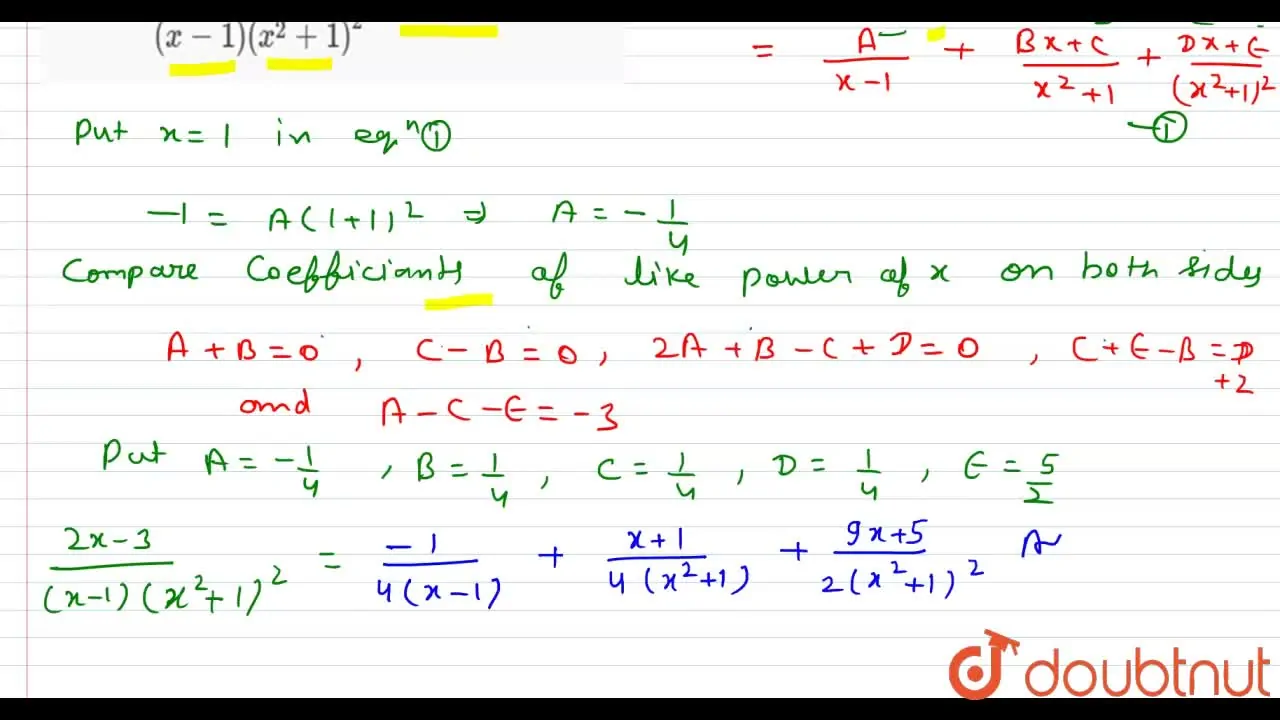
Let 2x−3(x−1)(x2+1)2=Ax−1+Bx+Cx2+1+Dx+E(x2+1)2. Then, 2x−3=A(x2+1)2+(Bx+C)(x−1)(x2+1)+(Dx+E)(x−1)…………(i)
Putting x=1 in eq (i) , we get −1=A(1+1)2⇒A=−
Comparing coefficients of like powers of x on both side of (i), we have
A+B=0,C−B=0,2A+B−C+D=0,C+E−B−D=2 and A−C−E=−3.
Putting A=−14 and solving these equations, we get
B=14=C,D=14 and E=52∴2x−3(x−1)(x2+1)2=−14(x−1)+x+14(x2+1)+9x+52(x2+1)2
|
Updated on:21/07/2023
Related Playlists
- BOOK - RESONANCECHAPTER - INDEFINITE INTEGRATION EXERCISE - EXERCISE -1 SUBJECTIVE QUESTIONS1videos
INDEFINITE INTEGRATION
- BOOK - RESONANCECHAPTER - INDEFINITE INTEGRATION EXERCISE - SUBJECTIVE QUESTIONS14videos
INDEFINITE INTEGRATION
- BOOK - RESONANCECHAPTER - GEOMETRYEXERCISE - Exercise-1 (Part-I: Previous Asked Question For Pre RMO)50videos
GEOMETRY
- BOOK - RESONANCECHAPTER - MATRICES & DETERMINANTEXERCISE - HLP33videos
MATRICES & DETERMINANT
Similar Questions
Resolve 2x+1(x+3)(x2+1)2 into partial fractions.
View SolutionResolve 3x−2(x−1)2(x+1)(x+2) into partial fractions.
View SolutionResolve x(x−2)(x2+3)2 into partial fractions.
View SolutionResolve 2x+1(x−1)(x2+1) into partial fractions.
View SolutionResolve 2x−1(x+1)(x2+2) into partial fractions.
View SolutionResolve 2x−5(x+2)(x2−x+5) into partial fractions.
View Solution2x+1(x+1)(x−2) into partial fractions
View SolutionResolve 3x2+2x+4(x−1)(x2−4) into partical fractions.
View Solution" " Resolve "(1)/((x-1)^(2)(x-2))" into partial fractions."
View SolutionResolve 2x+7(x+1)(x2+4) into partial fractions.
View Solution