Show that in general there are three values of t for which the following system of equations has a non- trival solution (a-t)x+by+cz=0, bx+(c-t)y+az=0 and cx+ay+(b-t)z=0.
Express the product of these values of t in the form of a determinant.
The correct Answer is:∣∣ ∣∣abcbcacab∣∣ ∣∣
To solve the problem, we need to show that the system of equations has three values of t for which it has a non-trivial solution and express the product of these values in the form of a determinant.
1. Write the System of Equations in Matrix Form:
The given system of equations is:
(a−t)x+by+cz=0
bx+(c−t)y+az=0
cx+ay+(b−t)z=0
We can express this system in matrix form as:
⎡⎢⎣a−tbcbc−tacab−t⎤⎥⎦⎡⎢⎣xyz⎤⎥⎦=⎡⎢⎣000⎤⎥⎦
2. Condition for Non-Trivial Solutions:
For the system to have a non-trivial solution (i.e., not all x,y,z are zero), the determinant of the coefficient matrix must be zero:
det⎡⎢⎣a−tbcbc−tacab−t⎤⎥⎦=0
3. Calculate the Determinant:
We need to compute the determinant:
D(t)=det⎡⎢⎣a−tbcbc−tacab−t⎤⎥⎦
Using the determinant formula for a 3×3 matrix, we can expand this determinant.
4. Expand the Determinant:
Expanding the determinant, we have:
D(t)=(a−t)((c−t)(b−t)−a2)−b(b(c−t)−ac)+c(b(a−t)−bc)
This will yield a polynomial in t of degree 3.
5. Form of the Cubic Equation:
The determinant D(t) can be expressed in the form:
D(t)=αt3+βt2+γt+δ
where α,β,γ,δ are constants dependent on a,b,c.
6. Roots of the Cubic Equation:
The cubic equation will have three roots (values of t). The product of the roots of a cubic equation at3+bt2+ct+d=0 is given by:
Product of roots=−da
In our case, we need to express this product in terms of the determinant.
7. Substituting t=0:
To find δ (the constant term), we substitute t=0 into the determinant:
D(0)=det⎡⎢⎣abcbcacab⎤⎥⎦
8. Final Expression:
Therefore, the product of the values of t for which the system has a non-trivial solution can be expressed as:
Product of roots=−D(0)α
where D(0) is the determinant calculated above.
The given system of equations is:
(a−t)x+by+cz=0
bx+(c−t)y+az=0
cx+ay+(b−t)z=0
We can express this system in matrix form as:
⎡⎢⎣a−tbcbc−tacab−t⎤⎥⎦⎡⎢⎣xyz⎤⎥⎦=⎡⎢⎣000⎤⎥⎦
For the system to have a non-trivial solution (i.e., not all x,y,z are zero), the determinant of the coefficient matrix must be zero:
det⎡⎢⎣a−tbcbc−tacab−t⎤⎥⎦=0
We need to compute the determinant:
D(t)=det⎡⎢⎣a−tbcbc−tacab−t⎤⎥⎦
Using the determinant formula for a 3×3 matrix, we can expand this determinant.
Expanding the determinant, we have:
D(t)=(a−t)((c−t)(b−t)−a2)−b(b(c−t)−ac)+c(b(a−t)−bc)
This will yield a polynomial in t of degree 3.
The determinant D(t) can be expressed in the form:
D(t)=αt3+βt2+γt+δ
where α,β,γ,δ are constants dependent on a,b,c.
The cubic equation will have three roots (values of t). The product of the roots of a cubic equation at3+bt2+ct+d=0 is given by:
Product of roots=−da
In our case, we need to express this product in terms of the determinant.
To find δ (the constant term), we substitute t=0 into the determinant:
D(0)=det⎡⎢⎣abcbcacab⎤⎥⎦
Therefore, the product of the values of t for which the system has a non-trivial solution can be expressed as:
Product of roots=−D(0)α
where D(0) is the determinant calculated above.
Topper's Solved these Questions
- DETERMINANTS BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Determinants Exercise 7:
3 Videos
- DETERMINANTS BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
- DETERMINANTS BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Exercise (Statement I And Ii Type Questions)
7 Videos
- DEFINITE INTEGRALBOOK - ARIHANT MATHS ENGLISHCHAPTER - DEFINITE INTEGRALEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
- DIFFERENTIAL EQUATION BOOK - ARIHANT MATHS ENGLISHCHAPTER - DIFFERENTIAL EQUATION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
26 Videos
Similar Questions
if the system of equations
(a−t)x+by+cz=0
bx+(c−t)y+az=0
cx+ay+(b−t)z=0
has non-trivial solutions then product of all possible values of t is
View SolutionThe value of a for which the system of linear equations ax+y+z=0,ay+z=0,x+y+z=0 possesses non-trivial solution is
View SolutionDetermine the values of λ for which the following system of equations : λx+3y−z=1, x+2y+z=2, −λx+y+2z=−1 has non-trival solutions.
View SolutionFind a∈R for which the system of equations 2ax-2y+3z=0 , x+ay + 2z=0 and 2x+az=0 also have a non-trivial solution.
View SolutionThe greatest value of for which the system of linear equations
x−cy−cz=0
cx−y+cz=0
cx+cy−z=0
Has a non-trivial solution, is 1k. The value of k is _________.
View SolutionIf the planes x−cy−bz=0,cx−y+az=0andbx+ay−z=0 pass through a line, then the value of a2+b2+c2+2abc is
View SolutionIf the planes x−cy−bz=0,cx−y+az=0andbx+ay−z=0 pass through a line, then the value of a2+b2+c2+2abc is….
View SolutionConsider the linear equations ax+by+cz=0,bx+cy+az=0andcx+ay+bz=0.
Match the conditions/expressions in Column I with statements in Column II.
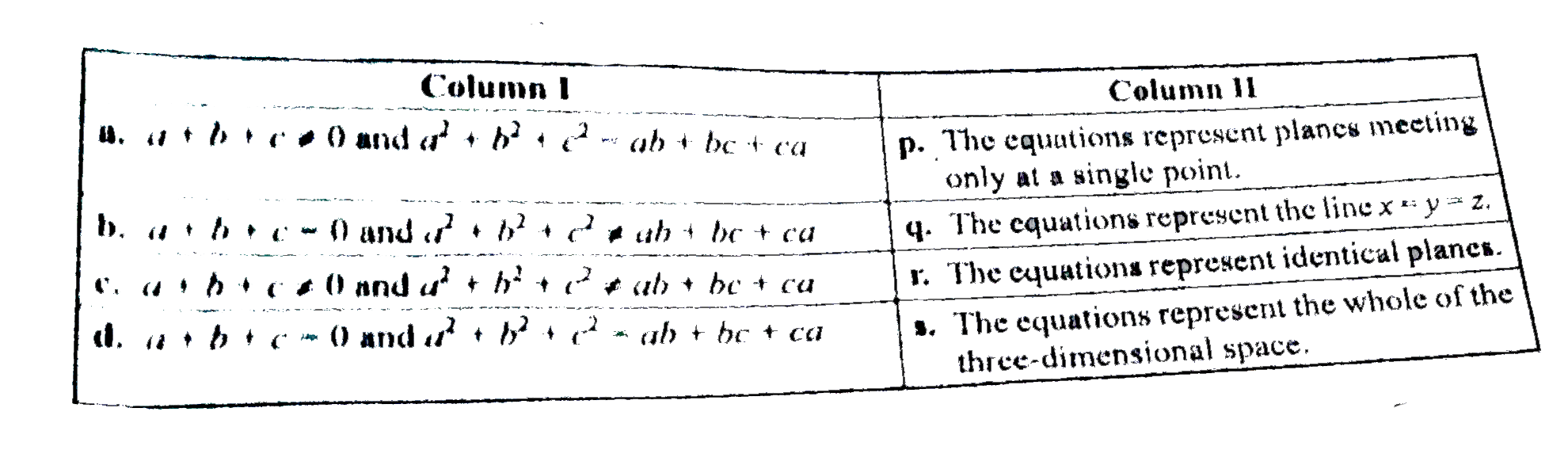
View SolutionProve that the system of equations in xa+y+z=0 , x+by+z=0 , x+y+cz=0 has a non - trivial solution then 11−a+11−b+11−c=
View SolutionCosider the equation ∫x0(t2−8t+13)dt=xsin(a/x)
The number of real values of x for which the equation has solution is
View Solution
