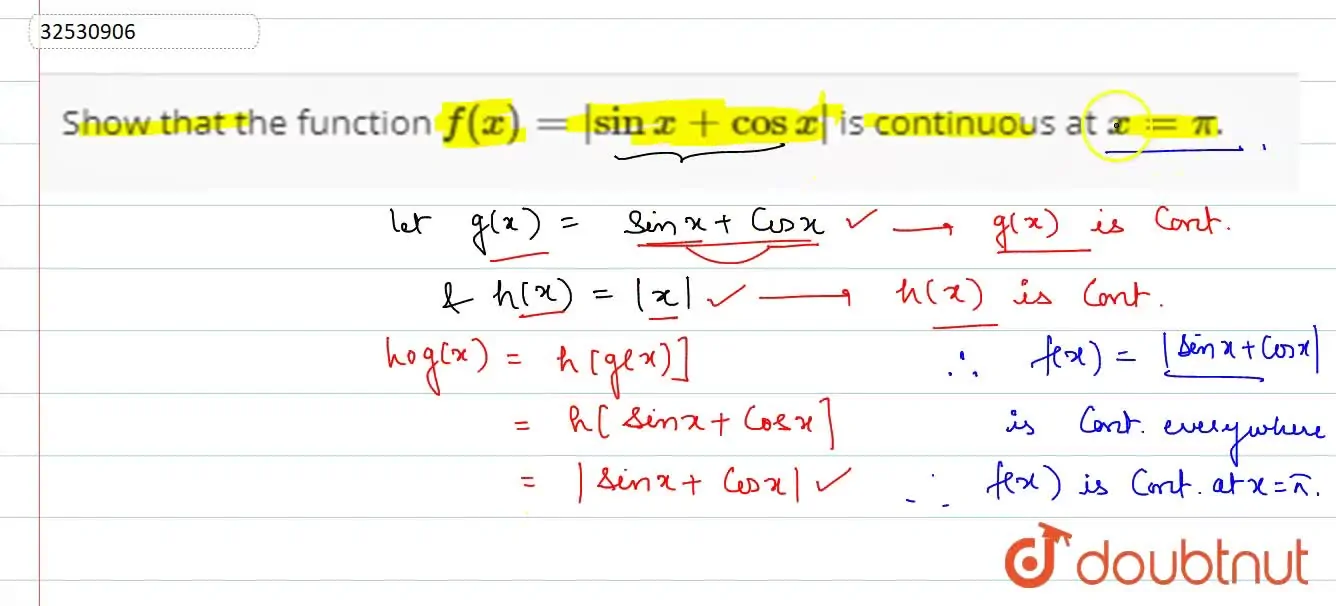
Show that the function f(x)=|sinx+cosx| is continuous at x=π.
Video Solution
Text Solution
The correct Answer is:N/a
We have, f(x)=|sinx+cosx| at x=π
Let g(x)=sinx+cosx
and h(x)=|x|
∴hog(x)=h[h(x)]
=h(sinx+cosx)
=|sinx+cosx|
Since, g(x)=sinx+cosx is a continuous function as it is forming with addition of two continous functions sinx and cosx.
Also, h(x)=|x| is also a continous function. Since, we know that composite function of two continuous functions is also a continuous function.
Hence, f(x)=|sinx+cosx| is a continuous function everywhere.
So, f(x) is continuous at x=π.
|
Updated on:21/07/2023
Related Playlists
Similar Questions
The period of the function
f(x)=|sinx|−|cosx| isView SolutionShow that the function f(x)=2x−|x| is continuous at x=0
View SolutionShow that the function defined by f(x)=|cosx| is a continuous function.
View SolutionShow that the function f(x)=2x−|x| is continuous at x=0 .
View SolutionShow that the function f(x)=2x−|x| is continuous at x=0.
View SolutionThe period of the function
f(x)=|sinx|−|cosx| , isView SolutionShow that the function defined by f(x)=cos(x2)is a continuous function.
View SolutionShow that the function defined by f(x)=sin(x2)is a continuous function.
View SolutionIf the function f(x)=x(esinx−1)1−cosx is continuous at x =0 then f(0)=
View SolutionThe period of the function
f(x)=||sinx|−|cosx∣∣ , isView Solution