The adjoining figure shows a square ABCD and an equilateral triangle DEC. Prove that :
(i) ∠ADE=∠BCE=30∘
(ii) ΔADE≅ΔBCE
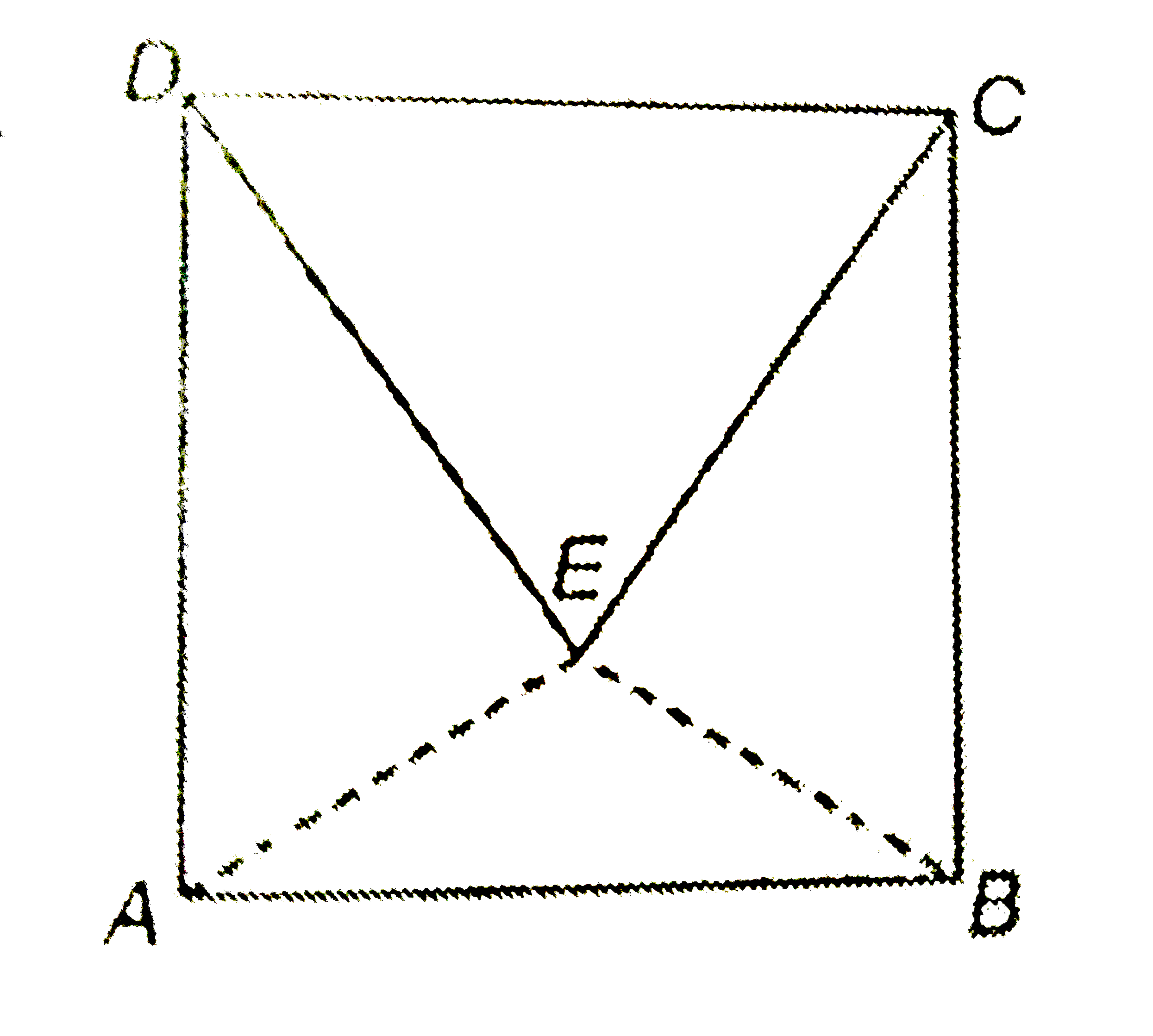
Step by step video & image solution for The adjoining figure shows a square ABCD and an equilateral triangle DEC. Prove that : (i) angleADE=angleBCE=30^(@) (ii) DeltaADE cong DeltaBCE by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7b
17 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Revision Exercise (very Short Answer Questions)
10 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
The following figure shows a square ABCD and an equilateral triangle DCE. Prove that :
(i) ∠ADE=∠BCE=150∘
(ii) ΔADE≅ΔBCE
(iii) AE=BE

View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
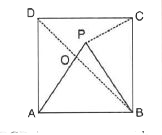
∠AOB
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
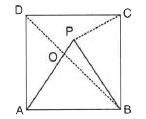
∠PCD
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
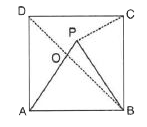
∠BPC
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
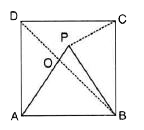
reflex ∠APC
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP.

Calculate :
(i) ∠AOB
(ii) ∠BPC
(iii) ∠PCD
(iv) reflex ∠APC
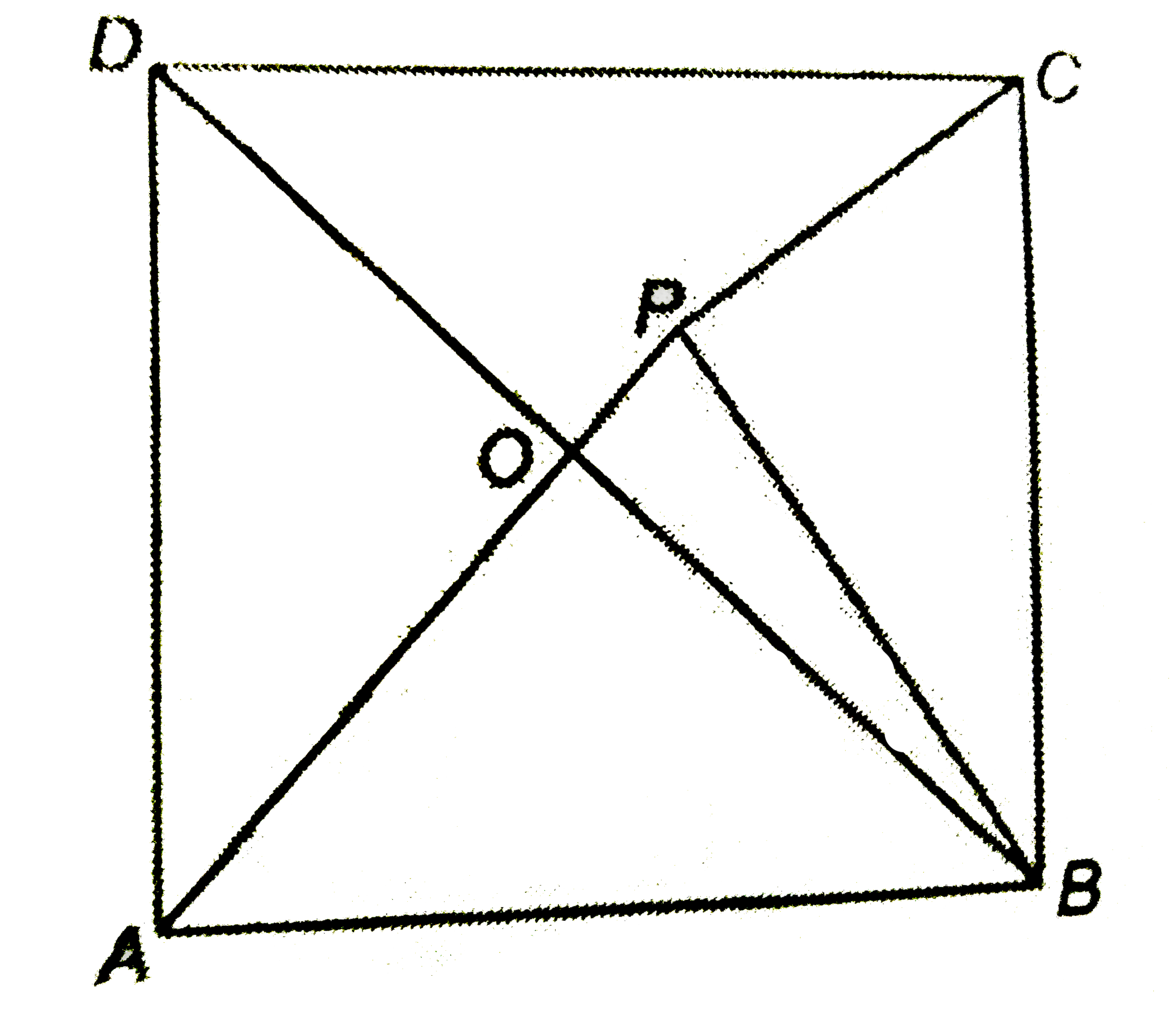
View SolutionThe give figure shows a square ABCD and an equilayeral teiangle APB. Calculate :
(1)∠AOB(ii)∠BPC(iii)∠PCD(iv)reflex∠APC
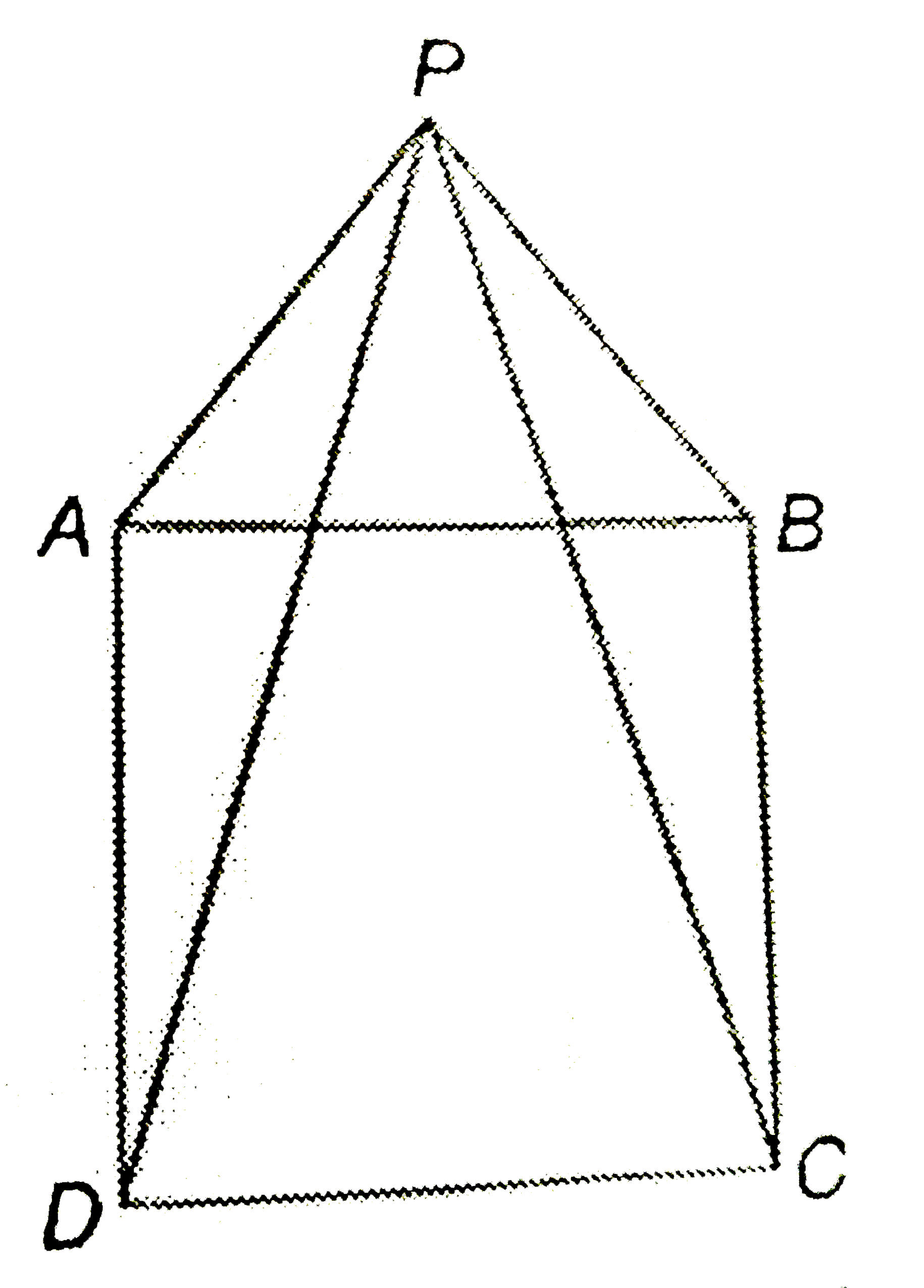
View SolutionIn the adjoining figure, ABCD is a square and PAB is an equilateral triangle. Find :
(i) ∠APD
(ii) ∠PDC
(iii) ∠DPC
(iv) Prove that DP=CP
View SolutionIn Figure, PQRS is a square and SRT is an equilateral triangle. Prove that PT=QT (ii) ∠TQR=150
View SolutionThe following figure shows a trapezium ABCD in which AB is parallel to DC and AD = BC.
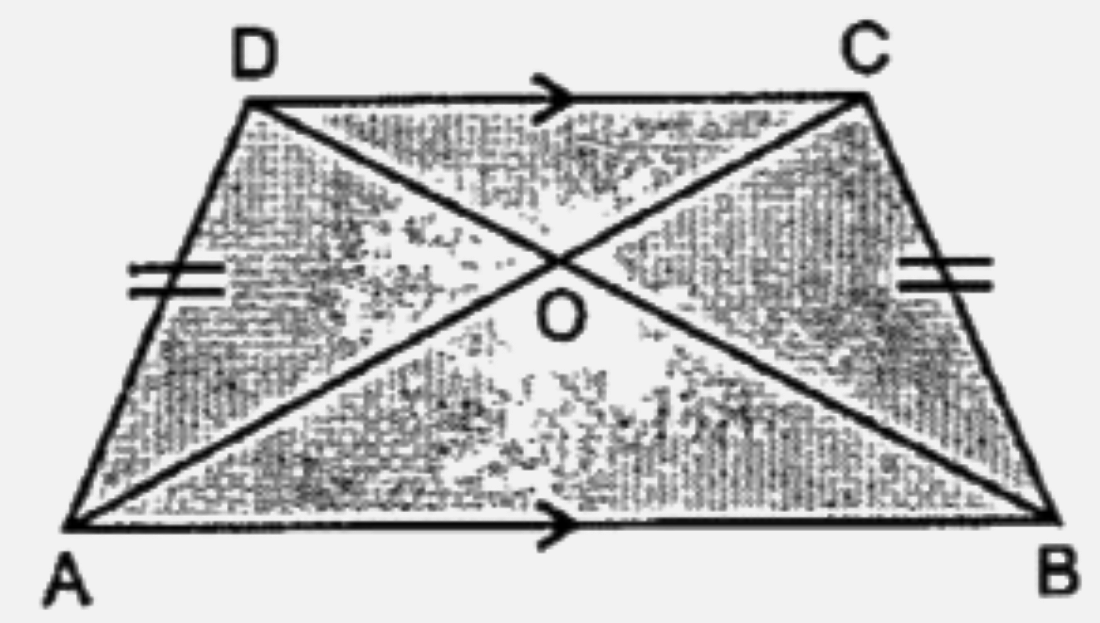
Prove that :
(i) ∠DAB=∠CBA
(ii) ∠ADC=∠BCD
(iii) AC = BD
(iv) OA = OB and OC = OD
View Solution
