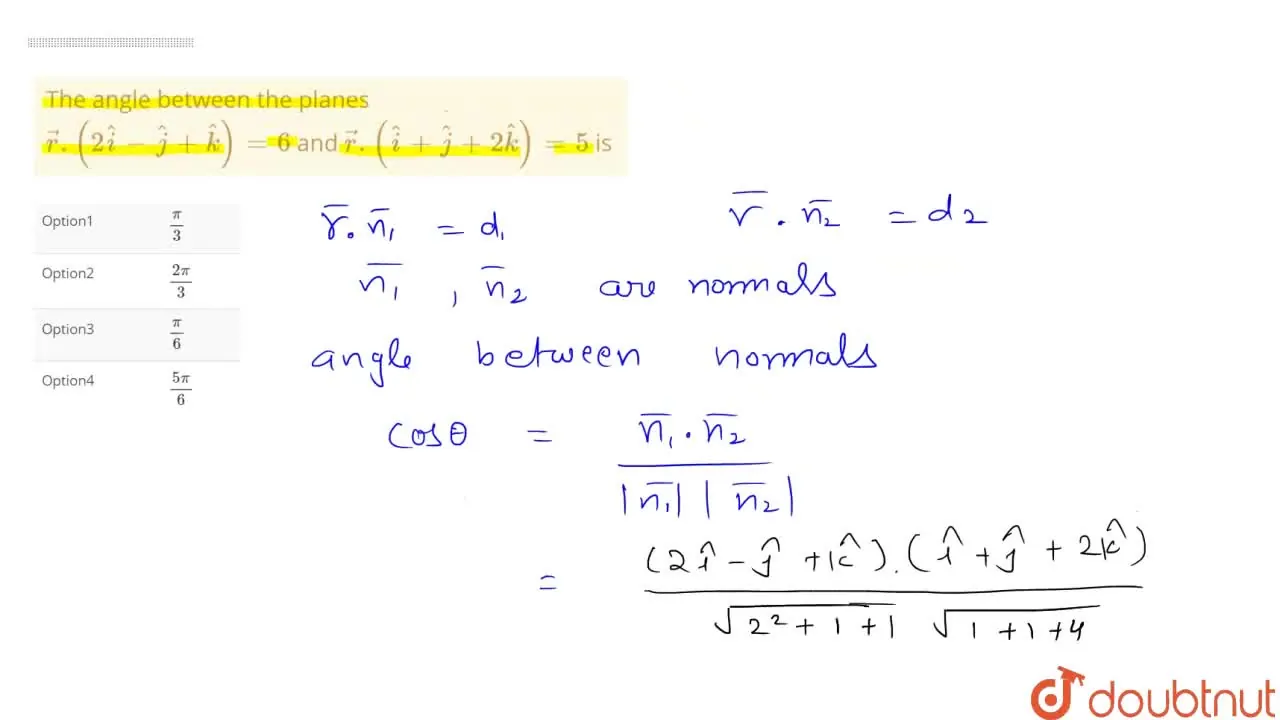
The angle between the planes
→r.(2ˆi−ˆj+ˆk)=6 and →r.(ˆi+ˆj+2ˆk)=5 is
π3
2π3
π6
5π6
The correct Answer is:A
We know that the angle between the planes →r.→n1=d1 and→r.→n2=d2 is given by
cosθ=→n1.→n2|→n1||→n2|
Here →n1=2ˆi−ˆj+ˆk and →n2=ˆi+ˆj+2ˆk
∴cosθ=(2ˆi−ˆj+ˆk).(ˆi+ˆj+2ˆk)∣∣2ˆi−ˆj+ˆk∣∣∣∣ˆi+ˆj+2ˆk∣∣=12
⇒θ=π/3
If a1x+b1y+c1z+d1=0 and a2x+b2y+c2z+d2=0 are Cartesian equations of two planes, then vectors normal to them are
→n1=a1ˆi+b1ˆj+c1ˆk and →n2=a2ˆi+b2ˆj+c2ˆk respectively.
Therefore, the angle θ between the planes is given by
costhet=→n1.→n2|→n1||→n2|
⇒cosθ=a1a2+b1b2+c1c2√a21+b21+c21√a21+b22+c22
If the planes are perpendicular then →n1 and →n2 are perpendicular.
∴→n1.→n2=0⇒a1a2+b1b2+c1c2=0
If the planes are parallel then →n1 and →n2 are parallel.
∴a1a2=b1b2=c1c2
Related Playlists
- BOOK - OBJECTIVE RD SHARMACHAPTER - PLANE AND STRAIGHT LINE IN SPACE EXERCISE - Section I - Solved Mcqs89videos
PLANE AND STRAIGHT LINE IN SPACE
- BOOK - OBJECTIVE RD SHARMACHAPTER - PLANE AND STRAIGHT LINE IN SPACE EXERCISE - Section II - Assertion Reason Type16videos
PLANE AND STRAIGHT LINE IN SPACE
- BOOK - OBJECTIVE RD SHARMACHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test30videos
MISCELLANEOUS EQUATIONS AND INEQUATIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMEXERCISE - Chapter Test55videos
PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEM
Similar Questions
The angles between the planes →r.(3ˆi−6ˆj+2ˆk)=4 and →r.(2ˆi−ˆj+2ˆk)=3, is
View SolutionFind the angle between the planes →r.(3ˆi−4ˆj+5ˆk)=0 and →r.(2ˆi−ˆj−2ˆk)=7.
View SolutionThe angle between the planes →r.(2ˆi−3ˆj+ˆk)=1and→r.(ˆi−ˆj)=4 is
View SolutionFind the angle between the planes →r.(ˆi+ˆj−2ˆk)=3and→r.(2ˆi−2ˆj+ˆk)=2
View SolutionFind the angle between the planes.
→r.(ˆi+ˆj+2ˆk)=5 and →r.(2ˆi−ˆj+ˆk)=8.View SolutionFind the angles between the planes →r(ˆi−2ˆj−2ˆk)=1 and →r(3ˆi−6ˆj+2ˆk)=0
View SolutionFind the angle between the planes →r.(ˆi+ˆj)=1 and →r.(ˆi+ˆk)=3.
View SolutionThe distance between the planes given by
→r.(ˆi+2ˆj−2ˆk)+5=0 and →r.(ˆi+2ˆj−2ˆk)−8=0 isView SolutionThe line of intersection of the planes
→r.(3ˆi−ˆj+ˆk)=1 and →r.(ˆi+4ˆj−2ˆk)=2 is parallel isView SolutionEquation of a plane passing through the intersection of the planes →r.(3ˆi−ˆj+ˆk)=1 and →r.(ˆi+4ˆj−2ˆk)=2 and passing through the point (ˆi+2ˆj−ˆk) is :
View Solution