The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
To find the height of the tower given the angle of elevation and the distance from the tower, we can follow these steps:
Step 1: Understand the Problem
We have a right triangle formed by the height of the tower, the distance from the point on the ground to the foot of the tower, and the line of sight to the top of the tower. The angle of elevation from the point on the ground to the top of the tower is given as 30∘, and the distance from the point to the foot of the tower is 30 meters.
Step 2: Set Up the Triangle
Let:
- H be the height of the tower.
- The distance from the point on the ground to the foot of the tower is 30 m.
- The angle of elevation is 30∘.
Step 3: Use the Tangent Function
In the right triangle, we can use the tangent function, which is defined as the ratio of the opposite side (height of the tower H) to the adjacent side (distance from the tower, which is 30 m).
tan(30∘)=H30
Step 4: Substitute the Value of tan(30∘)
We know that:
tan(30∘)=1√3
Substituting this value into the equation gives:
1√3=H30
Step 5: Solve for H
To find H, we can cross-multiply:
H=30⋅1√3
This simplifies to:
H=30√3
Step 6: Rationalize the Denominator
To rationalize the denominator, multiply the numerator and denominator by √3:
H=30⋅√33=10√3
Conclusion
The height of the tower is:
H=10√3 meters
---
We have a right triangle formed by the height of the tower, the distance from the point on the ground to the foot of the tower, and the line of sight to the top of the tower. The angle of elevation from the point on the ground to the top of the tower is given as 30∘, and the distance from the point to the foot of the tower is 30 meters.
Let:
- H be the height of the tower.
- The distance from the point on the ground to the foot of the tower is 30 m.
- The angle of elevation is 30∘.
In the right triangle, we can use the tangent function, which is defined as the ratio of the opposite side (height of the tower H) to the adjacent side (distance from the tower, which is 30 m).
We know that:
tan(30∘)=1√3
1√3=H30
To find H, we can cross-multiply:
H=30⋅1√3
H=30√3
To rationalize the denominator, multiply the numerator and denominator by √3:
H=30⋅√33=10√3
The height of the tower is:
H=10√3 meters
Similar Questions
The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution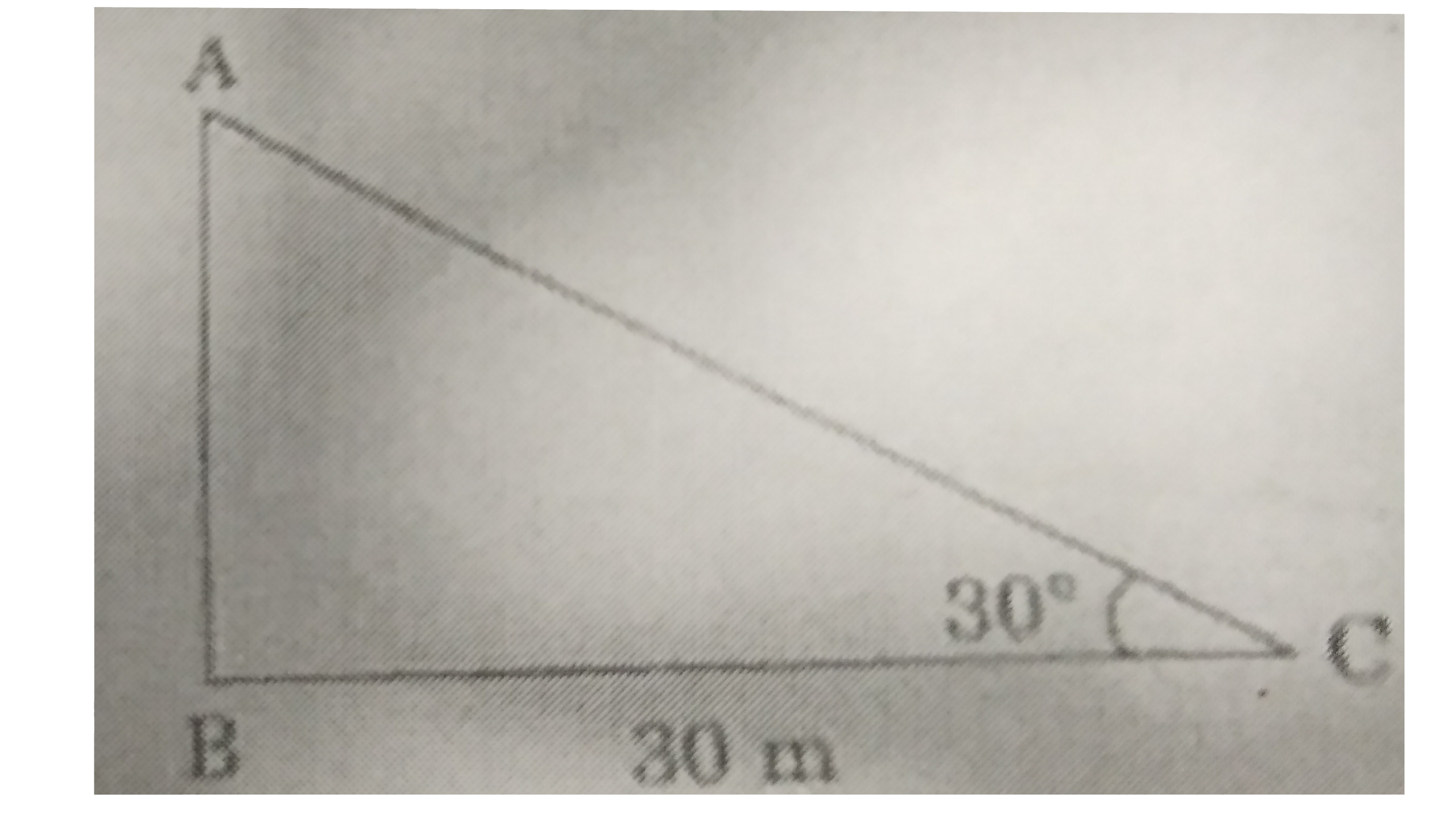
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.View Solution
Knowledge Check
- Question 1 - Select One
The angle of elevation of the top of a tower. from a point on the ground and at a distance of 160 m from its foot, is find to be 60∘ . Find the height of the tower .
A277.12 mB377.12 mC477.12 mDNone
The angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.
 View Solution
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View SolutionFrom the top of a 7 m high building, the angle of elevation of the top of a tower is 60° and the angle of depression of the foot of the tower is 30°. Find the height of the tower.
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
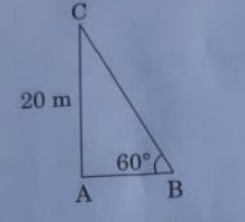 View Solution
View SolutionThe angle of elevation of the top of a tower at any point on the ground is 30∘ and moving 20 metres towards the tower it becomes 60∘. The height of the tower is
View Solution
