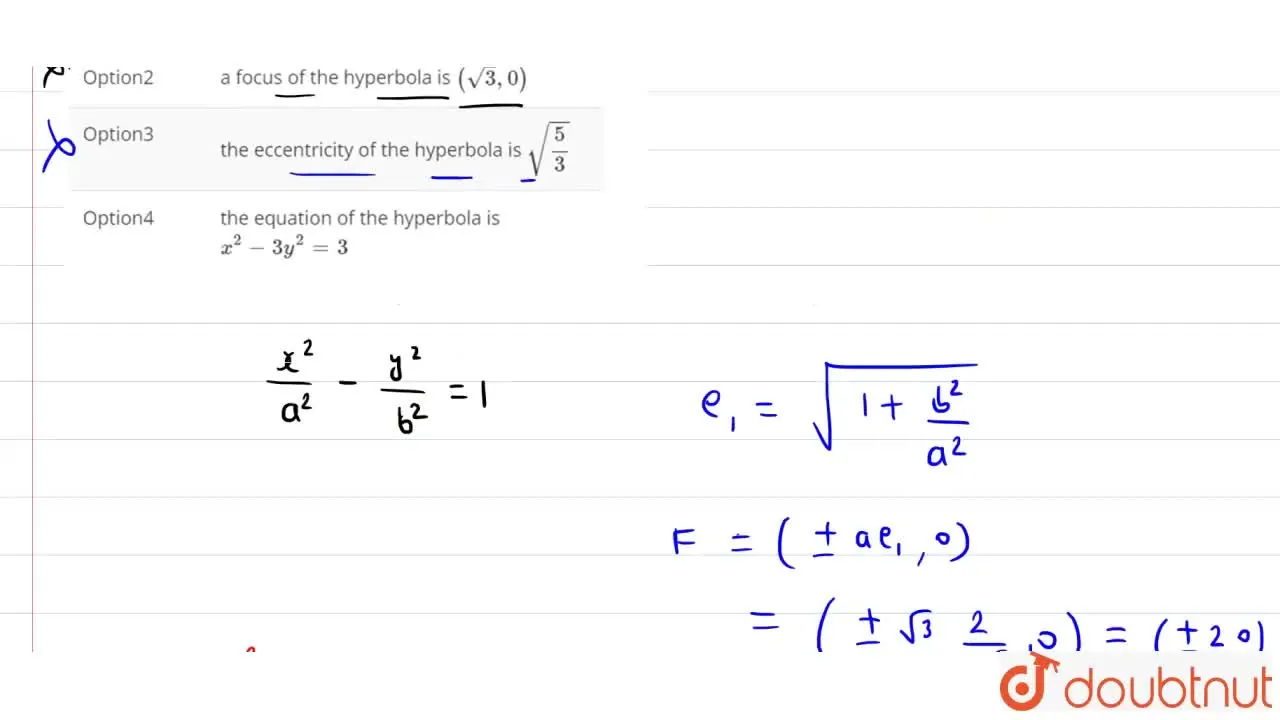
The eccentricity of the hyperbola x2a2−y2b2=1 be reciprocal to that of the ellipse x2+4y2=4. If the hyperbola passes through a focus of the ellipse, then :
The equation of the hyperbola is x23−y22=1
a focus of the hyperbola is (√3,0)
the eccentricity of the hyperbola is √53
the equation of the hyperbola is x2−3y2=3
The correct Answer is:D
Eccentricity of the ellipse =√1−14=√32
Focus of the ellipse =(−√3,0)
Eccentricity of the hyperbola =√1+b2a2=2√3⇒ba=1√3
Since the hyperbola passes through the focus of the ellipse 3a2−0=1⇒a2=3 and b2=1
and equation of the hyperbola is x23−y21=1 or x2−3y2=3
Focus of hyperbola is (±2,0)
Related Playlists
Similar Questions
Let the eccentricity of the hyperbola x2a2−y2b2=1 be the reciprocal to that of the ellipse x2+4y2=4. If the hyperbola passes through a focus of the ellipse, then the equation of the hyperbola, is
View SolutionThe eccentricity of the hyperbola 3x2−4y2=−12 is
View SolutionThe eccentricity of the hyperbola 3x2−y2=4 is :
View SolutionFind the eccentricity of the hyperbola 9y2−4x2=36
View SolutionLet the eccentricity of the hyperbola x2a2−y3b2=1 be reciprocal to that of the ellipse x2+9y2=9, then the ratio a2:b2 equals
View SolutionThe eccentricity of the hyperbola √20064(x2−y2)=1 is
View SolutionEccentricity of the hyperbola x2−y2=a2 is :
View SolutionFind the eccentricity of the hyperbola 9y2−4x2=36
View SolutionThe eccentricity of the hyperbola 5x2−4y2+20x+8y=4 is :
View SolutionThe eccentricity of the hyperbola 5x2−4y2+20x+8y=4 is
View Solution