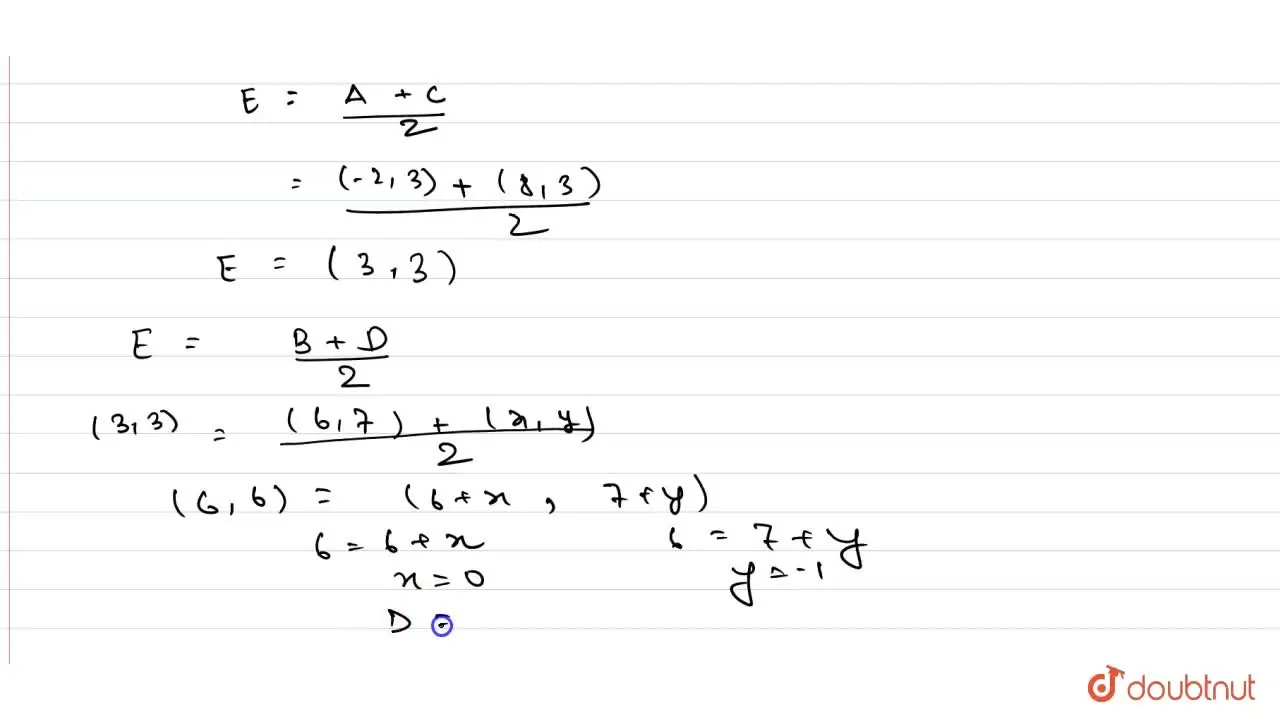
The fourth vertex D of a parallelogram ABCD whose three vertices are A(−2,3), B(6,7) and C(8,3)is
(0,1)
(0,−1)
(−1,0)
(1,0)
The correct Answer is:B
Let the fourth vertex of parallelogram , D ≡(x4,y4)and L,M be the middle points of AC and BD , respectively.
Then , L ≡(−2+82,3+32)≡(3,3)
[Since , mid - point of a line segment having points (x1,y1and(x2,y2)=(x1+x22,y1+y22)]
and ≡(6+x42,7+y42)

Since , ABCD is a parallelogram, therefore diagonals AC and BD will bisect each other. Hence ,L and M are the same points. ∴3=6+x42and3=7+y42
⇒6=6+x4and6=7+y4
⇒x4=0andy4=6−7
∴x4=0andy4=−1
Hence , the fourth vertex of parallelogram is D ≡(x4,y4)≡(0,1).
Related Playlists
- BOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.4 Long Answer type Questions7videos
CONSTRUCTIONS
- BOOK - NCERT EXEMPLARCHAPTER - INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSEXERCISE - Introduction To Trigonometry And Its Applications60videos
INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONS
Similar Questions
Find the possible pairs of co-ordinates of the fourth vertex D of the parallelogram, if three of its vertices are A(5,6),B(1,2)andC(3,−2)
View SolutionWhat is the fourth vertex of a parallelogram, if its three consecutive vertices are (-2,-1), (1,0) and (4,3)?
View SolutionFind the fourth vertex of the parallelogram whose three consecutive vertices are (8,8),(6,1) and (−1,1) .
View SolutionThe fourth vertex of a parallelogram,whose consecutive vertices are (3,−4),(−2,1)and(-6,5) is
View SolutionFind the possible pairs of co-ordinates of the fourth vertex D of the parallelogram if three of its verices are A (5,6) , B (1,-2) and C (3,-2) .
View SolutionFind the area of a parallelogram ABCD if three of its vertices are A(2, 4), B(2+√3, 5) and C(2, 6) .
View SolutionThe three vertices of a parallelogram ABCD taken in order are A(3, -4), B(-1, -3) and C(-6, 2). Find the coordinates of the fourth vertex D.
View SolutionFind the area of a quadrilateral ABCD whose vertices are A(-4, 8), B(-3, -4), C(0, -5) and D(5, 6).
View SolutionThe three vertices of a parallelogram ABCD are A(-1,3,4), B(2,-1,3) and C(5,1,2). Find the co-ordinates of its 4th vertex D.
View SolutionThe co-ordinates of three vertices of a parallelogram ABCD are A(1,0) , B(3,4) and C(1,2). The co-ordinates of fourth vertex D are :
View Solution