The graph of a linear programming problem is given below. The shaded region is the feasible region. The objective function is Max Z=px+qy
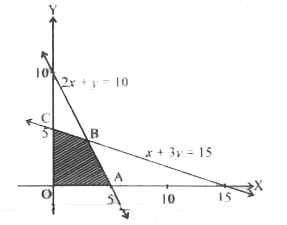
(i) What are the coordinates of the corners of the feasible region.
(ii) Write the constraints.
(iii) If the Z occurs at A and B, what is the relation between p and q.
(iv) If q=1 write the objective, function.
(v) Find the Max Z. Solution in Tamil
Step by step video & image solution for The graph of a linear programming problem is given below. The shaded region is the feasible region. The objective function is Max Z=px+qy (i) What are the coordinates of the corners of the feasible region. (ii) Write the constraints. (iii) If the Z occurs at A and B, what is the relation between p and q. (iv) If q=1 write the objective, function. (v) Find the Max Z. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - LINEAR PROGRAMMING EXERCISE - NECERT TEXT BOOK EXERCISE 12.110videos
LINEAR PROGRAMMING
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - LINEAR PROGRAMMING EXERCISE - NECERT TEXT BOOK EXERCISE 12.210videos
LINEAR PROGRAMMING
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - LINEAR INEQUALITIESEXERCISE - QUESTIONS FROM COMPETITIVE EXAMS38videos
LINEAR INEQUALITIES
- BOOK - NEW JOYTHI PUBLICATIONCHAPTER - MATHEMATICAL REASONINGEXERCISE - QUESTION FROM COMPETITIVE EXAMS23videos
MATHEMATICAL REASONING
Similar Questions
Maximise Z=3x+4y subject to the constraints x+y≤,4,x≥0,y≥0
04:57View SolutionReshma wishes to mix two types of food P and Q in such a way that the vitamin contents of the mixture contain atleast 8 units of vitamin A and 11 units of vitamin B. Food P costs Rs. 60// kg and food Q cost Rs. 80/kg. food P contains 3 units/ kg of vitamin A and 5 units/ kg of vitamin B while food Q contains 4 units/kg vitamin A and 2 units/kg of vitamin B. Determine the minimum cost of the mixture .
03:53View SolutionThe set of all x satisying the inequility 4x−13x+1≥1 is
05:41View SolutionThe statement ~(p→q) is equivalent to
02:03View SolutionFind the corner points of the feasible region of the linear programming problem;,Max Z=x : Subject to the constraints 3x+2y =0,y>=0
02:10View SolutionFind the area of the shaded region, given that the radius of each circle is equal to 5 cm.
02:17View SolutionMatch the following Column A to Column B
 02:10View Solution
02:10View SolutionThe area of the shaded part in the figure given below is ______.
 04:23View Solution
04:23View SolutionThe inequation represented by the graph given below is :
03:02View SolutionThe inequation that best describes the graph given below is _________
01:15View SolutionThe inequation that best describes the following graph is _________
03:41View SolutionWhich inequations represent the shaded region in the given figure
04:36View SolutionIn the given figure, PQRS is a parallelogram. A and B are the mid-point of ¯¯¯¯¯¯PQ and ¯¯¯¯¯SR respectively. If PS=BR, then the quadrilateral ADBC is a…………………… ,
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_E05_020_Q01.png" width="80%"gt00:44View Solutionవేర్ల ద్వారా శాఖీయోత్పత్తి జరిపే మొక్కbr i) డాలియాbr ii) ముల్లంగిbr iii) క్యారెట్br iv) క్యాబేజిbr
07:24View SolutionThe corner points of the feasible region determined by the system of linear constraints are as shown below:
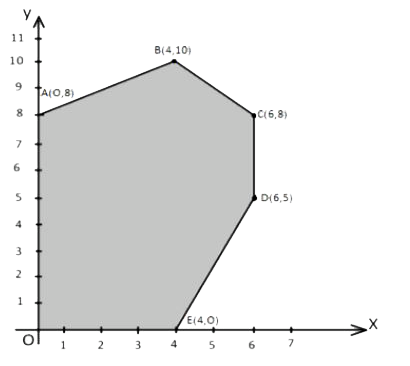
Let Z=px+qy where p,q>0 be the objective function. Find the condition on p and q so that the maximum value of Z occurs atB(4,10)andC(6,8)Also mention the number of optimal solutions in this case.05:47View Solution

