The list of number -10, -6, -2, 2, … is
an AP with d = -16
an AP with d = 4
an AP with d = -4
not an AP
The correct Answer is:B
To determine if the list of numbers -10, -6, -2, 2 is an arithmetic progression (AP), we need to find the common difference (D) between consecutive terms.
1. Identify the terms:
- First term (A1) = -10
- Second term (A2) = -6
- Third term (A3) = -2
- Fourth term (A4) = 2
2. Calculate the common difference (D):
- Find D by subtracting the first term from the second term:
D=A2−A1=−6−(−10)=−6+10=4
3. Check the next pair of terms:
- Now, calculate the difference between the second term and the third term:
D=A3−A2=−2−(−6)=−2+6=4
4. Check the last pair of terms:
- Finally, calculate the difference between the third term and the fourth term:
D=A4−A3=2−(−2)=2+2=4
5. Conclusion:
- Since the common difference (D) is the same (4) for all pairs of consecutive terms, we can conclude that the list of numbers forms an arithmetic progression (AP) with a common difference of 4.
Final Answer:
The list of numbers -10, -6, -2, 2 is an AP with D = 4.
- First term (A1) = -10
- Second term (A2) = -6
- Third term (A3) = -2
- Fourth term (A4) = 2
- Find D by subtracting the first term from the second term:
D=A2−A1=−6−(−10)=−6+10=4
- Now, calculate the difference between the second term and the third term:
D=A3−A2=−2−(−6)=−2+6=4
- Finally, calculate the difference between the third term and the fourth term:
D=A4−A3=2−(−2)=2+2=4
- Since the common difference (D) is the same (4) for all pairs of consecutive terms, we can conclude that the list of numbers forms an arithmetic progression (AP) with a common difference of 4.
The list of numbers -10, -6, -2, 2 is an AP with D = 4.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Very Short Answer Type Questions8videos
ARITHMETIC PROGRESSIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Short Answer Type Questions35videos
ARITHMETIC PROGRESSIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - AREAS RELATED TO CIRCLEEXERCISE - Long Answer Type Questions20videos
AREAS RELATED TO CIRCLE
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - EXERCISE 9.4 LONG ANSWER TYPE QUESTIONS14videos
CIRCLES
Similar Questions
Knowledge Check
- Question 1 - Select One
The average (arithemic mean) of a list of 5 numbers is n. When an additional number of added to the list, the average of all 6 numbers in n+3. Which of the following is the value, in terms of n, of the added to the list?
A6n+18B5nCn+18Dn+6 - Question 2 - Select One
2 is 10% of what number.
A10B12C20D24
An unbiased dice, with faces numbered 1, 2, 3, 4, 5, 6, is thrown n times and the list of n numbers shown up is noted. Then find the probability that among the numbers 1, 2, 3, 4, 5, 6 only three numbers appear in this list and each number appears at least once.
View SolutionAn unbiased dice, with faces numbered 1,2,3,4,5,6, is thrown n times and the list of n numbers shown up is noted. Then find the probability that among the numbers 1,2,3,4,5,6 only three numbers appear in this list.
View SolutionAn unbiased dice, with faces numbered 1,2,3,4,5,6, is thrown n times and the list of n numbers shown up is noted. Then find the probability that among the numbers 1,2,3,4,5,6 only three numbers appear in this list.
View SolutionWhich of the following list of numbers does form an AP? If they form an AP. write the next two terms :(i) 4, 10, 16. 22, . . .(ii) 1,-1, -3,-5, . . .(iii) -2,2,-2,2, . . .(iv)1,1,1,2,2,2,3,3,3, . . .
View SolutionFour numbers are such that the first three are in.A.P while the last three are in G.P. If the first number is 6 and common ratio of G.P. is 12 the the number are (A) 6,8,4,2 (B) 6,10,14,7 (C) 6,9,12,6 (D) 6,4,2,1
View Solution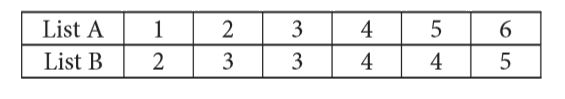
The table above shows two lists of numbers. Which of the following is a true statement comparing list A and list B ?View Solution

