Question

The minimum and maximum values problem, of Z for the minimise and maximise Z = 3x + 9 y subject to the constraints
$x+3y\le 60,x+y\ge 10,x\le y,$
are respectively

Options

A. 60 and 180
B. 180 and 60
C. 50 and 190
D. 190 and 50

Answer

A

Explanation

Given Z= 3x+9y
Subject to the constraints
` x +3y le 60`
` x+y ge 10 `
` x le y`
and ` x ge 0, y ge 0`
The feasible region is ABCD whose corner points A,B,C and D are (0,15),(5,5),(15,5) and (0,20) respectively .

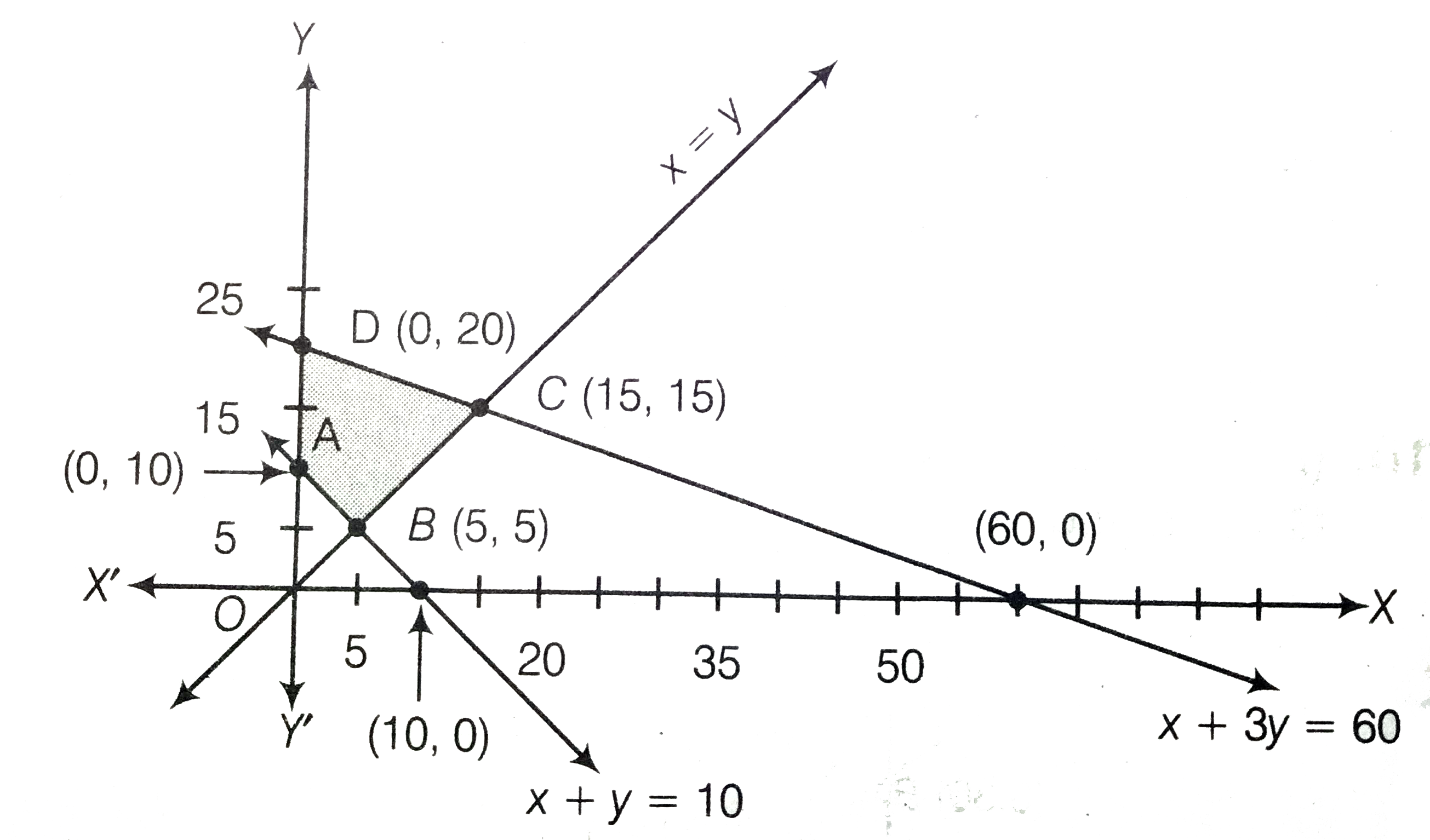
Form the table, the minimum value of Z is 60 and the maximum value of Z is 180.