The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. Find the probability that out of 5 such bulbs :
(i) none
(ii) none more than one
(iii) more than one
(iv) at least one
will fuse after 150 days of use.
To solve the problem, we need to calculate the probabilities for different scenarios regarding the fusing of bulbs. We will use the binomial probability formula since we are dealing with independent trials (the fusing of each bulb does not affect the others).
Given:
- Probability of a bulb fusing after 150 days (p) = 0.05
- Probability of a bulb not fusing (q) = 1 - p = 0.95
- Number of bulbs (n) = 5
Step 1: Calculate the probability that none of the bulbs fuse (P(X = 0))
Using the binomial probability formula:
P(X=k)=(nk)pkqn−k
For none of the bulbs fusing (k = 0):
P(X=0)=(50)(0.05)0(0.95)5
=1⋅1⋅(0.95)5
=(0.95)5
=0.77378 (approximately)
Step 2: Calculate the probability that none or more than one bulb fuses (P(X ≤ 1))
This includes the probabilities for 0 and 1 bulb fusing:
P(X≤1)=P(X=0)+P(X=1)
First, we already calculated P(X=0). Now, we calculate P(X=1):
P(X=1)=(51)(0.05)1(0.95)4
=5⋅(0.05)⋅(0.95)4
=5⋅0.05⋅0.8145
=0.20348 (approximately)
Now, add both probabilities:
P(X≤1)=P(X=0)+P(X=1)
=0.77378+0.20348
=0.97726 (approximately)
Step 3: Calculate the probability that more than one bulb fuses (P(X > 1))
This can be calculated as:
P(X>1)=1−P(X≤1)
=1−0.97726
=0.02274 (approximately)
Step 4: Calculate the probability that at least one bulb fuses (P(X ≥ 1))
This can be calculated as:
P(X≥1)=1−P(X=0)
=1−(0.95)5
=1−0.77378
=0.22622 (approximately)
Summary of Results:
1. Probability that none of the bulbs fuse: 0.77378
2. Probability that none or more than one bulb fuses: 0.97726
3. Probability that more than one bulb fuses: 0.02274
4. Probability that at least one bulb fuses: 0.22622
- Probability of a bulb fusing after 150 days (p) = 0.05
- Probability of a bulb not fusing (q) = 1 - p = 0.95
- Number of bulbs (n) = 5
P(X=k)=(nk)pkqn−k
P(X=0)=(50)(0.05)0(0.95)5
=1⋅1⋅(0.95)5
=(0.95)5
=0.77378 (approximately)
P(X≤1)=P(X=0)+P(X=1)
P(X=1)=(51)(0.05)1(0.95)4
=5⋅(0.05)⋅(0.95)4
=5⋅0.05⋅0.8145
=0.20348 (approximately)
P(X≤1)=P(X=0)+P(X=1)
=0.77378+0.20348
=0.97726 (approximately)
P(X>1)=1−P(X≤1)
=1−0.97726
=0.02274 (approximately)
P(X≥1)=1−P(X=0)
=1−(0.95)5
=1−0.77378
=0.22622 (approximately)
1. Probability that none of the bulbs fuse: 0.77378
2. Probability that none or more than one bulb fuses: 0.97726
3. Probability that more than one bulb fuses: 0.02274
4. Probability that at least one bulb fuses: 0.22622
Topper's Solved these Questions
- PROBABILITYBOOK - MODERN PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Miscellaneous Exercise on Chapter 13
19 Videos
- PROBABILITYBOOK - MODERN PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Exercise
5 Videos
- PROBABILITYBOOK - MODERN PUBLICATIONCHAPTER - PROBABILITYEXERCISE - NCERT-FILE (Exercise 13.4)
17 Videos
- MATRICESBOOK - MODERN PUBLICATIONCHAPTER - MATRICESEXERCISE - CHAPTER TEST (3)
12 Videos
- RELATIONS AND FUNCTIONSBOOK - MODERN PUBLICATIONCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - CHAPTER TEST (1)
12 Videos
Similar Questions
The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. Find the probability that out of 5 such bulbs(i) none(ii) not more than one(iii) more than one(iv) at least onewill fuse after 150 days of use.
View SolutionThe probability that a bulb produced by a factory will fuse after an year of use is 0.1. Then the probability that out of 4 such bulbs, only one is working after an year
View Solution
Knowledge Check
The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. What is the probability that out of 5 such bulbs none will fuse after 150 days of use?
A1−(1920)5B(1920)5C(34)5D90(14)5The probability that tulb produced by a factor will fuse after 150 days if used is 0.50. What is the probability that out of 5 such balbs none will fuse after 150 days of use ?
A1−(19/20)5B(19/20)5C(3/4)5D90(1/4)5
The probability that a bulb produced by a factory will fuse after 160 days of use is 0.06. Find the probability that out of 5 such bulbs at the most one bulb will fuse after 160 days of use.
View SolutionThe probability that a bulb produced by a factory will fuse after 6 months of use is 0.05 . Find the probability that out of 5 such bulbs
(i) none will fuse after 6 months of use
(ii) at least one will fuse after 6 months of use
(iii) not more than one will fuse after 6 months of use.
View SolutionThe probability that a bulb produced by a factory will fuse after 150 days if used is 0.05. what is the probability that out of 5 such bulbs none will fuse after 150 days of use? 1−(1920)5 b. (1920)5 c. (34)5 d. 90(14)5
View SolutionThe probability that the life of a bulb is 100 days is 0.05. Find the probability that out of 6 bulbs (i) atleast 1 (ii) greater than 4 (iii) none will be having a life of 100 days
View SolutionThe probability that a bulb produced by a factory will fuse after 200 days of use is 0.2. Let X denote the number of bulbs (out of 5 ) that fuse after 200 days of use . Find the probability of
(i)X=0,(ii)X≤1,(iii)X>1,(iv)X≥1.
View SolutionThe probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. If a sample of such 5 bulbs are taken out, then match the probabilities in column I with their respective values in column II and choose the correct option from the codes given below.
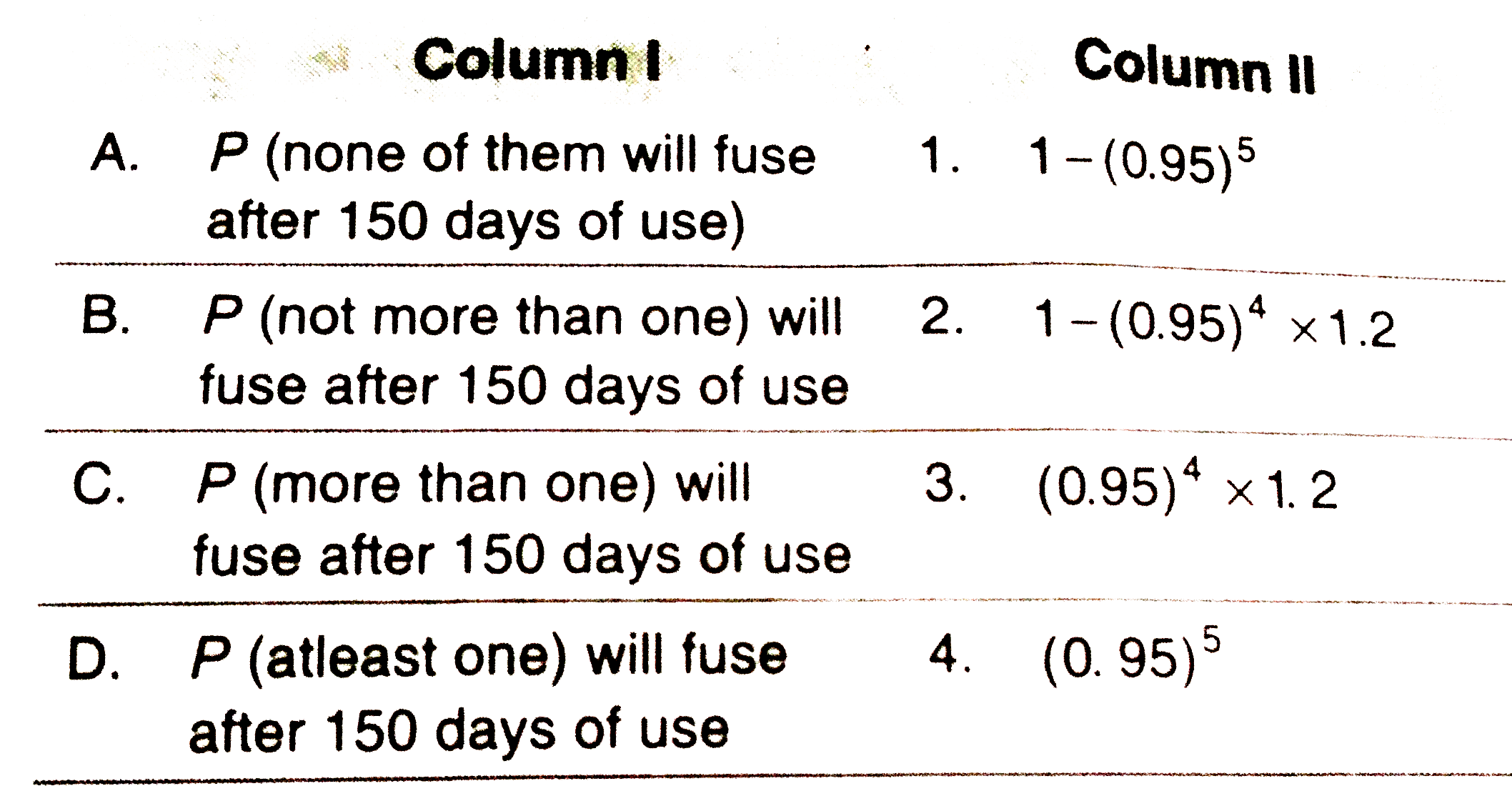
View Solution
