The shadow of a tower at a time is three times as long as its shadow when the angle of elevation of the Sun is 60∘. Find the angle of elevation of the Sum at the time of the longer shadow.

A

600

B

300

C

450

D

150

Text Solution
Verified by Experts

The correct Answer is:B

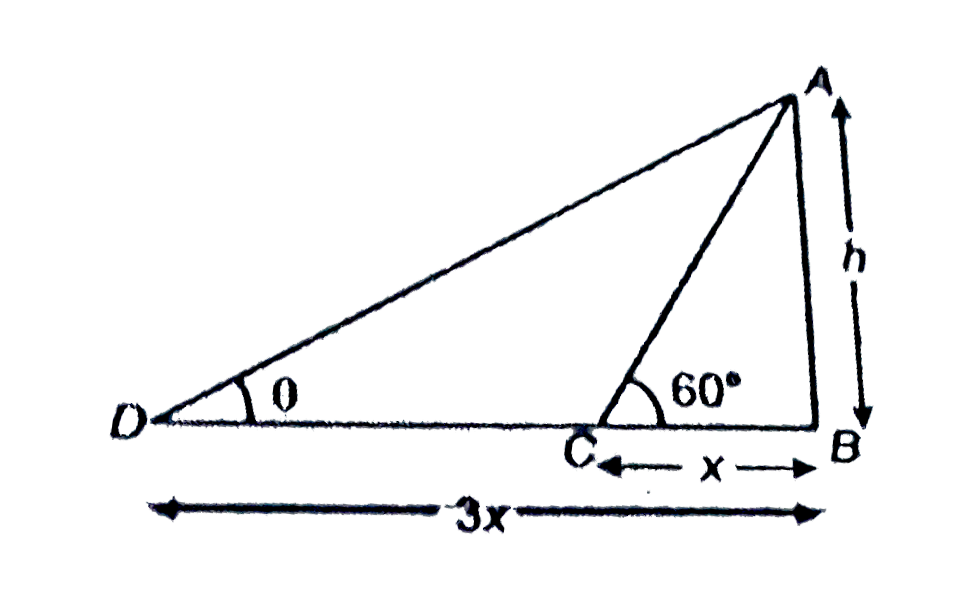
Let AB = h be the height of a tower.
Let at Sun's elevation of 60∘, its shadow be x.
some Applications of Trigonometry
Let θ be the angle of elevation when its shadow is 3 times the former i.e., 3x.
In right ΔABC,
tan60∘=hx⇒√3=hx⇒h=x√3...(1)
In right ΔABD,
tanθ=h3x
=x√33x [from (1)]
=1√3=tan30∘
θ30∘
ocr_image

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 10MATHSSOME APPLICATIONS OF TRIGONOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • If the length of the shadow of a tower is √3 times its height of then the angle of elevation of the sun is

    A45∘
    B30∘
    C60∘
    D90∘

  • The shadow of a tower is √3 times its height .Then the angle of elevation of the top of the tower is

    A45∘
    B30∘
    C60∘
    D90∘

  • The shadow of a flagstaff is three times as long as the shadow of the flagstaff when the sun rays meet the ground at 60∘ . Find the angle between the sun rays and the ground at the time of longer shadow

    A45∘
    B30∘
    C15∘
    D90∘