The value of the integral ∫10xcot−1(1−x2+x4) dx is
A
π4−12loge2
B
π2−12loge2
C
π4−loge2
D
π2−loge2
Video Solution
Text Solution
The correct Answer is:A
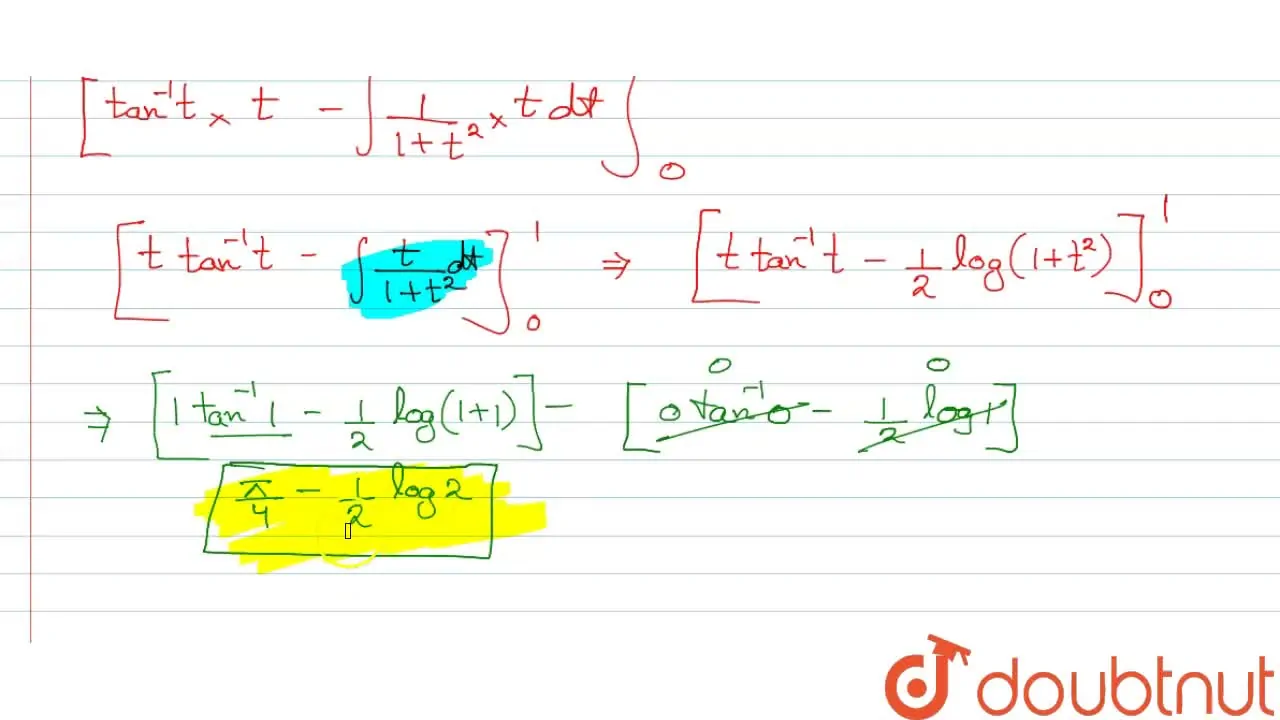
Let I=∫10xcot−1(1−x2+x4)dx
Now , put x2=t⇒ 2x dx = dt
Lower limit at x = 0 , t = 0
Upper limit at x = 1 , t = 1 ∴I=12∫10cot−1(1−t+t2)dt
=12∫10tan−1(11−t+t2)dt [∵cot−1x=tan−11x]
=12∫10tan−1(t−(t−1)1+t(t−1))dt
=12[∫10(tan−1t−tan−1)dt]
[∵tan−1x−y1+xy=tan−1x−tan−1y]
∵∫10tan−1(t−1)dt=∫−10tan−1(1−t−1)dt=−∫10tan−1(t)dt
because ∫a0f(x)dx=∫a0f(a−x)dx
So , I=12∫10(tan−1t+tan−1t)dt
=∫10tan−1tdt=[tan−1t]10−∫10t1+t2dt
[ by intergration by parts method]
=πp−12[loge(1+t2)]10=π4−12−loge2
|
Updated on:21/07/2023
Related Playlists
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - DEFINITE INTEGRATIONEXERCISE - PERIODICITY OF INTEGRAL FUNCTIONS6videos
DEFINITE INTEGRATION
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - DEFINITE INTEGRATIONEXERCISE - ESTIMATION, GAMMA FUNCTION AND DERIVATIVE OF INDEFINITE INTEGRAL22videos
DEFINITE INTEGRATION
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 5 DE-MOIVRES THEOREM,CUBE ROOTS AND nth ROOTS OF UNITY (INTEGER ANSWER TYPE QUESTION)1videos
COMPLEX NUMBERS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - TOPIC 3 APPLICATIONS OF HOMOGENEOUS DIFFERENTIAL EQUATIONS <br> ANALYTICAL & DESCRIPTIVE QUESTIONS7videos
DIFFERENTIAL EQUATIONS
Similar Questions
The value of the integral 1∫0cot−1(1−x+x2)dx, is
View SolutionThe value of the integral ∫10cot−1(1−x+x2)dx, is
View SolutionThe value of the integral ∫∞011+x4dx is
View SolutionThe value of the integral ∫10x(1−x)ndx, is
View SolutionThe value of the integral ∫10x(1−x)ndx is
View SolutionThe value of the integral ∫20∣∣x2−1∣∣dx is
View SolutionThe value of the integral ∫101(1+x2)32dx is
View SolutionThe value of the integral ∫10x31+x8dx is
View SolutionThe value of the integral l=∫10x(1−x)ndx is
View SolutionThe value of the integral ∫10sin−1(3x−4x3)dx is
View Solution