Three events A , B and C have probabilities 25,13and12 respecively. Given tat P(A∩C)=15andP(B∩C)=14, find the values of P(C/B)andP(A−∩C−).
To solve the problem step by step, we will find the values of P(C|B) and P(A′∩C′).
Step 1: Find P(C|B)
We know that:
P(C|B)=P(C∩B)P(B)
From the problem, we have:
- P(B)=13
- P(B∩C)=14
Substituting these values into the formula:
P(C|B)=P(B∩C)P(B)=1413=14×31=34
Step 2: Find P(A′∩C′)
We will use the formula:
P(A′∩C′)=1−P(A∪C)
To find P(A∪C), we use the formula:
P(A∪C)=P(A)+P(C)−P(A∩C)
From the problem, we have:
- P(A)=25
- P(C)=12
- P(A∩C)=15
Substituting these values into the formula:
P(A∪C)=25+12−15
To add these fractions, we need a common denominator. The least common multiple of 5 and 2 is 10. So we convert each fraction:
- 25=410
- 12=510
- 15=210
Now substituting:
P(A∪C)=410+510−210=4+5−210=710
Now we can find P(A′∩C′):
P(A′∩C′)=1−P(A∪C)=1−710=310
Final Answers
1. P(C|B)=34
2. P(A′∩C′)=310
P(C|B)=P(C∩B)P(B)
- P(B)=13
- P(B∩C)=14
P(C|B)=P(B∩C)P(B)=1413=14×31=34
P(A′∩C′)=1−P(A∪C)
P(A∪C)=P(A)+P(C)−P(A∩C)
- P(A)=25
- P(C)=12
- P(A∩C)=15
P(A∪C)=25+12−15
- 25=410
- 12=510
- 15=210
P(A∪C)=410+510−210=4+5−210=710
P(A′∩C′)=1−P(A∪C)=1−710=310
1. P(C|B)=34
2. P(A′∩C′)=310
Topper's Solved these Questions
Similar Questions
Three events A, B and C have probalities 25,13and12, respectively. If, P(A∩C)=15andP(B∩C)=14, then find the values of P(C/B) and P(A'∩C')
View SolutionIf P(A∪B)=3/4andP(A)=2/3 , then find the value of P(A∩B).
View SolutionIf P (A)=38, P(B)=12and P(A∩B)=14, f∈d P(A/B)and P(B/A).
View SolutionIf A and B are two independent events such that P(¯¯¯A∩B)=215 and P(A∩¯¯¯B)=16 , then write the values of P(A)and P(B)
View SolutionA and B are two mutually exclusive events of an experiment. If P(A)=0.35,P(A∪B)=0.65andP(B)=p, find the value of p.
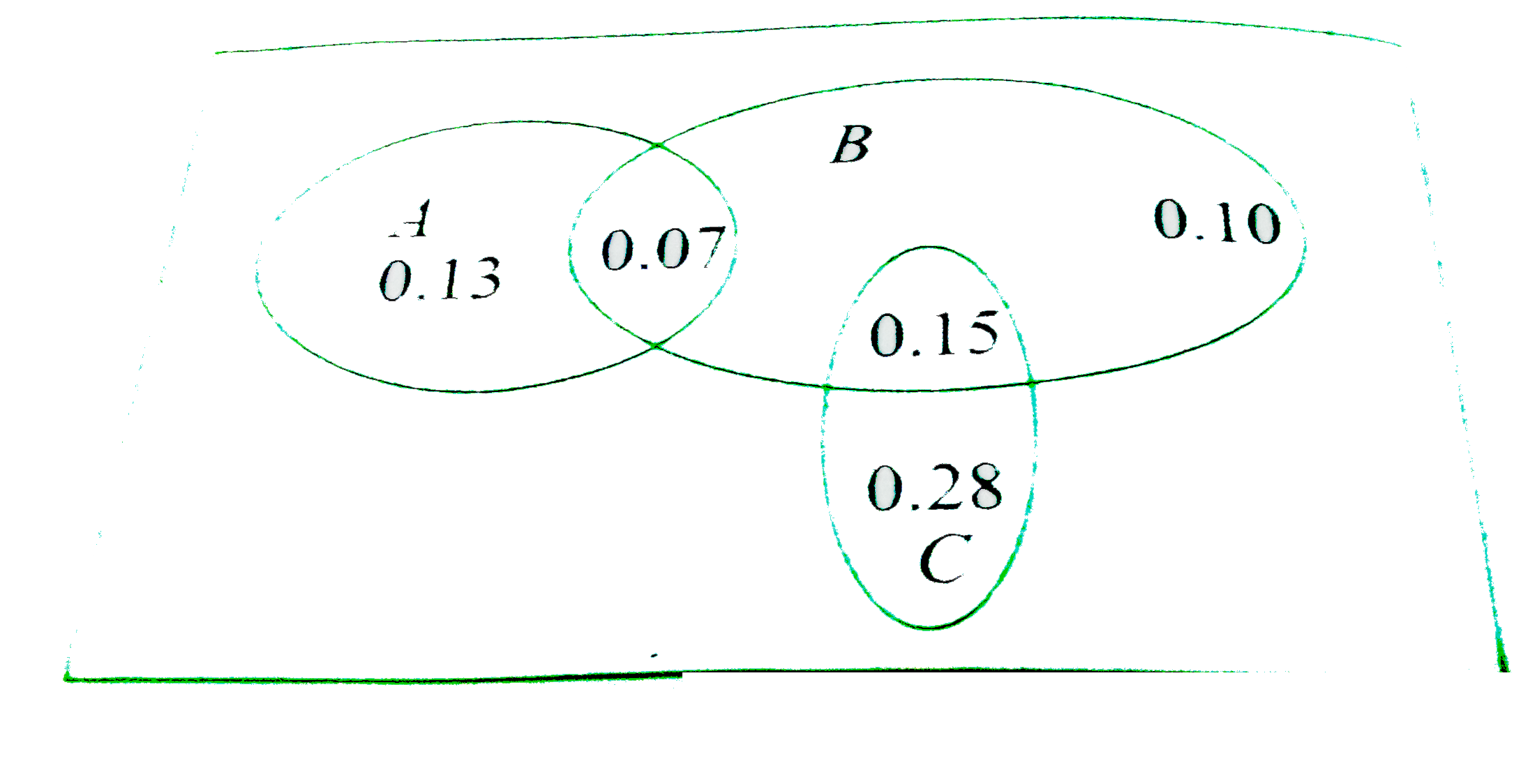
View SolutionThe following Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections.
Determine
(a) P(A)
(b) P(B∩¯¯¯C)
(c ) P(A∪B)
(d) P(A∩¯¯¯B)
(e) P(B∩C)
(f) Probability of the event that exactly one of A, B, and C occurs.
View SolutionIf P(notA)=0.7,P(B)=0.7andP(B/A)=0.5 , then find P(A/B)andP(A∪B).
View SolutionThe probabilities of three events A,B,andC are P(A)=0.6,P(B)=0.4,andP(C)If P(A∩B)=0.8,P(A∩C)=0.3,P(A∩B∩C)=0.2P(A∪B∪C)>0.85, then find the range of P(B∩C).
View SolutionIf two events AandB are such that P(Ac)=0.3,P(B)=0.4,andP(A∩Bc)=0.5 , then find the value of P[B/(A∪Bc)].
View SolutionLet AandB are events of an experiment and P(A)=1/4,P(A∪B)=1/2, then value of P(B/Ac) is 2/3 b. 1/3 c. 5/6 d. 1/2
View Solution
