Using analytical geometry, prove that the diagonals of a rhombus are perpendicular to each other.
we need to prove ABCD is a rhombus
where AB=BC=BD=DA
A(0,0) B(S,0)
C(A+S,B) D(A,B)
AD=√a2+b2=s
a2+b2=s2
AB=√s2+02=s
BC=√(a+s−s)2+(b−0)2=√a2+b2=S
CD=√(a+S−a)2+(b−b)2=√s2=S
DA=√a2+b2=√s2=S
secondly we need to prove they are perpendicular
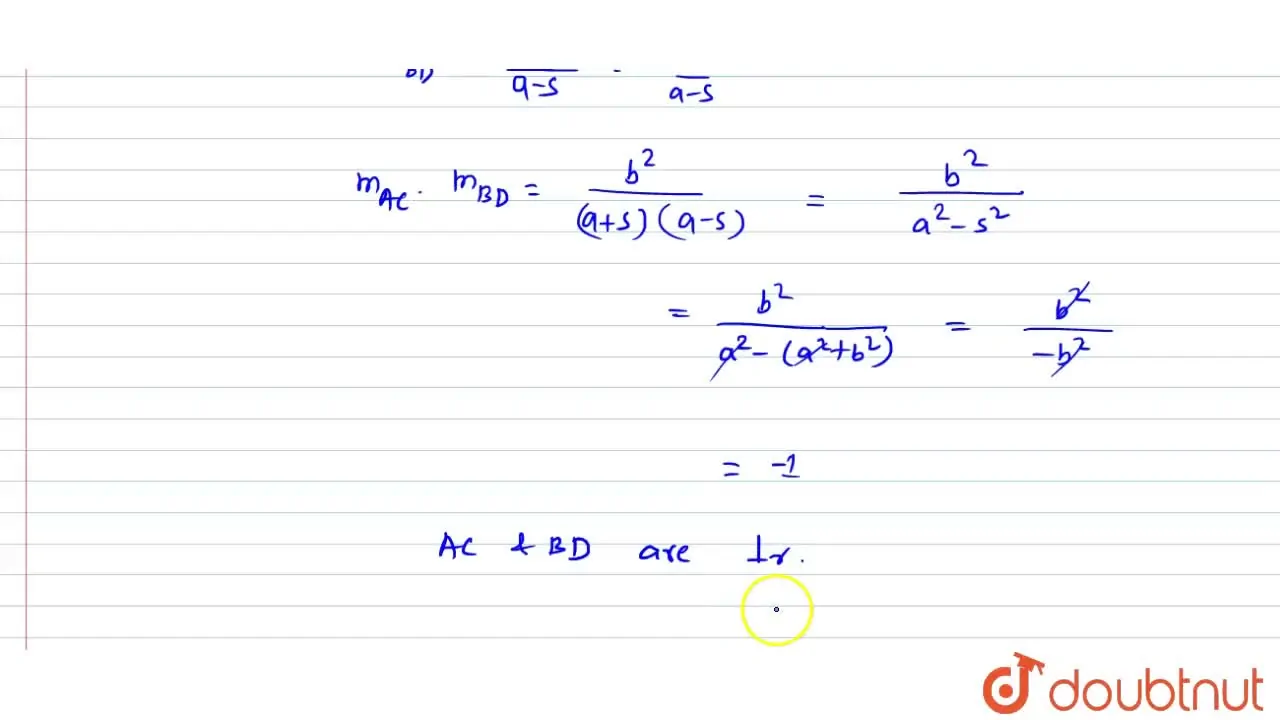
MAC=b−0a+s−0=ba+s
MBD=b−0a−s=ba−s
MAC⋅MBD
ba+s⋅ba−s
b2a2−s2
b2−b2
-1
multipliplication of there slope is-1 so they are perpenticular
Related Playlists
Similar Questions
Show that the diagonals of a rhombus are perpendicular to each other
View SolutionDiagonals of a rectangle are perpendicular to each other.
View SolutionThe diagonals of a square are equal and perpendicular to each other.
View SolutionProve that If the diagonals of a parallelogram are perpendicular, then it is a rhombus.
View SolutionShow that the diagonals of a rhombus bisect each other at right angles.
View SolutionThe diagonals of a rhombus are unequal and bisect each other at right angle
View SolutionState whether the statements are true (T) or (F) false.
Diagonals of a rhombus are equal and perpendicular to each other.View SolutionIf the diagonals of a parallelogram are perpendicular, then it is a rhombus.
View SolutionThe diagonals of a rhombus are perpendicular. The contrapositive of the above statement is
View SolutionName the quadrilaterals whose diagonals
are perpendicular bisectors of each other.View Solution